Rocket to launch 8 cubesat to LEO at an equidistant distance

Question

I am working on a project, which consists of the launch of 8 cubesat 1U to LEO (Low Earth Orbit) and I started working on the launch part, the idea of ​​the project is to be as cheap as possible, the problem is that cubesats have to be separated, at the same distance (or approximate) from each other to create a "full-time" communication network for probes and satellites to saturn and jupiter moons. and I'm looking for a rocket capable of launching these 8 cubesats in a zolo launch, the total load will be 0.8 kg per cubesat so there will be a total weight of approximately 6.5kg. The distancing could make it the last stage of the rocket or it could add a small stage of cold gas to each cubesat to make the distancing themselves, but that would add more weight to the payload. What rocket do you recommend? Which method is cheaper, the distance by cold gas or using the last stage and go dropping cubesats?

Answer 1

You'll be definitely better off with thrusters on individual cubesats, when taking the total mass: last stage + payload, into consideration. To create the distance needed, with a single launch, you'll need to put the satellites into an elliptical orbit tangent to the target one, but of period longer or shorter by 1/8 or an integer fraction multiple of 1/8.

In this sort of problems orbital period is the defining characteristic of the orbits needed. Unlike in most other orbital mechanics problems, semi-major axis, eccentricity, velocity at the apses, all that stuff is to be derived from orbital period, and it's the period of the orbit - time to make "full circle" - that's the one control variable ruling all the others.

For simplicity of the example, let's make the the multiplier be 1, and so the period of the orbit where the cubesats are deployed would be 9/8 of desired (using 7/8 instead would be cheaper but if it's LEO, that would likely result in reentry trajectory.) So, the spacecraft deploys the cubesats, then fires retrograde to burn up and not be space junk, or goes elsewhere to drop main payload. The first cubesat immediately slows down until it enters the target orbit, while the rest continue on the elliptic orbit. After completing 1 orbit, the next satellite fires its thruster - it's 1/8 of the orbit behind the first one (it took it 1/8 longer to return to the same spot). The rest continue. Next orbit, another fires to park 1/8 of the orbit after the second, and 1/4 after the first. And so on.

Now if you use cubesats without propulsion, instead of them putting themselves into the target orbit, you have the propulsion stage slow down, deploy cubesat, accelerate back to the 9/8 orbit (with all remaining cubesats on board), an orbit later slow down to deploy next one into target orbit, accelerate again, over and over - and slowing down or accelerating all the payload it didn't deploy yet. I hope you see how this gets awfully expensive in terms of fuel and launch mass.

In practice you'd probably go with a smaller multiplier - 1/8 of a LEO period is an awful lot of delta-V and probably too much for puny gas thrusters, but instead you can enter an orbit that is, say, 65/64th of the target period, each orbit would distance the deployed swarm from the last cubesat already in target orbit by 1/64th of the orbit, so after 8 orbits next cubesat fires its engine and starts following the previous one, 1/8 orbit behind.

I'm sorry but I won't help with picking the right spacecraft.

Answer 2

I was looking for a constellations of smallsats (they are not cubesats) that were able to space themselves out using only drag. I hypothesized it was TROPICS but that hasn't been launched yet. But I finally found it, and it turns out to be the Cyclone Global Navigation Satellite System or CYGNSS and is discussed in How can the CYGNSS spacecrafts (actually) measure ocean roughness?.

The technique is called differential drag. Briefly, you use the attitude control of a small satellite to increase or decrease its drag relative to the others. All satellites in a given low Earth orbit will experience drag and their orbits will constantly drop and their periods shorten, but if one drops a little bit faster it will "speed up" relative to the others and move forward in phase relative to them.

While at first it seems like "free" or cost-less maneuverability, it comes at the cost of all the satellites eventually deorbiting. However if the lifetime of the mission is only a few years than it's no problem.

See for example @Terrance Yee's answer to What goes into the planning and execution of the deployment of groups of LEO satellites?

Wikipedia explains that it uses differential drag to move spacecraft around at least within a given orbital plane.

Inter-satellite spacing is controlled by adjusting spacecraft orientation and, as a result, the difference in atmospheric drag between satellites. This technique is referred to as differential drag. An increase in drag lowers a satellite's altitude and increases its orbital velocity.²⁶ The distance between spacecraft changes as a result of their relative velocities. This is an alternate way of managing the spacing between a constellation of satellites, as opposed to using traditional active propulsion, and is significantly lower cost. It allows for more satellites to be built for the same net cost, resulting in more frequent sampling of short lived, extreme weather events like tropical cyclones.¹⁶ Differential drag maneuvers were conducted throughout the first year and a half of on-orbit operations, and have resulted in a well-dispersed constellation that is able to make measurements with the desired sampling properties.²⁷,²⁸

¹⁶Ruf, Christopher S. et al. (2015) New Ocean Winds Satellite Mission to Probe Hurricanes and Tropical Convection

²⁶Finley, T.; Rose, D. (2014). Astrodynamics 2013: Proceedings of the AAS/AIAA Astrodynamics Specialist Conference held 11-15 August 2013, Hilton Head, South Carolina, U.S.A. 150. American Institute of Aeronautics and Astronautics. pp. 1397–1411.

²⁷Ruf, Christopher et. al (2018) A New Paradigm in Earth Environmental Monitoring with the CYGNSS Small Satellite Constellation.

²⁸Bussy-Virat, C. D. et al. (2018) Assessment of the Differential Drag Maneuver Operations on the CYGNSS Constellation (paywalled). IEEE Journal of Selected Topics in Applied Earth Observations and Remote Sensing. 12: 7–15.

See also Differential Drag Control Scheme for Large Constellation of Planet Satellites and On-Orbit Results in arXiv. It's from the 9th International Workshop on Satellite Constellations and Formation Flying, Boulder, CO, 19-21 June 2017 and refers to Planet Labs' constellations of Dove satellites. Here's the abstract:

A methodology is presented for the differential drag control of a large fleet of propulsion-less satellites deployed in the same orbit. The controller places satellites into a constellation with specified angular offsets and zero-relative speed. Time optimal phasing is achieved by first determining an appropriate relative placement, i.e. the order of the satellites. A second optimization problem is then solved as a large coupled system to find the drag command profile required for each satellite. The control authority is the available ratio of low-drag to high-drag ballistic coefficients of the satellites when operating in their background mode. The controller is able to successfully phase constellations with up to 100 satellites in simulations. On-orbit performance of the controller is demonstrated by phasing the Planet Flock 2p constellation of twelve cubesats launched in June 2016 into a 510 km sun-synchronous orbit.

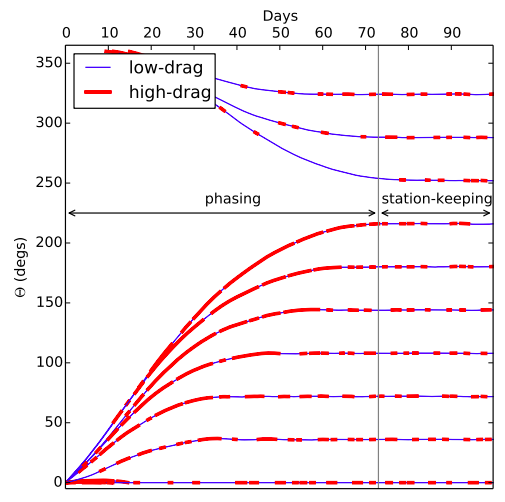
Here is an example of a simulation of the use of alternating between high and low drag configurations to achieve equal spaced phasing and then to maintain it using small station-keeping drag adjustments:

Figure 8: Time-discretized high-drag commands are assigned to achieve desired slots (b) with commands

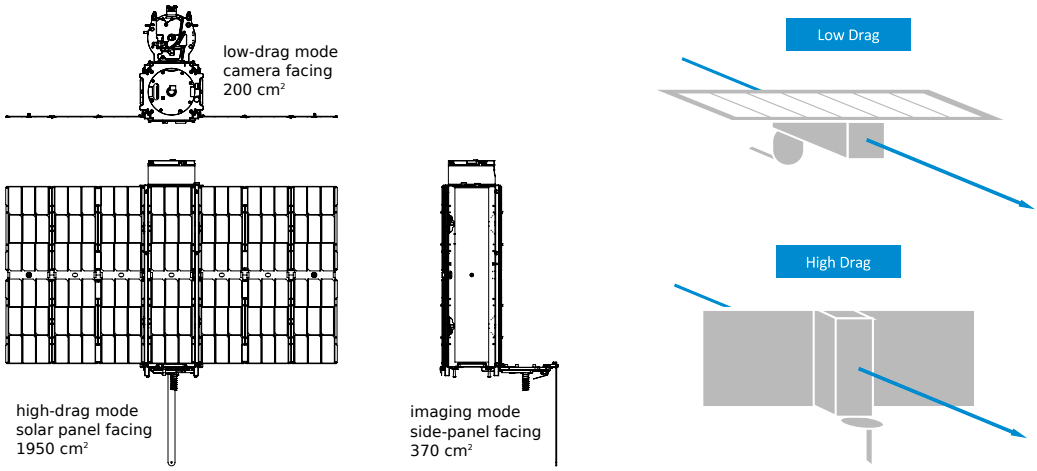
Figure 9: Attitude modes of Dove satellite enable large drag area ratios (a) Orthographic projections with cross-sectional areas. (b) High-drag and low-drag attitudes