You must use all six the orbital parameters. @RussellBorogove points out one, but $e, a, i, \Omega, \omega, L$ are all needed to obtain the position of an object at a single point in time in three dimensions.
There are several other answers here in SXSE that explain how to get the position in 3D from these parameters. I'll add links to one or two hereCheck the following; 1, 2, 3. 4, 5 and 6, listed in a few hoursno particular order.
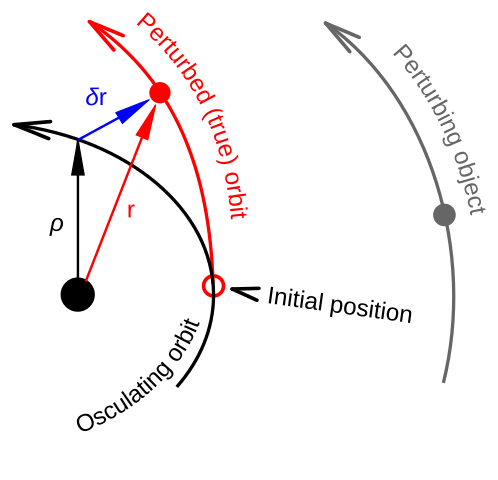
As an asside, Keplerian osculating orbits are handy, but the reality of gravity is that in a complex system like this — Earth having such a big moon, the Sun not fixed because of Jupiter and the other giant planets — they are just approximations. It's why the numbers change daily, and it's why if you use these osculating elements to predict a position at any other time, even for example half way between one day and the next in your example, you'll get a small error.
below: Vector diagram of Encke's Method of perturbation analysis, showing the osculating orbit, the perturbed orbit, and a perturbing body. From here.