Update 2
The taper ratio formula seems to be correct. The crosscheck stress calculation error mentioned in the Update 1 below is found to be due to:
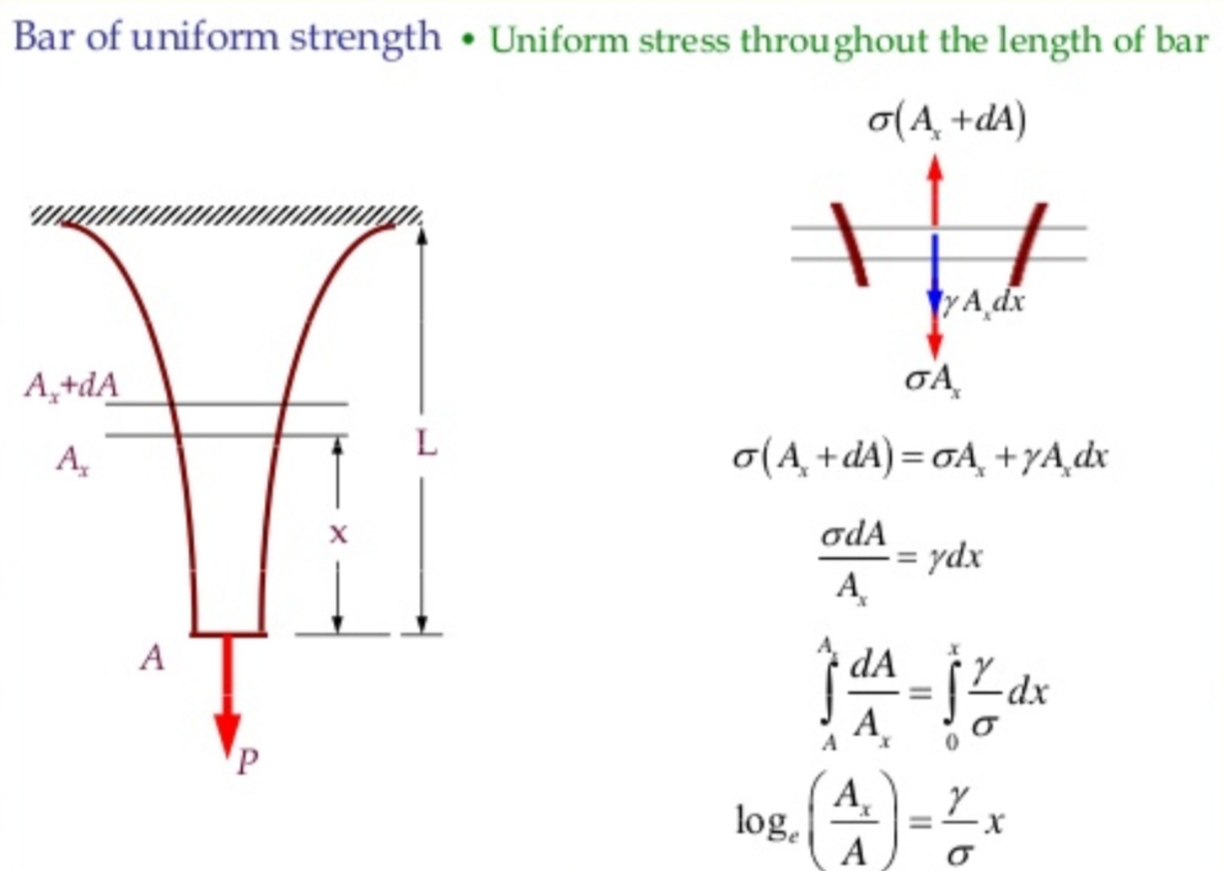
a) incorrectly calculated tether volume (and therefore mass). I assumed a linear change of cross section area across the tether length. In fact, to achieve the uniform strength, a logarithmic increase in erea is required.
b) not accounting for varying gravity across the altitude (from 8.87001 m/s2 at the surface to 8.72529 m/s2 at 50 km altitude).
The tether mass values are now corrected in the text of the Update 1 below.
Update 1: Apologies for making already long answer even longer, but I forgot to mention few other very important factors in the above list:
Now that we've accounted for hot venusian climate (tensile stress reduction), let's see what our tether is going to look like in order to have a similar safety coefficient as originally planned (**total SF **willtotal SF will be 3.3 for the following example):
SF for tension = 5: operating tensile stress 2.1GPa/5=0.42GPa.
Taper ratio formula gives us the ratio of 16.9
(by the way, it looks like the OP has simplified the formula from the referenced answer and, when I use the formula from the body of the current question, the taper ratio is not very accurate, which is highlighted by the fact that I
always receive a higher stress when doing the cross check: (mass of probe + mass of tether)×gravity/(larger diameter of the tether))(by the way, it looks like the OP has simplified the formula from the referenced answer and, when I use the formula from the body of the current question, the taper ratio is not very accurate, which is highlighted by the fact that I
always receive a higher stress when doing the cross check: (mass of probe + mass of tether)×gravity/(larger diameter of the tether))
The error has been attributed to incorrectly calculating tether volume (therefore mass) and not accounting for varying gravity across the height. The tether mass values in the examples below are now updated to the corrected ones.
The tether mass now grows from 270kg to quite disappointing 2,093 21,093kg604 kg.
- Total SF 4.5 (6.5 for tension and 15 for bending)
Taper ratio 39.5;
Areas: 2.75 to 108.4 sq.mm;
Diameters: 1.87 to 11.75 mm;
Bend radius: 3.5 m (I list the figure for single wire purely for comarison, remember it will be smaller for rope);
Tether mass: 5
5,7783,778884 kg. - Total SF 5.2 (8 for tension and 15 for bending)
Taper ratio 92.2
Areas: 3.38 to 311.6 sq.mm;
Diameters: 2.07 to 19.92 mm;
Bend radius: 6.05 m (again, only for comparison, rope will accomodate smaller);
Tether mass: astonishing
15,640159,640199 kg.