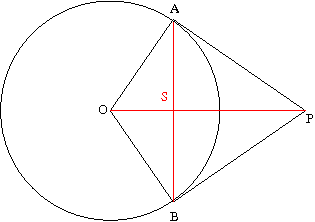
I would need to compute the solid angle of the Earth from a spacecraft when close to the Earth, where no small angles approximation can be made. I thought it wouldn't be that hard but I feel actually quite stuck on that problem...
If anyone has a hint that'd be great!
oz380