For standard orbits we can use Keplerian elements, TLE, or other similar. These don't make much sense for Halo orbits, which are not around a central body, but around a Lagrangian point, and follow an entirely different set of rules. What description is used to parametrize these?
For example: how would the description of Queqiao orbit look like, and how could I go about reading it, to see e.g. if it's eclipsed by Moon some of the time, or remains in view of Earth at all times?
tl;dr: For a given pair of bodies in circular orbits around their center of mass, there are two symmetric families ("Northern" and "Southern") of proper halo orbits associated with each of the Lagrangian points L1, L2, and L3. We usually only talk about those with L1 and L2 because L3 is so far away from the secondary body (Earth in the case of Sun-Earth Lagrangian points, the Moon in the case of Earth-Moon). So you need three parameters; two enumerations and one floating-point value. 1) North or South, 2) L1, L2, or L3-associated, and 3) some floating point number that represents the position that the orbit lies in between the two extreme ends of the family where it either terminates or bifurcates. So far I don't know if that has a generally accepted parameterization that always works, or not. I am not sure if something simple like "energy" ($C_3$) or some amplitude or distance would work without ambiguities in some cases.
As a practical answer, you could describe a periodic halo orbit with an in-plane amplitude $A_Y$ and out-of-plane amplitude $A_Z$ to someone, and then they could try to calculate the orbit and find the X, Y, and Z positions as a function of time to get the motion in space, and then determine when the orbit would be blocked from points on the Earth by the Moon. I discuss this further in this answer to Are large halo orbits around L₁'s and L₂'s preferred over small orbits for reasons other than geometry?, but see the pics below from Robert W. Farquhar's hundred page tome The Utilization of Halo Orbits in Advanced Lunar Operations, NASA Tech. Note D-6365.
But remember: this is for *circular orbits of 2 bodies only, and the real Moon's movement (and other effects) is more complex.
In section II.B.2.b, he points out:
For every value of $A_y$ > 32,871 km, there is a corresponding value of $A_z$ that will produce a nominal path where the fundamental periods of the y-axis and z-axis oscillations are equal. In this case, the nominal path as seen from the earth will never pass behind the moon. The exact relationship between $A_y$, and $A_z$, for this family of nominal paths is given in Figure 5.
The extremely cool and colorful paper E. J. Doedel et al, (2007) Elemental periodic orbits associated with the libration points in the circular restricted 3-body problem International Journal of Bifurcation and Chaos 17, 2625 (2007). https://doi.org/10.1142/S0218127407018671 builds a system of illustrations that show all of the known, periodic, orbits in the CR3BP (Circular Restricted Three-Body Problem). This includes many kinds or classes of orbits as shown in the table, but excludes Lissajous Orbits because they are not in general periodic. (note: ignore the drawing in the Wikipedia article!)
You can also download the paper from its non-paywalled ResearchGate site, make some coffee, then spend six months enjoying it.
There is also an un-paywalled copy of their earlier paper available: The Computation of Periodic Solutions of the 3-Body Problem Using the Numerical Continuation Software AUTO D. J. Dichmann, E. J. Doedel, and R. C. Paffenroth Int. Conf. on Libration Point Orbits and Applications, Aiguablava, Spain, 10-14 June, 2002
I have made three montages of Figure 3 with Figures 13 (L1), 14 (L2) and 15 (L3) and shown them below. For each, only the Northern Halo orbit is shown, the Southern would be symmetrically reflected below the plane. These drawings use the Earth-Moon system for simple visualization, and Figure 3 is for the mass ratio of the Moon to the Earth ($\mu \approx 0.01215$).
You can also see how to generate and plot a few Halo orbits with Python using the script in the question How to best think of the State Transition Matrix, and how to use it to find periodic Halo orbits? which comes from the classic paper written by Kathleen Connor Howell Three-Dimensional, Periodic 'Halo' Orbits Celestial Mechanics 32 (1984) 53-71.
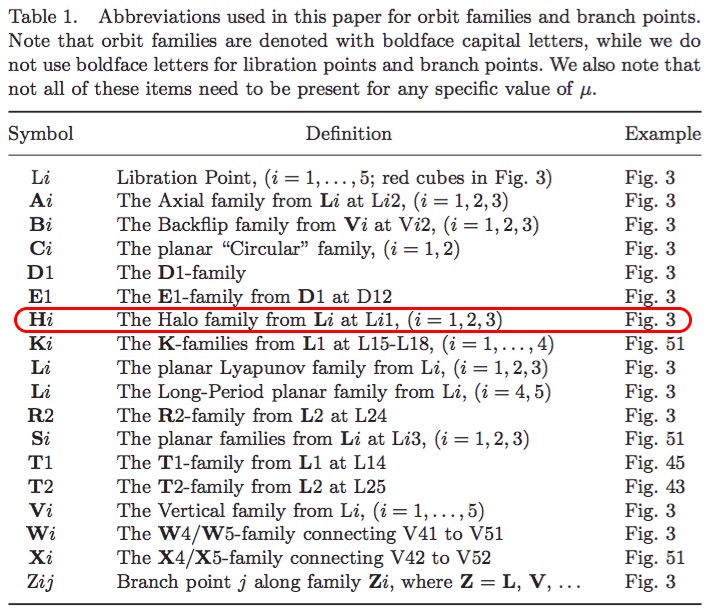
Caption for Figure 3: (the lower part with all the elbows):
Fig. 3. Bifurcation diagram for the Earth–Moon system (μ = 0.01215), showing families of periodic orbits that emanate from the libration points and from subsequent branch points. The red cubes are the libration points. Small white spheres denote branch points, and small dark-red spheres denote collision orbits. The planar families C1, C2, and D1, are only partially represented; in particular, the fact that D1 arises from C1 via a period-doubling bifurcation is not indicated in the diagram. A glossary of the notation used is given in Table 1.
