If a spacecraft was in an EM-L2 halo/lissajous orbit, and another craft would was going to approach EM-L2 a few days later, could they rendezvous immediately or is there a limitation when the second craft could rendezvous with the first? Is such a rendezvous even possible?
$\begingroup$
$\endgroup$
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
note: The following two questions are related, may possibly have some helpful information, but both remain unanswered:
- Did DSCOVR travel “along the stable manifold of its future SE L1 Halo orbit” to get there?
- Can Lissajous orbits have stable/unstable manifolds?
If a spacecraft was in an EM-L2 halo/lissajous orbit, and another craft would was going to approach EM-L2 a few days later, could they rendezvous immediately or is there a limitation when the second craft could rendezvous with the first?
tl;dr: They could rendezvous immediately or at any time later.
Theoretically you can approach a halo orbit at any point by following a trajectory that falls within it's associated Lagrange point's stable manifold. But if your target craft were in a near-rectilinear halo orbit for example these are extreme and have certain parts that travel close to the Moon at higher relative velocities, you may want to avoid approaching at those points.
For more on near-rectilinear halo orbits see answers to:
- What is a near rectilinear halo orbit?
- Why is a near rectilinear halo orbit proposed for LOP-G (formerly known as Deep Space Gateway?)
Orbits about equilibrium points, including halo orbits have what are called stable and unstable manifolds. The manifolds are surfaces made up of an infinite number of trajectories laying side-by-side that all spiral in towards (stable) or away from (unstable) one halo orbit.
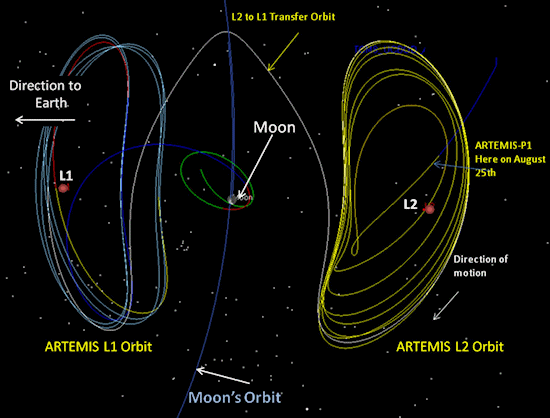
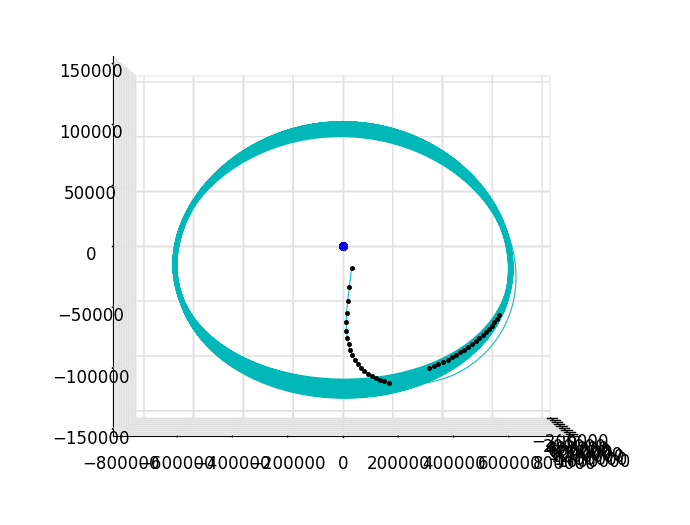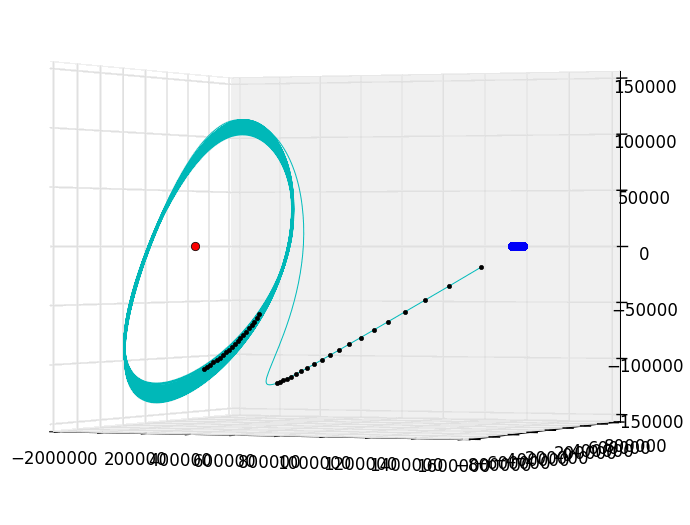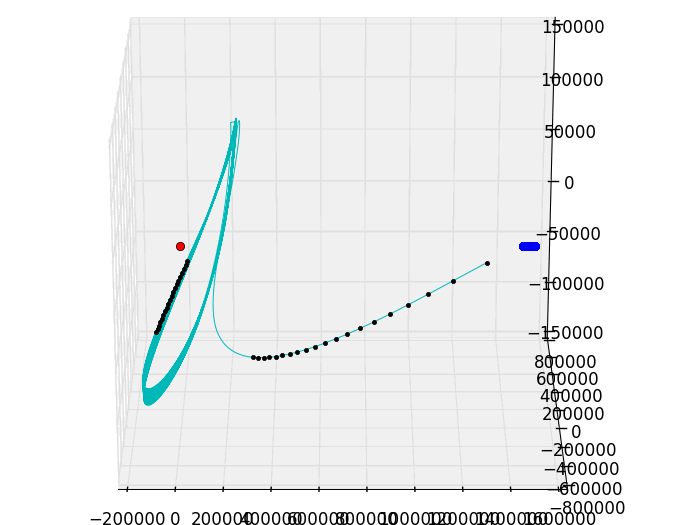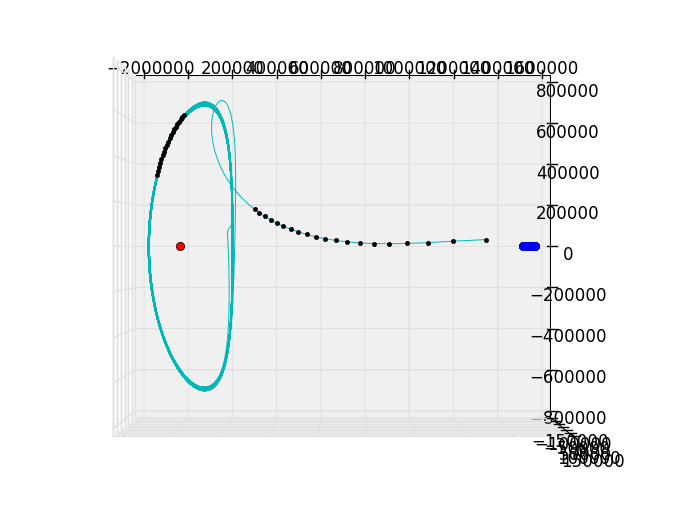
Here is the Artemis spacecraft exiting a halo orbit about L2 by spiraling away from its halo orbit towards the Moon along one trajectory in L2's unstable manifold, passing the Moon, then traveling up on one trajectories in L1's stable manifold:
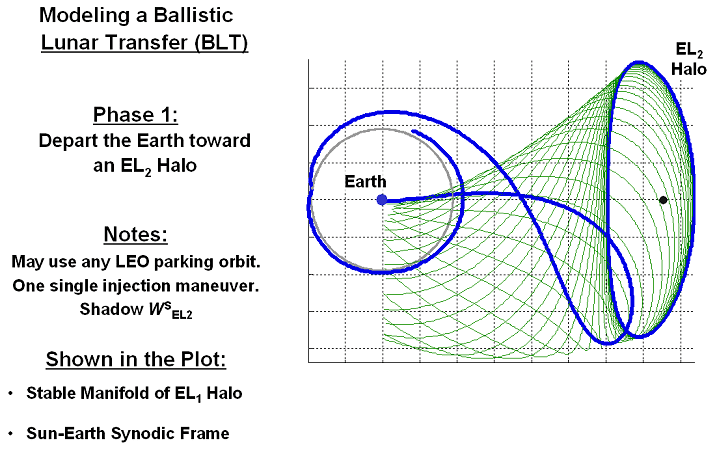
Here is what the manifolds look like when you represent them by a closely spaced set of trajectories within it:
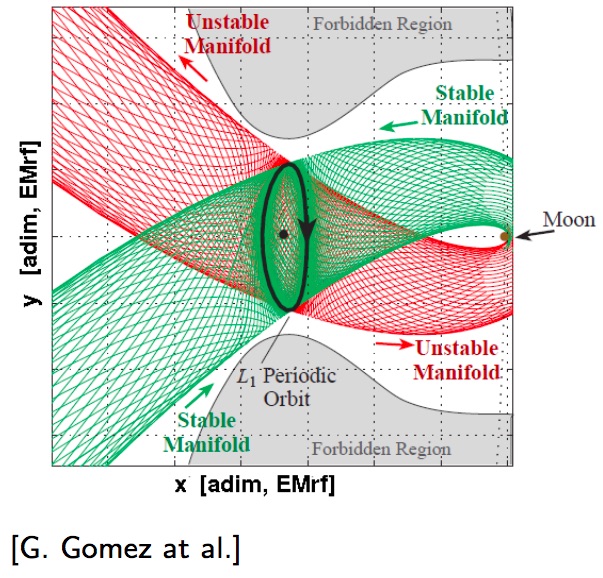
and here is what it looks like if you represent both manifolds coming from (or going towards) both directions:
Here is the trajectory of the SOHO spacecraft from Earth to it's halo orbit presumably along a stable manifold. The way it nicely spirals right up to the halo orbit in the right combination of velocity and position shows that it hopped on to the manifold at some point near LEO. The diagram is from this question:
Here's a maddeningly slow video of an approach along a stable manifold (I recommend speeding it up to maximum in YouTube):