This answer to Does NASA really report the power of rockets in horsepower? cites NASA news item Space Launch System Booster Aimed and Ready to Fire which says
"What's impressive about this test is when ignited, the booster will be operating at about 3.6 million pounds of thrust, or 22 million horsepower," said Alex Priskos, manager of the SLS Boosters Office at Marshall. "This test firing is critical to enable validation of our design."
If I said my car was operating at 400 Nm or 200 kW you'd balk because of the "or" which suggests that these are two ways to say the same thing. You can use "and" because you'd be measuring two different pieces of information. They may be related but you cant' simply get one from the other.
And yet here is 3.6 million pounds of thrust, or 22 million horsepower.
Questions:
- While it wouldn't make physical sense, how can we arrive at something like 6 hp/lbf for the SLS booster?
- Is this very roughly an invariant? Would all large boosters be within a factor of two of this number, or at least boosters of a similar propellant type?
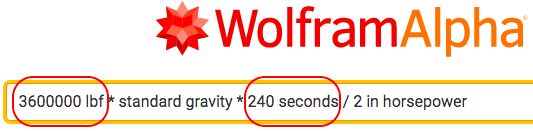
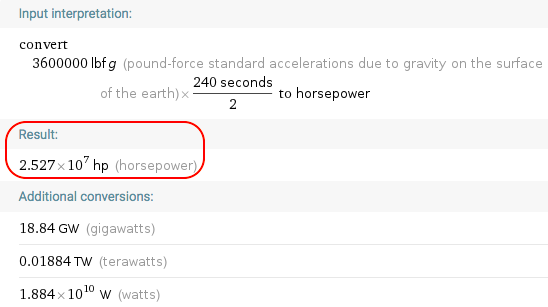
update: @OrganicMarble has pointed to @MarkAdler's answer which explains a definition and provides a link to a calculator in Wolfram Alpha. When I enter 3.6 million pounds of thrust I get back 48 million hp, which is over a factor of two higher than the 22 million horsepower mentioned above.
Somebody is wrong, or there are multiple definitions, or maybe I didn't use the calculator properly...?