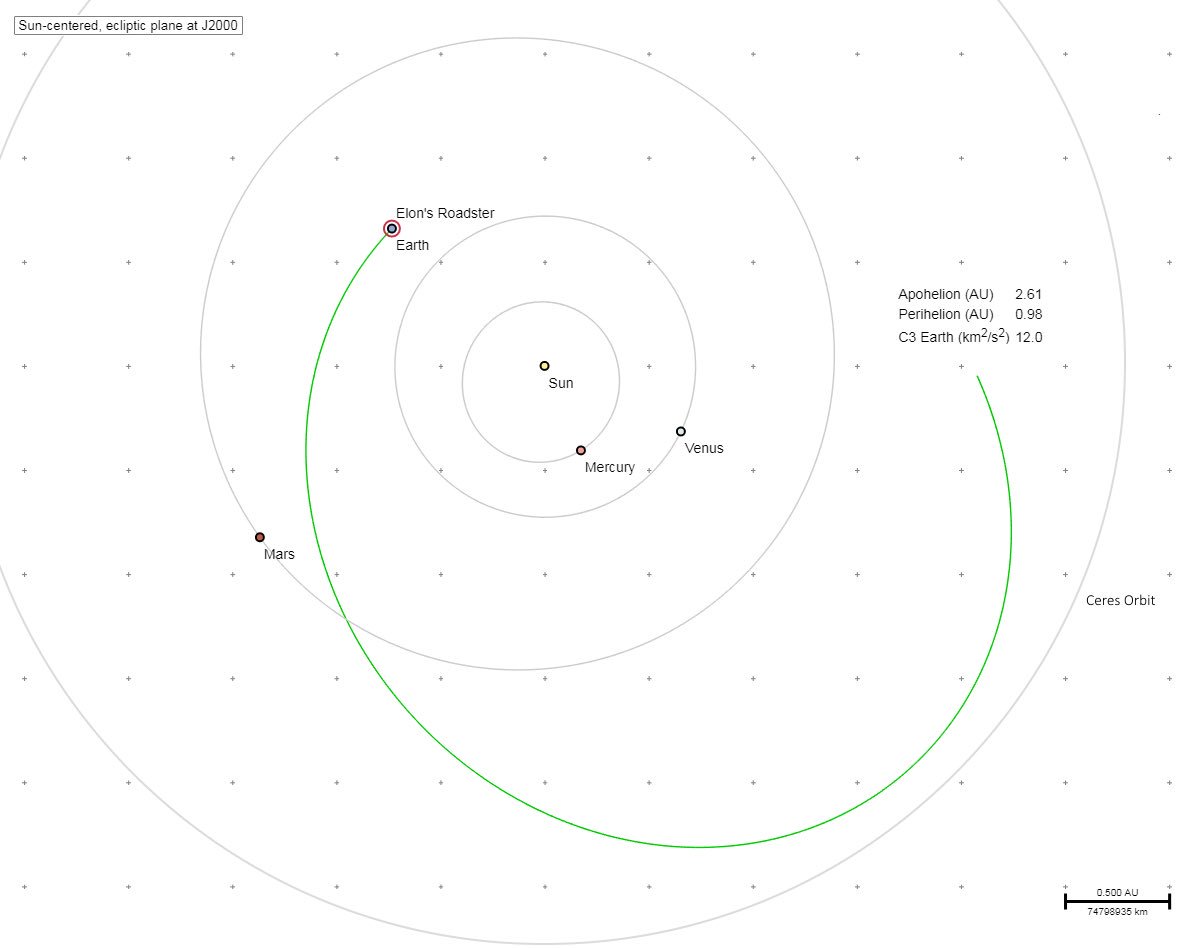
In this answer to How close would the Tesla Roadster with Starman have to get to Earth in order to become attracted and fall on Earth? I just wrote:
When Roadster was launched from Earth Elon Musk mis-tweeted that it had achieved 12 km^2/sec^2 excess C3 ("energy") relative to Earth after launch. He got the number wrong but the point is that once Roadster had this positive energy relative to Earth it could never "fall back" to Earth.
See Starman/Roadster in a=1.795 AU orbit, now what's the method to this madness? and this answer.
Question: How and/or why did Elon Musk mistweet Roadster's C3 so badly? He predicted a heliocentric apoapsis of 2.61 AU; way past Mars and almost to Ceres. Where is Roadster's close approaches and milestones says
Far point from Sun on May 20, 2020 at a distance of 1.664 AU.
Is there any somewhat reliably sourced-information about how or why this mistweeted announcement was off by so incredibly much? Surely they would have known roughly how much propellant remained while in the 6 hour high parking orbit around Earth, it's hard to imagine believing a number that was off by 1 AU.
Perhaps it had enough to go to 2.61 but its engine was pointed in the wrong direction because it lost attitude control?
WARNING:
This plot in Musk's tweet is now known to be incorrect! See this answer for further clarification.