Most of the literature discusses the pitch angle and constraints on it, namely, trying to keep it zero for load relief. This leads to the gravity turn. I understand that ideal trajectories are within the pitch-plane alone. However, I would expect certain constraints to be present for yaw angle as well - perhaps to change inclination, or to reduce atmospheric load. But no discussion was found in this regard. Would there be any such constraints?
5
-
$\begingroup$ Some (many?) rockets will "roll to pitch" so they don't have to yaw. If the rocket is sufficiently radially symmetric (longitudinally) then yaw angle is no different than pitch angle (aerodynamically) $\endgroup$– BrendanLuke15Commented Mar 15, 2022 at 13:56
-
1$\begingroup$ @OrganicMarble I've just found my new favourite word: squatcheloid $\endgroup$– BrendanLuke15Commented Mar 15, 2022 at 13:58
-
1$\begingroup$ @BrendanLuke15 yeah, that is a weird word. $\endgroup$– Organic MarbleCommented Mar 15, 2022 at 13:59
-
1$\begingroup$ Shuttle had limits on the product of dynamic pressure and angle of attack (Qalpha) and the product of dynamic pressure and sideslip angle (Qbeta). Example: i.sstatic.net/6FbzF.jpg $\endgroup$– Organic MarbleCommented Mar 15, 2022 at 13:59
-
2$\begingroup$ OK folks... my challenge? Use squatcheloid in casual 'cocktail party' conversation without forcing it. If you can... please introduce me to your circle of friends! $\endgroup$– BradVCommented Mar 16, 2022 at 0:29
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
1
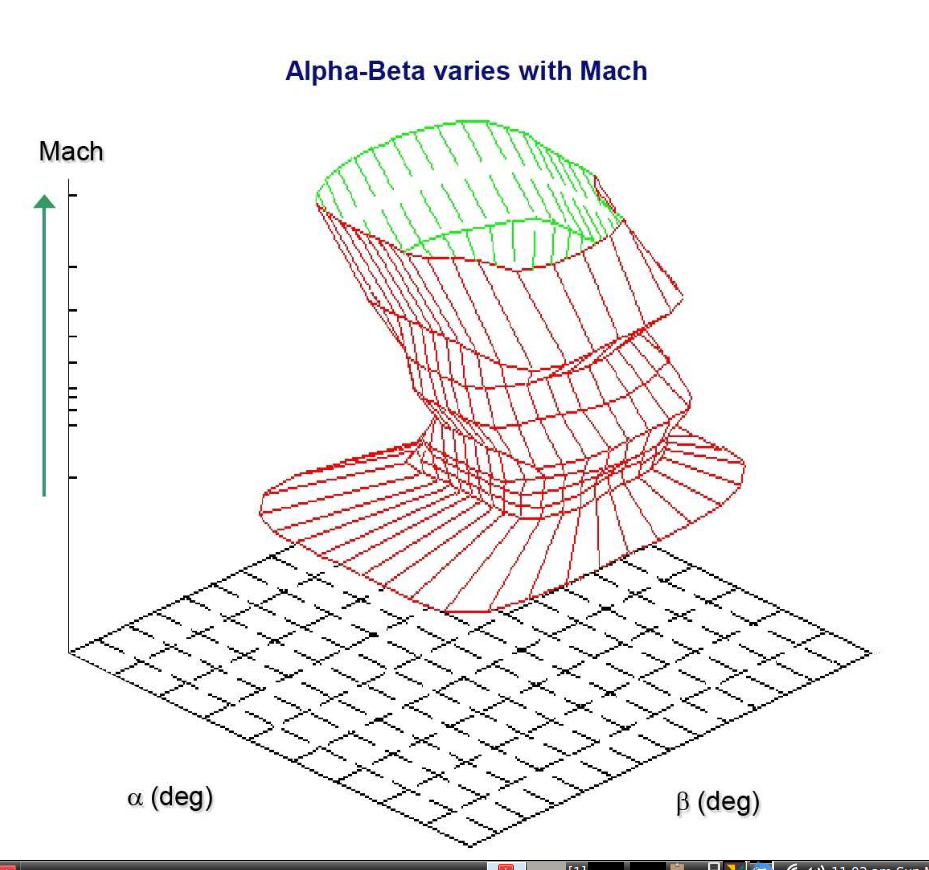
I am familiar with some legacy launch vehicles that had some such similar constraints. However, these contraints are usually stated in the form of the product of the dynamic pressure and the aerodynamic angles alpha (angle of attack) and beta (angle of sideslip).
- Shuttle had such Q-alpha and Q-beta constraints defined as a series of "Q-planes" or "squatcheloids" indexed by Mach number. Visualize an oddly shaped funnel the vehicle had to fly through.
- I don't know details, but Atlas boosters had similar constraints. These vehicles used a system called ADDJUST to calculate the first stage trajectory. My impression is that ADDJUST was fundamentally similar to the shuttle system DOLILU given the differences in the vehicles.
ADDJUST also verified that all equally critical trajectory criteria were met to ensure mission success
• Structural loads
• Control capability
• Nose fairing temperatures
• Angles of attack, pitch and yaw
• Integrated heating
• Product of dynamic pressure and angle of attack (Q-Alpha) and sideslip (Q-Beta) at jettison events
• Angular rates and accelerations, pitch and yaw
• Performance to Centaur target orbit
• Range safety boundaries
(emphasis mine)
References:
answered Mar 15, 2022 at 14:55
-
$\begingroup$ Thanks for your answer! While I intended the question to be more towards symmetric launch vehicles, your answer did provide some avenues to explore. $\endgroup$ Commented Apr 5, 2022 at 7:14