Are there any studies on Mars-Earth ballistic cyclers taking Mars-gravity assist into account?

Question

In studies pertaining Earth-Mars cyclers, purely ballistic cyclers are usually relegated to many orbit solutions. For instance the classic Aldrin cycler, while elegant, can't achieve the desired turn-angle at Earth without slamming into the ground. However, these studies, for simplicity sake, usually omit Mars as contributing to any gravity assists. A Mars encounter, despite its low mass, might change the tisserand parameter with respect to Earth just enough to allow a safe angle change during Earth flyby. Has someone done the math on this?

Answer 1

Earth-Mars-Earth trajectories are objectively possible, see the Rosetta mission and Europa Clipper. Mars' eccentricity and inclination causes problems finding periodic, repeating solutions (and is ignored in both papers linked).

If there exists a viable Mars gravity assist that brings the spacecraft back to Earth an integer multiple of the Earth-Mars synodic period (780 days) later & with a reasonable Earth $v_{\infty}$ value, then it might be possible to flyby Earth and reach Mars again.

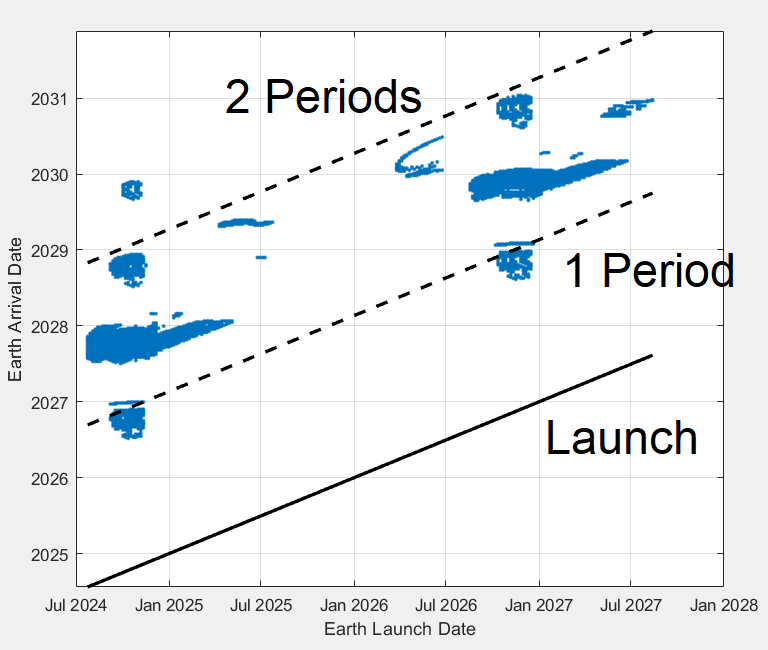
I've done this search and in the plot below we can see some potentially viable dates after one synodic period (first dashed line):

(Personal work)

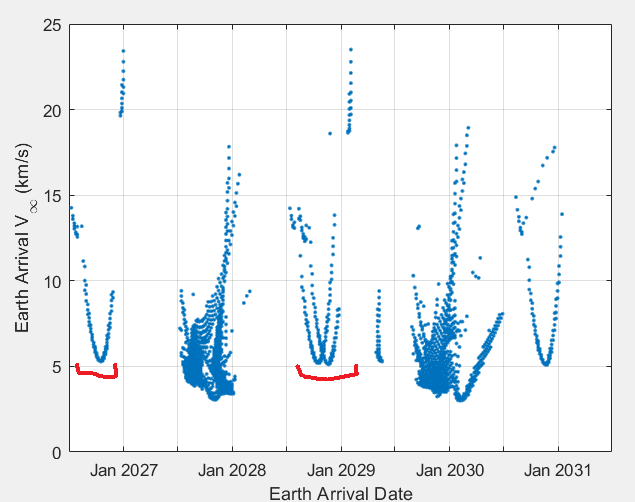
The Earth arrival $v_{\infty}$ from this search are:

(Personal work)

With the promising windows denoted in red. The range of these values is perhaps best described as "not great, not terrible" for getting back to Mars. Recall that a vessel must launch from Earth at these same $v_{\infty}$ to rendezvous with the cycler which are higher than what you would typically want for launching to Mars, but not too high.

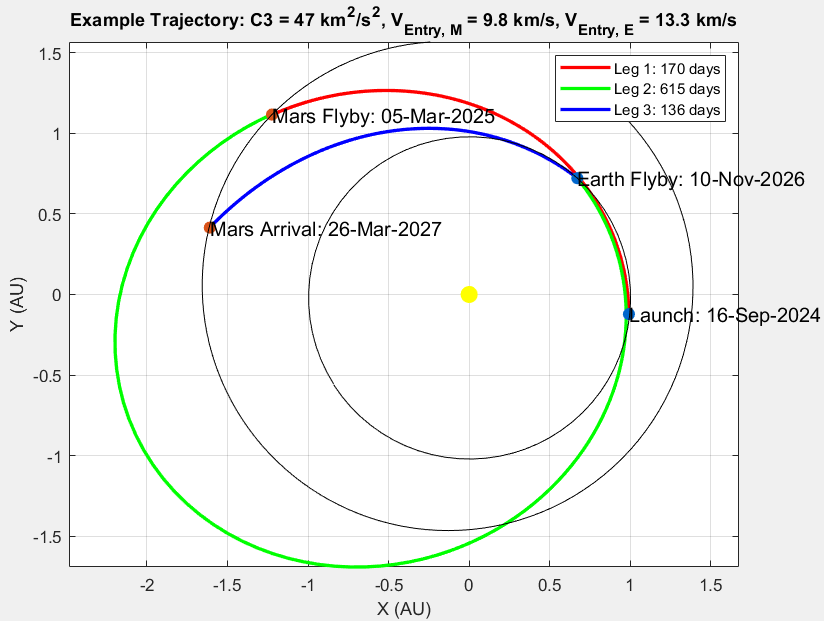
For a selected Earth-Mars-Earth trajectory shown below:

(Personal work)

The deflection angle at Mars is only ~6.5° (though this is due the high required flyby periapsis of ~85,000 km). The "design space" is characterized by short launch windows and inefficient trajectories. A typical C3 to launch towards Mars (during optimal window) is less than 20 $km^2/s^2$ and typical entry velocity is a little less than 6 km/s. The entry velocity at Earth is also higher than humans have ever demonstrated. Additionally, the second trip to Mars (leg 3) does not have the same time of flight as the first trip (leg 1), messing up the periodic-like solution we are looking for.

For this representative trajectory the turn angle at Earth is ~70° and the flyby periapsis is slightly below the GEO belt at 39,900 km radius.