Is the "airship to orbit" mission profile feasible?

Question

By one of those weird coincidences, I had been on JP Aerospace's site mere hours before reading this question about space dirigibles here. Their mission plan seems too good to be true.

They are proposing a three part infrastructure

Earth to the top of the atmosphere.
High Altitude airships fly from the ground to the station at 140,000 feet.

Transfer point at the edge of space.
A two mile wide station parked at 140,000 feet is the new way station to space. The station acts not only as a port for the orbital airship but also as a research center, construction site and tourist destination.

From the edge of space to orbit.
This 6,000 foot long vehicle never touches the ground. This airship flies from the upper atmospheric station to orbit. It uses hybrid chemical/electric propulsion to slowly accelerate and reach orbit.

L to R: Airship, Station and Orbitor (not to scale).

Set aside, for the moment, the engineering & technical challenges of establishing a permanent, crewed "suborbital space station" two miles wide at 140,000 feet. The company does, after all, hold the world's record for the highest flying airship. What really lifted my eyebrow was their Orbital Ascender:

This is a huge (a mile or more long) lighter-than-air craft to be assembled at 140,000 feet.

The airship uses buoyancy to climb to 200,000 feet. From there it uses hybrid electric/chemical propulsion to slowly accelerate. As it accelerate it dynamically climbs. In nearly nine hours it achieves orbital velocity.

They're a bit cagey about their "hybrid electric/chemical propulsion," but the ion engine (or whatever) is to be powered by solar cells overlaying most of the top surface.

Is there anything about high-altitude dynamics or orbital mechanics that renders the concept of an airship reaching LEO orbit unlikely? Or are there any other show-stoppers to this concept?

If you're interested, there's a book by the concept's originator:
Floating to Space: The Airship to Orbit Program, by John M. Powell (Apogee Books Space Series, 2008)

Answer 1

Nearly all balloons that have been constructed have been for flights from the surface to altitude. That requires a structure that can survive tethered at the surface in a range of wind speeds in high-density air, then expand to several times their original size in order to maintain lift as the air density decreases. The minimum density ends up being determined by the need to survive liftoff.

ATO is a two-stage system with the upper stage being constructed at 140k feet. Given that it will never be tethered to the ground, it will experience no wind at all, because the Dark Sky Station will be in exactly the same air the upper stage is in. That means the lower limit on its density ends up being determined by other factors such as differential air speeds at different parts of the structure. That could still be a killer, but "the thinnest balloons" (mentioned in Charles Pooley's answer, as being barely lighter than the 4 g/m³ of air at that altitude) is not relevant here, because the thinnest balloons anyone would have experienced must still be far denser than what you could build at 140k feet.

Lift is a function of drag. At any altitude where drag would prevent the upper stage from achieving orbital velocity for that altitude, the upper stage would continue to generate lift. This profile continues smoothly until the upper stage is close enough to orbital velocity that it no longer matters. It's possible that, at whatever altitudes you'd want to operate, the upper stage would need to generate thrust continuously, and anything you wanted in actual orbit would need an extra kick, but with solar-powered ion propulsion, that doesn't seem like much of an inconvenience.

ATO will run into plenty of challenges, but I'm not aware of any that are obvious killers. There are problems like radiation and the logistics of construction in full gravity and extremely low pressure, but I imagine the DSS and upper stages will end up being constructed by robots. Even if it only ends up being used for cargo, a "grown up" spacefaring society should be sending humans and cargo up separately anyway, because they have different requirements for speed and safety. Humans can wait on the ground until the cargo they work with is safely in orbit.

Answer 2

To float something at 140,000 feet (> 42 km) requires an impossibly low density structure.

At 40 km the density of air is less than 4 grams per cubic meter. Barely attainable with the lightest of balloons. The proposed added structures, engines etc make it quite impossible.

As to making a balloon structure ascend to orbit, again impossible. The drag will be orders of magnitude too great.

Answer 3

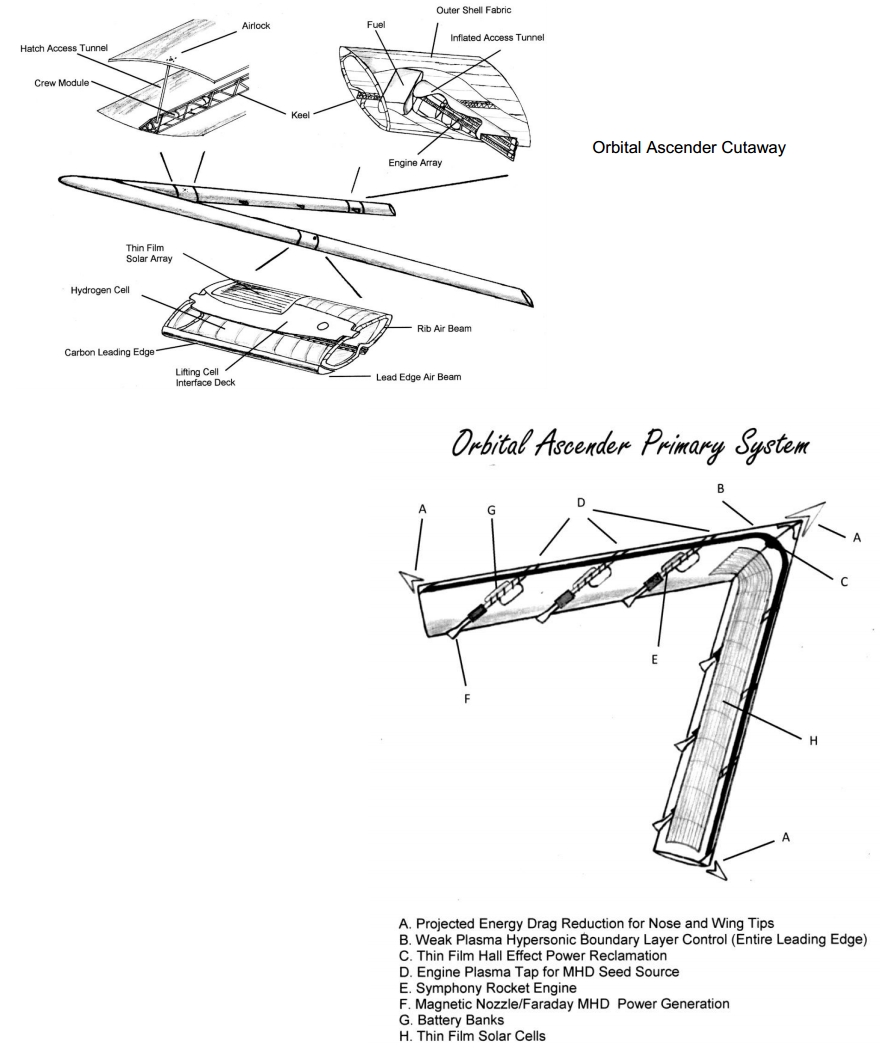
I think it's possible that the airship can go hypersonic which is already a major achievement if it does. If they can reach Mach 12 then it could be combined with momentum exchange tether assist to get to orbit. That's still a big ask though.

[

see also this paper

For details of HASTOL system see this paper.

I'm not so sure about accelerating to orbit because the size of their airship needed is so vast - it's a major challenge.

It doesn't help that his figures, which are given in feet, seem to need to be reinterpreted as meters to get the required buoyancy. E.g. he says his 6000 foot airship would be neutrally buoyant at 200,000 feet and carry 20 tons to orbit, but it works out as only enough buoyancy lift for 2.6 tons. If it is 6000 meters then it works out fine because you multiply all the numbers by 35 making it 91 tons making it much more feasible to take 20 tons to orbit even with skin, engine, crew, and fuel. See my Can JP Aerospace's Future Giant Airships Slowly Accelerate To Orbit? Looking At The Numbers

As for the strength of the airship - they plan to use airbeams and given its vast kilometer scale, and the very thin atmosphere - and that the skin is not intended to give structural support (a bit like the Skylon) I don't see why that would be impossible. The JAXA balloon skin is very strong - with a thickness of 3.4 microns it can withstand a breaking strain of 400 kilograms per square centimeter. It would seem to be way overkill for this application, given equal pressure inside and out. It's overkill for JAXA too - the main reason they have it so thick is because it is hard to make it uniform in thickness if it is very thin. So if that can be cracked, then the skin can be much thinner and still very strong.

As for meteorite impacts - then the outer skin is kept in equilibrium with the atmosphere outside and the inner lifting balloons are inflated to only 2 millibars. So if there is a breach in the lifting balloons - a small hole from a micrometeorite - the hydrogen will escape very slowly. They would be designed to be replaced easily - and also he could pump out the hydrogen to equalize pressure inside and out as it ascends for the lifting balloons too.

So anyway - I think the strength of the airship is a secondary issue. Other very important but secondary issues are the issue of maintainability - and of inflating it at a height of 140,000 feet.

Here I want to focus on what seem to be the most essential points - basic points in physics that have to work to make ot possible at all.

My conclusion is that if it can achieve a high lift to drag ratio through hypersonic flight and a high ISP ratio then it could be feasible. So let's look at this in detail

NEED FOR HIGH ISP

If it has an ISP of 2000 with his dirty ion thruster then so long as at most 50% of the rocket thrust is used to overcome drag, then it's an easy calculation for the total fuel to orbit. It has the same effect as doubling the delta v with an assumption of no drag.

So I just input double the delta v to orbit ( which we are taking as 7,844 m/s), or 15,688 m/s into the ideal rocket equation, assuming an isp of 2000, mass of, say 100 tons. It turns out that the dry mass you get to orbit is 44.9 tons. So you need 55.1 tons of fuel. Here is an ideal rocket equation calculator to check the calculations.

CONSIDERATIONS OF LIFT TO DRAG RATIO

You can also work out the acceleration needed for a given lift to drag ratio assuming at most half of the rocket thrust is used to overcome drag. For instance if the lift to drag ratio is 4, then if half of the thrust is used to overcome drag it needs a rocket capable of accelerating it at 1/2 g in free space, and the airship would accelerate at 1/4 g. Anything less than that and it's very wasteful of fuel and it would surely be worthwhile adding an extra engine to increase the thrust.

If half the thrust is used to overcome drag then that's the rocket equation with double the delta v to orbit we just used. If, say, two thirds of the thrust is used to overcome drag, that's equivalent to tripling the delta v to orbit in the dragless rocket equation.

Now, a 4 : 1 lift to drag ratio is reasonable if airships can be as good as a typical hypersonic plane. Skylon can achieve close to that. But 1/2 g of thrust is a big ask for his dirty ion thruster.

You can also work out the time to orbit from the lift to drag ratio - assuming half the thrust is used to overcome drag - and the lift to drag ratio from the time to orbit. The equations are:

Time to orbit from Lift to Drag ratio L:

7,844 * L / 9.80655 secs

Example, if the average lift to drag ratio is 4, the time to orbit from rest should be 7,844 / (9.80655/4) = 3199 seconds or about 53 minutes.

So now, suppose that our orbital airship can somehow manage a lift to drag ratio of 70 like the best glider, the ETA glider, and again uses 50% of its thrust to offset drag. Then it would need 1/35 g of thrust, and it would get to orbit in 7,844 *70 / 9.80655= 55991 seconds, or about 15 hours 33 minutes. (You can use this online tool to help convert seconds to hours and minutes quickly).

Lift to drag ratio from time to orbit:

L = t * 9.80655 / 7,844 where t is the time to get to orbit in seconds.

More generally, L = t * 9.80655 * (R-1) / 7,844 where R is the thrust to drag ratio.

Since JP Aerospace says their airship would take three days to get to orbit, this suggests a very high lift to drag ratio of over 300 - either that or they have a very low thrust to drag ratio of not much more than 1 which doesn't make a lot of sense to me.

The airship will automatically rise to whatever level in the atmosphere gives it the amount of drag corresponding to its lift to drag ratio. For instance, if it has a lift to drag ratio of 10 and a weight of 1,000,000 newtons, say, then if the drag is more than 100,000 newtons then the balance of forces will cause it to rise until it reaches a level in the atmosphere where the drag is no more than 100,000 newtons.

So, the higher the lift to drag ratio, the lower the drag, and so the lower the thrust needed. The total fuel is the same, so long as only half of the thrust is used to overcome drag. However their dirty ion thruster is going to be low thrust, so it needs a high lift.

Sadly he doesn't give any figures for its performance either. But if his targeted lift to drag ratio is indeed over 300 then it only needs to have enough thrust to accelerate at 1/150 g in free space. Which would indeed put it somewhere between a standard ion thruster and a chemical rocket.

HIGH LIFT TO DRAG RATIO AIRSHIPS?

So are such high lift to drag ratios possible?

I think it is hard to say because it is simply outside of any real world experience. There doesn't seem to be any literature to consult on this.

Airships can be counter intuitive even at subsonic speeds. The Airlander 10 has a huge cross section but the addition of those tiny fins is enough to let it achieve a lift to drag ratio of 3.8.

So what happens with a hypersonic airship? First of all there's the objection that it has to cross the sound barrier - but he answers this, saying that it will happen so high in the atmosphere at such low densities that it's not an issue.

So let's leave that for now (I discuss it some more in my article about it) and focus on what I see as the most fundamental issue - the lift to drag ratio.

The thing is that the orbital airship is designed as a wave rider. When flying at hypersonic speeds then it rides on top of the shock wave caused by its hypersonic flight. The shock wave actually touches its body beneath it. Then - he would have drag reduction technology that lifts the shock wave away from its nose. So there is little drag from the front, and the V shape is designed to be optimized as a wave rider.

A HYPERSONIC WAVE RIDER GETS LIFT FROM PRESSURE DIFFERENCE, NOT BULK AIR MOMENTUM CHANGE

I hadn't heard of a hypersonic wave rider until I read about it in JP's book, but it's a well established concept goes back to work that started in 1951 theoretically and has been tested many times in practice. Here is the wikipedia page about it with lots of links. It depends on "compression lift" which is described here

Then - it gets lift not so much from change of momentum of bulk air as it hits the skin, like a normal airship. Instead it gets it from the density and pressure difference between the air beneath it and the air above it.

So now bear in mind that his airships are very low density.

Using his figures as meters instead of feet, assuming a 100 ton airship - actually it's 91 tons for 6000 meters length and neutral buoyancy if the calculations are right - but let's assume 100 as a round number.

So, taking its weight in force units as 1,000,000 newtons, and the very low density, with the lifting bags inside pressurized to 2 millibars, I calculated his airship requires only 3.7 newtons per square meter upwards pressure to offset its weight completely.

That's using an estimate of the surface area of the lower part of the airship - the part in contact with the shockwave, as over 270,000 square meters (reading feet as meters again) - there I just multiplied the 6000 meters by 2 and by the width of 300 meters, and subtracted 300 * 300 for the overlap between the two wings, as an estimate for the area for only one side. It under estimates because the cross section is oval, not flat, so would be a bit more than that.

Since the excess pressure from the shockwave would be acting upwards only, it doesn't need to be much to offset the weight of the airship. It doesn't have to be as much as the neutral buoyancy pressure of 2 millibars at 200,000 foot.

Atmospheric pressure is 10.3 metric tons per square meter, or around 101,000 newtons per square meter. So his 3.7 newtons corresponds to an overpressure of 0.0366 millibars. Just a tiny overpressure in the shockwave would be enough to offset the entire weight of his airship.

When you start to think about it like that - an ultralightweight hypersonic waverider presenting a huge surface area to the shockwave and traveling in the upper atmosphere in what would normally be called a hard vacuum - well it is something totally out of any normal aerodynamic experience.

So - could he achieve high lift to drag ratios in this way - far better even than our best gliders?

Although he never talks about the lift to drag ratio anywhere, it seems from these simple arguments that he must be targeting a very high lift to drag ratio for the concept to work.

OPEN QUESTION - IS SUCH A HIGH LIFT TO DRAG RATIO POSSIBLE FOR A HYPERSONIC WAVERIDER

This is a very unusual situation of an ultra lightweight hypersonic wave rider operating in air so thin it would count as a hard vacuum. Could it have a high lift to drag ratio. I leave this as an open question - I don't know the answer and haven't found any research papers that tackle the question in this unusual physics setting.

So - that's my main insight into it. The calculation of the buoyancy is due to James Fincannon though the rest of this here is my own work and I wouldn't like to suggest he supports any of the rest of it. The ideas here come out from a very stimulating discussion with him in the SpaceShow comments section

More background and calculations. Can JP Aerospace's Future Giant Airships Slowly Accelerate To Orbit? Looking At The Numbers