In many online resources, like Wikipedia, five Lagrange points are specified. From my understanding, a Lagrange point is a point where the gravitational attractions of the two bodies (Sun and Earth, for example) cancel themselves. This can be easy to see in the case of L4 and L5, where the distances to Earth and Sun are equal. Considering this, why can't Lagrange be points outside of the elliptic plane, or on the line connecting L4 and L5?
$\begingroup$
$\endgroup$
1
-
$\begingroup$ There are three accelerations: gravity of central body, gravity of orbiting body and inertia in a rotating frame (what we used to call centrifugal acceleration). $\endgroup$– HopDavidCommented Jul 23, 2015 at 23:58
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
4
The points can't be out of the plane of the orbit because then the combined gravity at that point would be pulling toward the plane instead of being balanced.
This diagram shows the contours of the gravitational attraction for a two-body system:
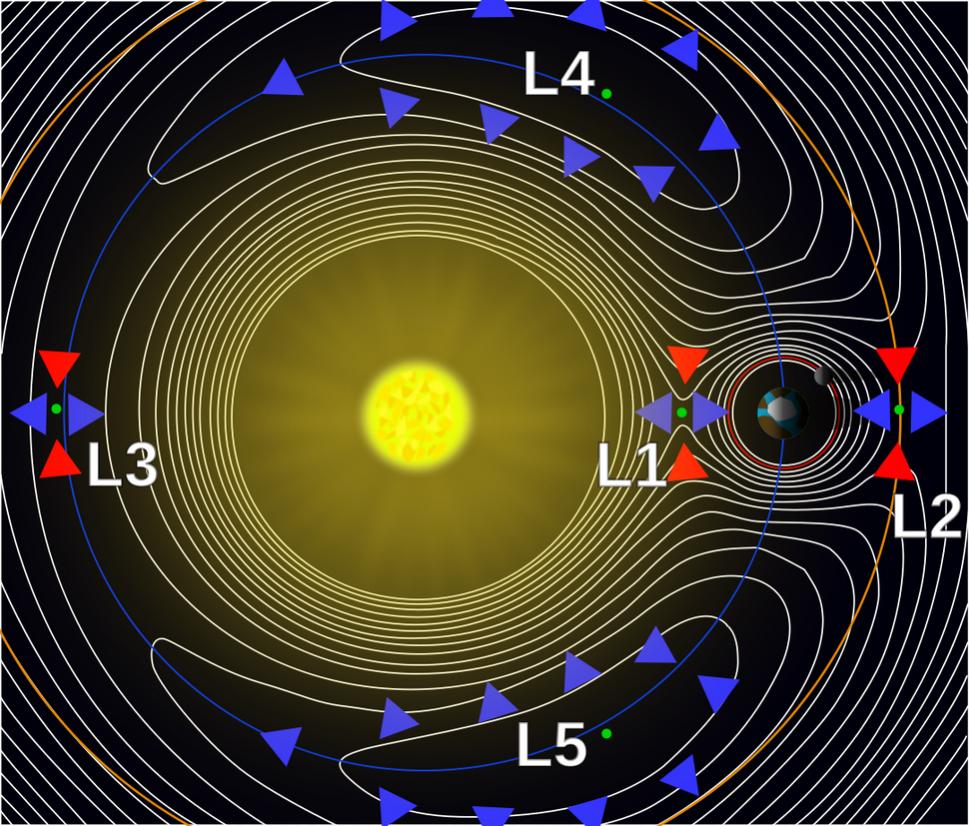
The points where forces are balanced are at the saddles and peaks (reading it like a topographical map). Between L4 and L5 would be more attracted to the sun or the earth (except for L1 where those forces are balanced).
I should add that none of the Lagrange points is completely stable; the best you can get is stable in one dimension at L1, L2, and L3 (indicated by the red arrows in the diagram). That means that a slight offset in the direction of a blue arrow will cause you to start drifting that direction away from the Lagrange point you started at, so you still have to do some station-keeping to maintain your position. The closeness of the contours indicates how much station keeping you would need to do to maintain the orbit relative to the sun/Earth. While there are only the 5 Lagrange points the gentle slope around L4 and L5 means that there are large regions there where not much station keeping is required to maintain relative position (the points are the absolute minimum).
-
$\begingroup$ Things are getting weird when one allows for solar pressure and starts looking at stability of solar sailcraft. $\endgroup$ Commented Jul 22, 2015 at 16:27
-
2$\begingroup$ Lagrange points are defined solely with respect to gravity, once you start adding thrust (such as solar pressure on a sail) how you use the Lagrange points may change but the points themselves don't. $\endgroup$– 1337joeCommented Jul 22, 2015 at 16:31
-
1$\begingroup$ yeah, that's why I didn't use the L-word in my comment :) $\endgroup$ Commented Jul 22, 2015 at 16:33
-
2$\begingroup$ The L4 and L5 points are stable in the proper approximation-two very massive bodies, one low mass body, nothing else around. Some discusion is here $\endgroup$ Commented Jul 23, 2015 at 3:49