5
$\begingroup$

I know this is a bit of apples-to-oranges comparison, since bi-elliptic transfer is a purely mathematical construct in a two-body problem, while ITN is a great "maze of opportunities" based on the immense complexity of the solar system, that is largely time-dependent, and finding an optimal trajectory (as opposed to just a good one) may be an NP-hard problem.

Still, a rough estimate? Say, how much delta-V would one save/lose going to Saturn through a bi-elliptic transfer vs ITN, willing to spend, say, 30 years? Or some similar figure, that gives a rough hunch which one may be preferable in what conditions?

Improve this question
$\endgroup$
6
  • $\begingroup$ Is there any (interplanetary) ITN at all? Isn't the use of Lagrange points restricted to cis-Lunar space? How could a Lagrange point help a mission to Saturn? $\endgroup$
    – LocalFluff
    Commented Apr 1, 2016 at 14:39
  • $\begingroup$ @LocalFluff: ITN stands for interplanetary transfer network. It definitely exists, and if you spend some time searching space.SE you'll find a proposed asteroid capture for use as a shuttle between Earth and Mars using ITN. $\endgroup$
    – SF.
    Commented Apr 1, 2016 at 15:27
  • $\begingroup$ @LocalFluff: The confusion may come from the fact, that the interplanetary mainfolds possibly don't come into contact with each other, which means there may be no free connections where infinitesimal nudges suffice, between planets. But the gravity well separating them is really shallow. ITN isn't free, it's just very cheap. $\endgroup$
    – SF.
    Commented Apr 1, 2016 at 15:36
  • $\begingroup$ I don't do this math, someone else will give a proper answer. But since you use ITN as a reference level, maybe it would be helpful to include an example in the question (unless I'm alone in being confused). I would think that the shallowness between the gravity wells of Earth and Mars varies conventionally with their eccentricities and periods. How would going from a Sun-Earth L-point to a Sun-Mars L-point gain any dV compared with surface to surface Hohmann style? $\endgroup$
    – LocalFluff
    Commented Apr 1, 2016 at 16:55
  • $\begingroup$ @SF. Indeed, the Mars and earth manifolds don't overlap, as Toppotu has noted. Belbruno and Toppotu have written a paper describing a conventional 3.6 km/s LEO to TMI burn and a 2 km/s aphelion burn to inject into a WSB leading to Mars capture. Unfortunately for Belbruno, Mars capture can be acheived for as little as .7 km/s. The Belbruno Toppotu route is 1.3 km/s more expensive than Mars capture via Hohmann route. $\endgroup$
    – HopDavid
    Commented Apr 1, 2016 at 19:53

1 Answer 1

Reset to default
5
$\begingroup$

To enjoy a bi-elliptic savings, destination needs to be greater than destination orbit by a factor of 11.94 or greater.

Going from LEO to the moon certainly qualifies: 384,400/6678 = ~58.

I'll pull a bi-elliptic path out of the hat, don't know if it's optimal: 300 km altitude circular orbit to a 900,000 km altitude apogee: 3.16 km/s

Apogee burn at 900,000 km to raise perihelion to 384,400 km: .43 km/s

Circularize burn at 384400 perihelion: .18 km/s.

So a total of about 3.8 km/s. It's noteworthy that Farquhar's more direct route reaches EML2 for around 3.4 km/s

If you time your burn from LEO to land near a certain region of earth's Hill Sphere, the sun's tidal influence can raise your perihelion to 443,000 km (the neighborhood of EML2). From there the ship can do a ballistic slide into EML2. I describe such a path in EML2 blog post. This can take as little as 3.1 km/s.

So for LEO to EML2 a .7 km/s savings can be enjoyed over bi-elliptic and a .3 km/s savings over the Farquhar route.

Saturn's orbit is less than 11.94 A.U., so no bi-elliptic savings.

But there is no ITN route from earth to Saturn or even from earth to Mars or Venus.

"What?" you may ask. "How come the earth-moon-spaceship 3-body system confers savings while the sun-rocky planet-spaceship 3-body doesn't" The difference is in the 3 body mass parameter.

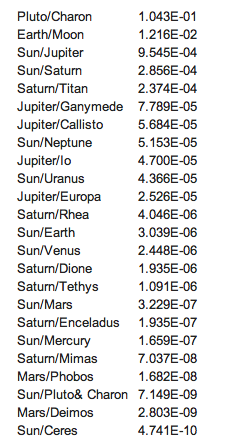
enter image description here

All kinds of interesting trajectories emanate from the Pluto/Charon L1 and L2. Ditto Earth/Moon L1 and L2 as well as gas giant/big moons L1 and L2

But the sun/small rocky planets mass parameters are in the millionths to hundred millionths. I don't believe there are any interesting WSBs leading from the sun earth L2 to Mars or any other planet. At least none that take less then centuries to arrive at the destination.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.