How can delta-v and aerobraking as shown in this chart be explained?

Question

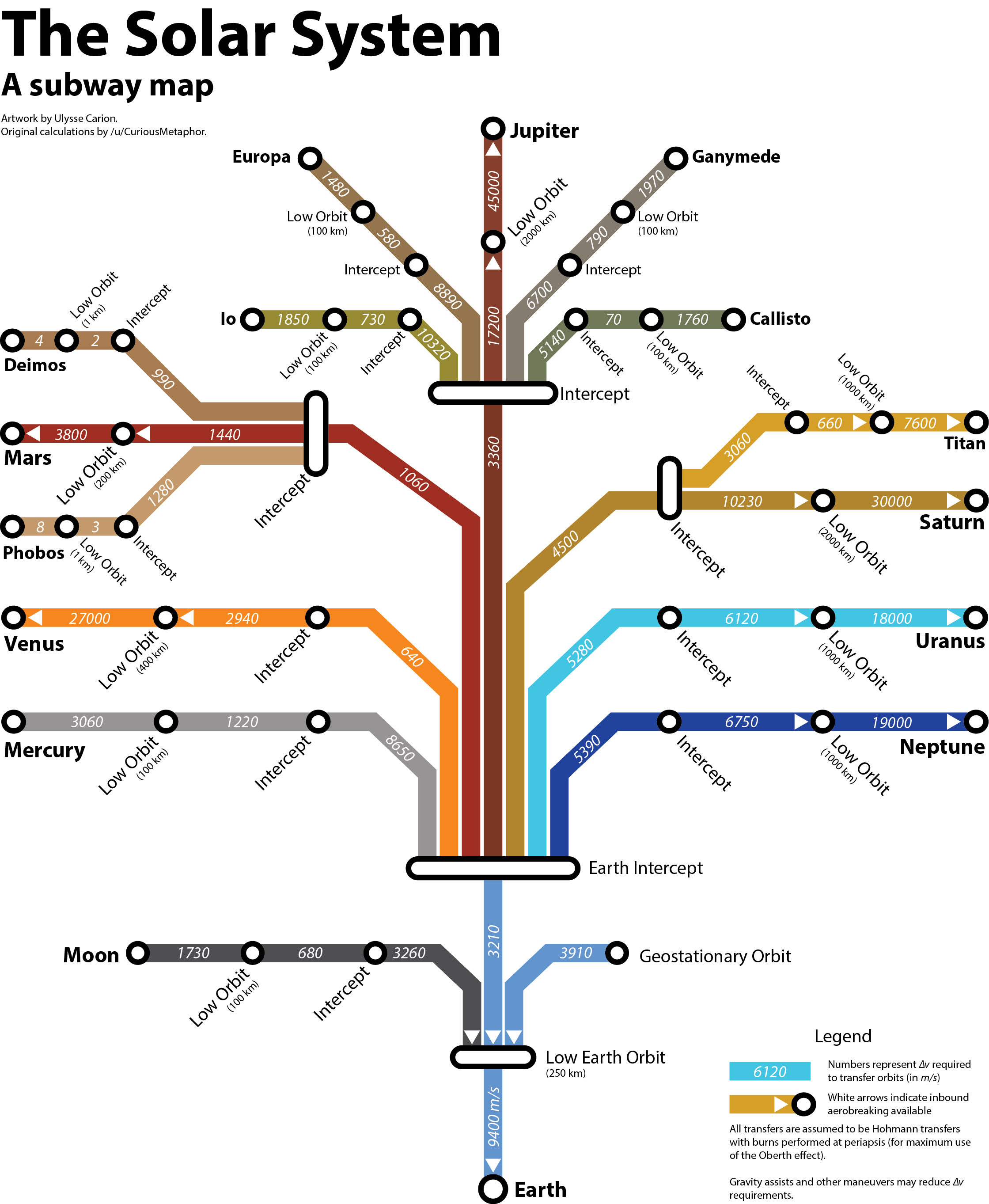
The following diagram was included in Rikki-Tiki-Tavis' answer to this fine question of a few hours ago.

I am hoping somebody can explain some of the questions I have about this data. It shouldn't matter which direction you are travelling, the numbers are supposed to indicate delta-v in transferring from one spot or orbit to another.

Some strange things I want to observe about this data are:

1) The value between Venus and Venus Low orbit is 27000 while the value between Earth and earth low orbit is 9400. That's almost 3 factors difference. The escape velocities of the two planets however, are nearly identical (Earth's is roughly 11,200 m/sec and Venus's is roughly 10,200 m/sec). How can the delta-vs be so much farther apart?

2) The arrows and their explicit direction are supposed to indicate that "inbound aerobraking is available". Yet these arrows are placed 250km above Earth and 400km above Venus. Those would be the upper limits of the braking as I understand it (the top of the atmosphere) and any potential braking would drop off sharply above that. Yet, several people have contradicted my interpretation of the data as though the transition from intercept to low orbit is going to provide much braking.

3) other users also seem convinced that when the delta-v is associated with aerobraking, that 100% of it can necessarily be applied as braking.

I am hoping that these issues can be better clarified, specifically with regards to how the numbers and arrows in the chart are to be interpretted, maybe with an example fly-thru from Earth to another planet and back, ignoring the obvious problem of fuel capacity.

Perhaps if the actual source of this data was identified, I would be less confused.

Answer 1

Here's the original source of the diagram:

https://www.reddit.com/r/space/comments/29cxi6/i_made_a_deltav_subway_map_of_the_solar_system/

Your concerns are also discussed there.

27000m/s is a very theoretical value, that takes into account the losses of a chemical rocket escaping the thick atmosphere of Venus. This makes it clear that launching a rocket from Venus is impractical to say the least.

For the precise example of the question why early planetary exploration (especially in the Soviet Union) had such a focus on Venus, it is only relevant to calculate the delta-v required to intercept Venus. Unless you want to orbit Venus, it is not necessary to expend any further fuel. A robotic probe can just slam into the atmosphere with interplanetary speed.

For example, the Russian Wikipedia says that the Venera Probes did just that and entered the atmosphere with 11km/s. This caused a deceleration exceeding 300G and a heat shield temperature of 11,000 °C.

Answer 2

The calculations for Ulysse Carion's chart were done by Curious Metaphor. If you go the this Reddit thread, you'll see a conversation between Curious Metaphor and I. For years I made delta V charts where it took about the same to escape earth and Venus. But Metaphor pointed out that Venus has a much thicker atmosphere.

But who would go to the surface of Venus? My earlier delta V maps assumed Venusians were arriving at and departing from the strata of Venus' atmosphere where temperature and pressure are hospitable to humans.

So acknowledging Metaphor's point, I redid my delta V map with "Landis Land" included enroute to Venus' surface. I call the hospitable atmosphere layers "Landis Land" since it was Geoffrey Landis that popularized the notion of humans inhabiting the floating cloud cities on Venus.

Regarding aerobraking, on each branch with a red vine there's a smaller number. For example look at the Phobos to Mars branch. See a .6 near where the red vine exits? It would take a .6 km/s deceleration to drop the periaerion low enough to feel substantial friction from aerobraking.

This delta V map is described in more detail at my blog post Cartoon Delta V Map.

What Metaphor and Carion call "Intercept", I call "Capture" (I believe). My capture orbits have peri apsis 300 km above the planet's surface and a apoapsis just within the planet's sphere of influence. This is an elliptical orbit that would only take a tiny nudge to achieve an escape orbit.

Answer 3

Adding to @Rikki-Tikki-Tavi answer.

For destinations where aerobraking is possible, and you have a sufficiently heat and acceleration hardened vehicle, it's possible to cheat. The map shows how much delta-v must be applied, not where it has to come from. The single most fuel efficient way of shedding speed is to smack into an atmosphere blunt end first.

To reach venus surface with a well hardened vehicle, you don't enter orbit at all. Go for a direct re-entry from an interplanetary transfer orbit. This saves you the costs of capture, transfer to low orbit, and re-entry, leaving only the cost to reach a venus intercept.

To achieve this, you have to hit a fairly narrow window between skipping out of the atmosphere without slowing down enough, and burning up.

Answer 4

A 250km periapsis Earth orbit won't produce significant atmospheric drag. The inbound-aerobraking-possible notations for orbits are assuming that you dip lower into the atmosphere in order to slow down into an eccentric orbit, which you then circularize once you've come back up out of the atmosphere. This is tricky; if you hit the atmosphere too high, you won't slow down enough to close your orbit, but if you hit the atmosphere too low, you'll lose too much speed and fall to the surface.