Yes, a cubic celestial would most certainly have anomalous gravity depending on your position relative to its sides. Gravity anomalies are however a perfectly normal thing in space travel, and I'm not aware of any celestials (including the Earth) that would have a seamless gravitational field throughout its surface. Reasons for this could vary, but in simplest terms possible, we could say that:
- The body is not a perfect sphere, and
- The mass of the body is not equally distributed (inhomogeneous)
Any of these properties of a celestial will affect the surface gravity to vary depending on your position relative to its surface. For example, our moon - Luna - was mapped for gravitational fields over its surface to high detail by the GRAIL (Gravity Recovery and Interior Laboratory) probe, and this is an example of the resulting map:

Gravity map of the Moon as mapped by the NASA's Discovery Program GRAIL probe (Source: Wikipedia)
So we have established, that a non-varying gravity over the surface of celestial objects is a normal thing. Great. What we now have to do is convince you that when we say x orbits y what we actually mean is that x and y orbit each other, albeit it might appear to a casual observer that since gravitational pull of one object is much larger than that of the other, one is dominant, and the other submissive in their role in this cosmic dance. To somewhat show what I mean, here are 3 models of two celestial object orbiting one another:
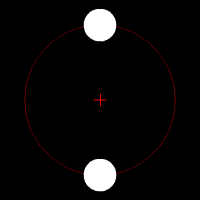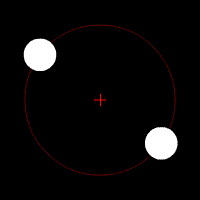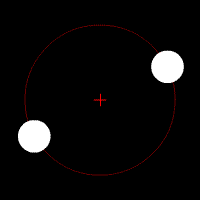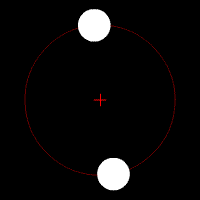
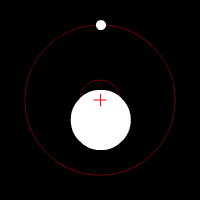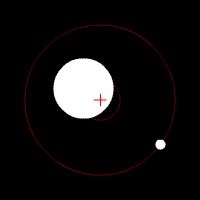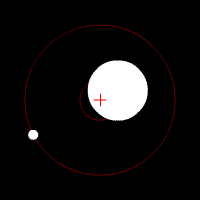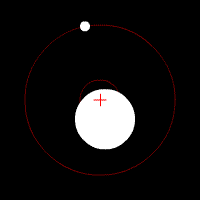
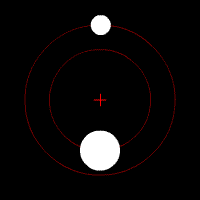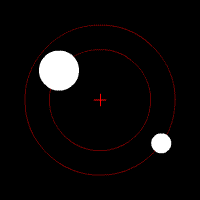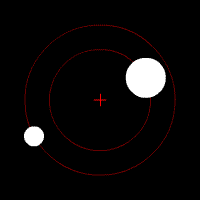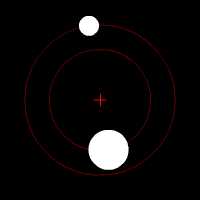
Cosmic dance: Two bodies with different mass ratios orbiting around a common barycenter. (Animations: Wikipedia)
Both these explanations, hopefully demonstrated well enough for the purpose, will serve us later when we describe an orbit of the cube shaped celestial. We'll assume these three conditions are true, to simplify things a bit:
- The body's is homogeneous, i.e. made of same material throughout,
- The body's material is non-compressible by its own weight, and
- The body's equator is going along the middle of the four of its faces
This simplification is important, because we don't want the cube's own mass to flatten its gravity along its surface closer to what it would be, if it was a perfect sphere, and we intentionally exaggerate the effects of its shape for the sake of this demonstration. The cube's gravitational pull would of course still point to a single cube's center of mass, like so:
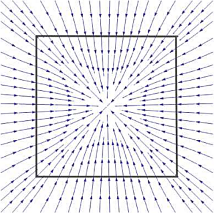
Gravity field through a cube sliced in half through the faces.
We can observe the slight distortion of the field lines between the edges and the center of each face, and this is exactly what we want. If we compared the gravitational potential along the equator of the cube to the one of a perfect sphere of the same volume, we would get:
- Cube's gravitational potential over its corners is higher than nominal, and
- Cube's gravitational potential over the center of any of its face is lower than nominal
Where with "nominal", I mean the gravitational potential of the perfect sphere that is equally distributed along its equator. If we transferred these gravity potential values into a graph, we would get something similar to a sine wave for the cube, and a straight line for the sphere, as we move along its equator. So each cube's corner would translate to a higher gravitational potential, and each center of its sides to a lower one than our nominal gravitational potential of the sphere. Assuming our cube has the same mass and volume as the Earth does, we'd get such a diagram:
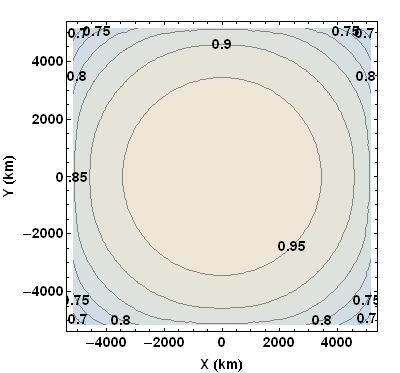
The force of gravity on the cube surface, in Earth g's. (Source: If the Earth Were a Cube)
OK, I'm probably already losing your patience, but I believe we've established our parameters well enough and somewhat described why they might not equally apply in all cases, so let's press on with answering your questions more directly:
Would something weigh more/less in certain areas over other areas?
Yes. Assuming all other parameters described above don't magically cancel each other out (and why wouldn't we, mountains as high to protrude the thickness of the Earth's atmosphere many times over and at the same time both themselves and an equal weight worth of their basin made out of marshmallow sitting in an otherwise chocolaty planet is sadly hard to come by in the real Universe), the certain areas of a cube shaped celestial would have higher gravity potential than others. Large part of this is already explained in the introductory text.
Would it be possible to slingshot objects around something non-spherical to change their velocity?
Again - yes. But the difference in gravitational potential along the body's surface would somewhat affect your slingshot vector, so it's something that would need adjustment for. This is of course assuming the body doesn't rotate on its axis at approximately the same radial velocity as your average rate of the change of your vector when you slingshot past its equator (i.e. the body is more or less showing the same face towards your vehicle as it flies past it). You would also want to do your slingshot in as few passes around the body as possible, since your orbit will decay towards the direction the cube's body is moving. Eventually, you would hit it, and your orbit might look something like this:
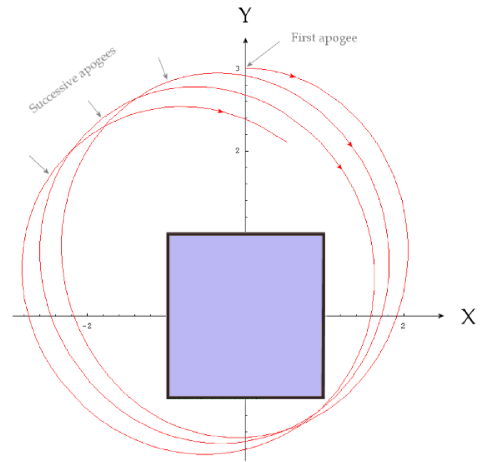
A satellite orbiting around the equator of a cube. Eventually, it will hit the celestial.
If this massive object was orbiting our sun, would it's orbit be as uniform as ours? if not what would that look like and why?
Provided the body is not tidally locked with the Sun that it orbits, always showing it same face and with it not change the gravitational potential oriented towards the Sun, then this periodic change in the gravitational potential will reflect in a slight "wobble" in the orbit for both bodies, more pronounced for the one with on average lower gravitational potential. As the cube celestial rotates on its own axis to face with one of its corners towards the body it rotates, this gravitational potential will slightly increase, moving the bodies slightly closer to one another, and the opposite as the cube body shows towards the other celestial it rotates one of its sides. If the masses of the two bodies orbiting each other would approximately match, this "wobble" would look a bit like one energetic drunkard dancing around another, perhaps slightly less energetic drunkard (depending on their mass distribution).
So this is about it. Hopefully not too difficult to imagine and easy enough to understand, as per your request to keep the answers simple. If you're interested in reading slightly heavier to read sources on this same presented situation of a cubic celestial in orbit of another celestial (or vice versa), here are some external resources:






