The Hohmann orbit tangent to both LEO and GEO would have an perigee of 6678 km and an apogee of 42164 km.
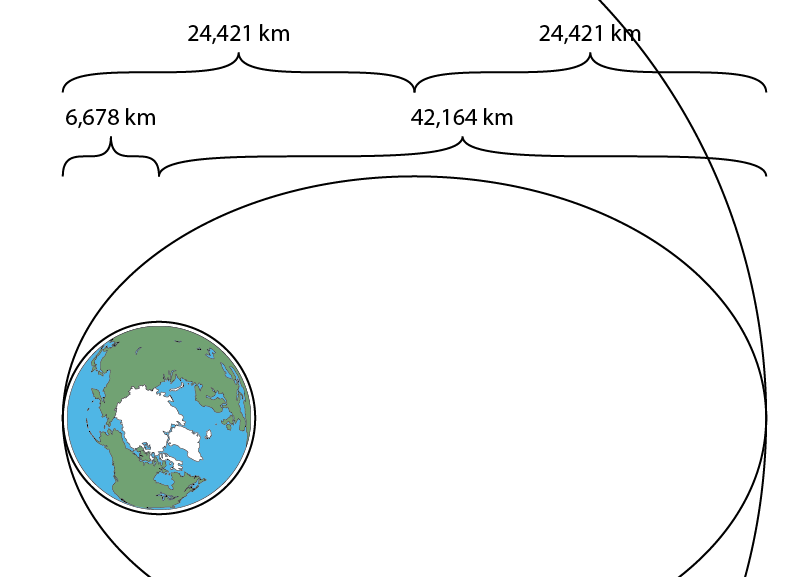
I hope it's evident that the semi-major axis, a, of this transfer orbit is (6678 km + 42,216)/2.
The vis viva equation can be used to calculate the speed of a spacecraft on this orbit provided you know the distance from earth's center, r.
$v=\sqrt{GM(2/r - 1/a)}$
G=Gravitational Constant
M=Mass of Earth (see right column under Physical characteristics)
a=24,421 km for this transfer orbit.
r=6,678 km when ship's at perigee and 42,164 km at apogee
So there's the tools to get transfer orbit speed at perigee and apogee.
But what's the speed of the LEO and GEO orbits?
Well, with a circular orbit, r = a.
$v=\sqrt{GM(2/r - 1/a)}$
Substituting a = r,
$v=\sqrt{GM(2/r - 1/r)}$
reduces to
$v=\sqrt{GM/r}$
You can use the above to get speeds of both LEO and GEO orbits. At LEO r=6678, at GEO r=42,164.
The burn from LEO would be transfer orbit's perigee speed minus LEO speed. The circularization burn at apogee would be GEO orbit speed minus the transfer orbit's speed at apogee.
I hope I've given you enough to work this out on your own.