In Wikipedia's article SpaceX Falcon-Heavy the section titled Capabilities has a table comparing maximum payload masses to Falcon 9 for different payload destination. The current numbers there are shown below.
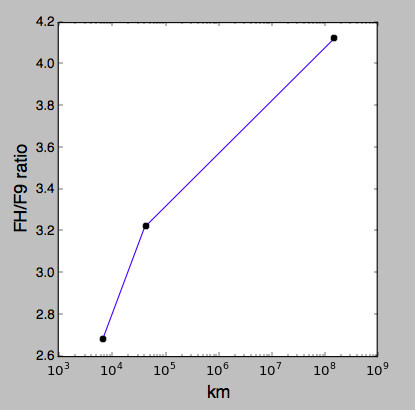
There is probably some fundamental, easy to grasp reason why Heavy can bring 4.2 times as much mass to Mars than F9, but only 3.2 times as much to GTO and only 2.7 times as much to LEO, but I don't know what that would be.
Payload Falcon Heavy Falcon 9 Ratio
LEO (28,5°) 63,800 kg 22,800 kg 2.68
GTO (27°) 26,700 kg 8,300 kg 3.22
GTO (27°) Reusable 8,000 kg 5,500 kg 1.45 - does not apply here
Mars 16,800 kg 4,020 kg 4.18
Pluto 3,500 kg - -
below: rough plot of ratio versus "altitude" of the three (presumably non-reusable) data points, just to show there is a trend.
below: Artist conception of a Falcon Heavy, from here.