Difference between Tundra and Molniya orbits?

Question

This excellent answer nicely describes both Tundra orbits and Molniya orbits. They are both repeat groundtrack orbits with periods of rational fractions of a sidereal day, and both have inclinations of $\arcsin(\sqrt{4/5}) \approx 63.4°$ to zero the precession of the argument of perigee.

The linked articles say that Tundra orbits have eccentricity of about 0.2 to 0.3 (though QZSS are only about 0.075) and Molniya orbits are about 0.7.

Question: What are the orbital mechanical and functional differences between these two classes of orbits? Is a Molniya the same as a Tundra, except that the eccentricity of the latter is high enough to be useful to Moscow while the Tundra is good enough for Tokyo (e.g. QZSS, see also the orbit)? Or are there other meaningful distinctions?

Answer 1

They are definitely not identical.

Tundra is geosynchronous; period = 1 day. The eccentricity allows it to spend most of the time over a region of Earth off the equator, something not possible for geostationary (sit always over one point on equator) or circular geosynchronous orbits (sinusoidal function with slow-down at both extremes). Tundra is intended for a single satellite to observe one specific point of Earth.

Molniya is an orbit intended for 3-satellite constellations and it has a period of half a day. It's also used to observe two off-equator regions of Earth, 180 degrees of longitude apart. It utilizes the similar "dwelling" effect of a satellite staying for a long time near apogee, above roughly the same region of Earth (not nearly as narrow as in case of Tundra though), and by the time the satellite leaves the apogee, another from the constellation approaches it, and enters region of interest for the observation.

The most important part is about the trajectory over ground:

Tundra:

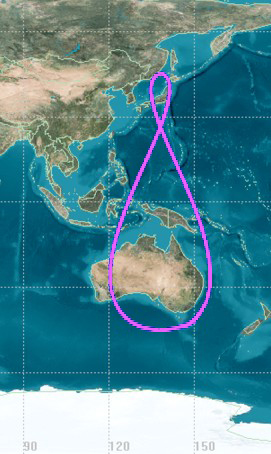
Molniya:

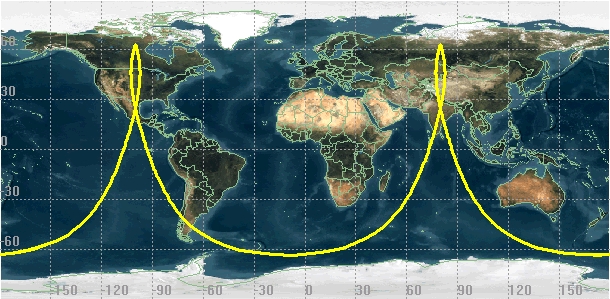
In Tundra, the satellite spends most time over the "narrow" top part of the figure 8, then dashes through the lower part, to return to the top.

In Molniya, there are three satellites on exactly the same track over ground, 1/3 period apart. They dash through the long southern stretches, then dwell on the northern "peaks".

Due to Earth rotation, the ground track rotates, so their RAAN are actually 120 degrees apart; also, their true anomaly is such, that their epoch would differ +8/0/-8 hours if the true anomaly was identical.

Summing up:

Orbital:

Tundra: 1 satellite, 1 day period, 0.2 to 0.3 eccentricity.

Molniya: 3 satellites, 12h period, 0.7 eccentricity, RAAN and True Anomaly spread 1/3 period apart.

Functional:

Tundra: observing one point of Earth, for most of the time. (also possible to use two or three satellites for 100% time coverage).

Molniya: observing two areas on Earth 180 degrees apart, at all times.

As for main technical differences, Molniya requires three separate launches, but Tundra requires a stronger booster to reach the higher orbit. Also, Tundra's somewhat higher apogee may require stronger radio connection or result in worse results due to higher distance; the difference is not big though (about 15%).

Answer 2

This is an addendum to SF.'s already excellent answer regarding why such orbits are inclined by roughly 63.4 degrees.

That Earth's oblateness causes a satellite's node to precess is a well known effect. A lesser known effect is that the Earth's oblateness also causes a satellite's argument of perigee to precess. This apsidal precession is essentially meaningless for satellites in a nearly circular orbit. Apsidal precession is rather important for a satellite in a highly eccentric orbit, a feature common to tundra and Molniya orbits. Both of these types of orbits depend on having apogee occur at the northernmost extent of the orbit. Such satellites must either fight apsidal precession with large amounts of fuel for orbit maintenance or they must be placed in an orbit where apsidal precession is strongly reduced.

While the $J_2$ effect on nodal precession is proportional to $\cos i$, where $i$ is the satellite's inclination with respect to the Earth's orbital plane, the $J_2$ effect on apsidal precession is proportional to $5\sin^2i-4$. This makes an inclination of $\arcsin\left(\sqrt{4/5}\right)=\arccos\left(\sqrt{1/5}\right)=\arctan(2)$ be rather critical. This inclination results in zero apsidal precession from $J_2$. For this reason and others this is called the critical inclination.