9
$\begingroup$

Having never been to a rocket launch, can you tell me how far the sound carries?

I can hear the rumble of F-15 Eagles take off from inside my office building five miles from the airport. An F-15C is about 149 dB with afterburners.

The space shuttle and Saturn V rockets were much louder. A test of the Saturn V Rocket reached 204 decibels.

Is there a standard distance from Cape Canaveral that launches can be heard?

Improve this question
$\endgroup$
8
  • 2
    $\begingroup$ If there's a cloud deck, a reasonable sized launch from Cape Canaveral will travel approximately 50 miles along the coast line. The shuttle launches had enough force to rattle my garage door. $\endgroup$
    – fred_dot_u
    Commented Feb 2, 2018 at 10:07
  • 1
    $\begingroup$ @OrganicMarble I would imagine those rules would require that launches over a certain noise level are only allowed during certain daylight hours. $\endgroup$
    – Dan Sorensen
    Commented Feb 2, 2018 at 16:21
  • 1
    $\begingroup$ @DanSorensen I believe the rules called for scrubbing if the predicted noise/sound power levels were too high in the surrounding communities (based on weather conditions at the predicted time of launch). I don't think this ever happened though so the margins were presumably generous. I was hoping to find a copy of those rules somewhere on the internet to set an upper bound for the sound levels in your question, but so far I have not been able to. $\endgroup$
    – Organic Marble
    Commented Feb 2, 2018 at 16:47
  • 3
    $\begingroup$ The answer is probably in nasa.gov/pdf/207914main_Cx_PEIS_final_Chapter_4.pdf somewhere. My favorite part is on page 4-14: 80 dBA is "Moderate hearing damage", 85 is "Very annoying", and 90 is "Affect mental and motor behavior". $\endgroup$
    – Phil Frost
    Commented Feb 3, 2018 at 21:05
  • 2
    $\begingroup$ Also keep in mind when you read "F-15C is about 149 dB with afterburners", that's 149 dB at some distance. If the distance isn't specified it doesn't really mean much. My cat can purr at 65 dBA (at least) if I put her throat right on my ear. $\endgroup$
    – Phil Frost
    Commented Feb 3, 2018 at 21:09

2 Answers 2

Reset to default
2
$\begingroup$

Normally, if the soundwaves are omnidirectional in three-dimensional space, the power of the sound will decay with $1/r^2$, where '$r$' is the distance from the source of the sound to the listener. However, if the sound is confined within a waveguide its rate of decay versus distance will be slower. The ground, ocean, and cloud cover create an imperfect waveguide for the sound of a rocket. But if the ground, water, and clouds were perfect reflectors then theoretically the sound would begin to spread out more and more within a plane. The decay rate will begin to approach $1/r$.

But, at the risk of being down-voted by flat-earthers, the earth is not flat, so as the sound travels around the earth its rate of decay drops further. At the point where it has traveled 1/4 of the way around the earth, it is no longer spreading out. At this point, all of the sound is traveling in the same direction and it is (again theoretically) confined within an annular region bounded by the ground or ocean below and the clouds above. From this point on, the sound will become more concentrated until at the exact opposite side of the planet from the launch site (the antipodal point), it will reach a local maximum in intensity - again theoretically.

In practice, there is some evidence to support this. The eruption of Krakatoa in 1883 was heard 4800 km away and recorded by baraographs around the world. According to Wikipedia

Several barographs recorded the wave seven times over the course of five days: four times with the wave travelling away from the volcano to its antipodal point, and three times travelling back to the volcano the wave rounded the globe three and a half times.

Now the sound of Krakatoa was estimated to be 310dB SPL.

Starship's loudness was studied by the FAA.

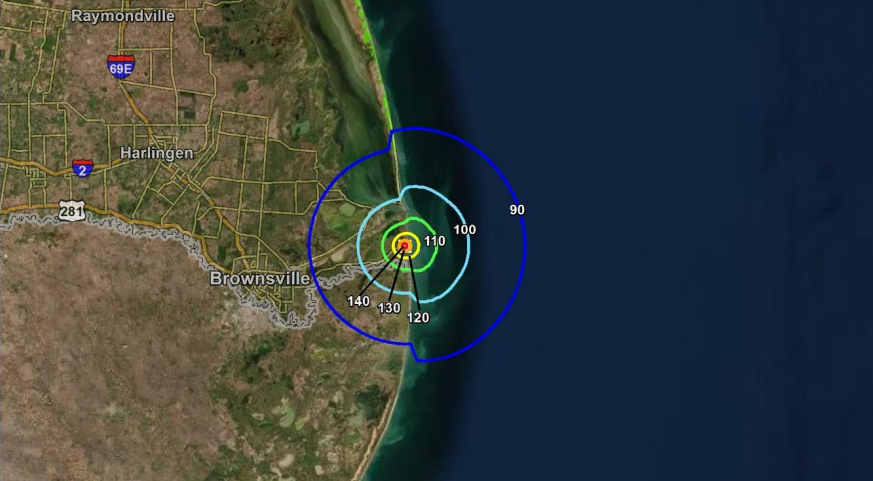
enter image description here

You can observe that the distance between the colored rings increases as the distance increases. I think this is due to the shift from $1/r^2$ behavior to $1/r$ behavior. You will also observe that the sound travels further over water than over land.

The report provided lots of different simulation results, so it's probably worth giving it a thorough read if you want to understand this well.

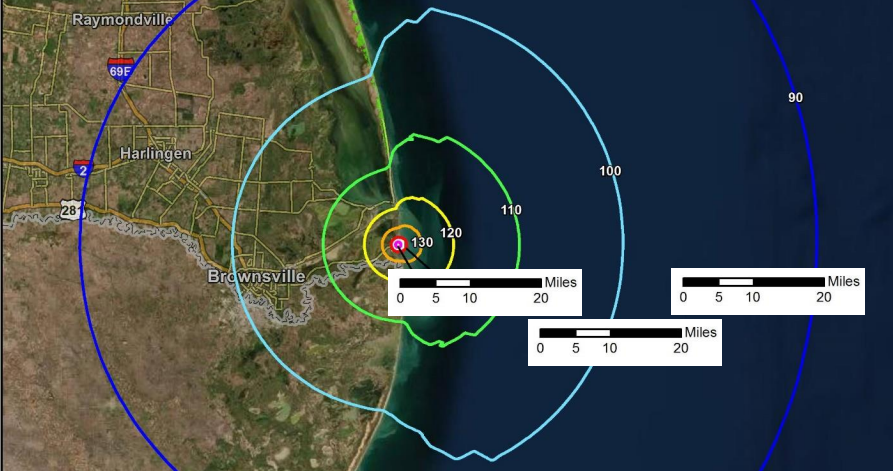
enter image description here

However, if we use the above image as a representative example, we can see that the 100 dB and 90 dB rings are about 27 miles apart. Zero dB is defined to be the threshold of human hearing.

enter image description here

If we assume that the 80, 70, etc. rings are spaced the same as the 100-90 rings, it would still be possible to hear the sound of the launch if you were out to sea at a distance of 300 miles, or around 482 km. But the spacing of the rings might continue to increase for a while longer, in which case you would be able to hear the launch from even further away.

Given what we know about Krakatoa, it would be interesting to learn whether it would be possible for someone stationed at Starbase's antipodal point (which is in the middle of the Indian Ocean) to hear the sound of the launch. I suspect that it would be possible to record the sound there - with a sensitive enough microphone.

Improve this answer
$\endgroup$
-4
$\begingroup$

We were in the Porcupine Mountains in Michigan on 5/17/24. We heard the SpaceX rocket launched from Florida for about 15 seconds. There was a storm front to the west and I believe the water also played a role. I have been to multiple rocket launches and this was definitely a rocket at the exact time of the Florida launch.

Improve this answer
$\endgroup$
2
  • 11
    $\begingroup$ Porcupine Mountains is 1,355 mi (2,182 km) from Cape Canaveral. The Starlink 6-59 launch headed East over the Atlantic, further increasing the distance. And within minutes it would be past the effective atmosphere and no longer producing sound. You may have heard coincidental thunder or a military jet aircraft but it was certainly not a Falcon 9 rocket. $\endgroup$
    – Steve Pemberton
    Commented May 20 at 12:22
  • 1
    $\begingroup$ I didn't factor the speed of sound. That only goes around 700 miles per hour. It must have been something else. Sure felt like the rocket launches I experienced at Vandenberg $\endgroup$
    – Jeffrey DeDecker
    Commented May 21 at 15:39

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.