Spiraling out from circular orbit to escape via low thrust, what is γ (gamma)?

Question

@MarkAdler's comment led me to ask Why would a slow spiral from a C3 of zero take about 2.4 times as much ΔV as an impulsive maneuver? which resulted in this tidy and efficient @MarkAdler answer which points to another thoughtful answer about slowly spiraling out from a circular orbit to escape in the limit of very weak prograde propulsion, which (at first counterintuitively) slows you down while raising your orbit.

Below that answer is yet another easter-egg-like comment gem.

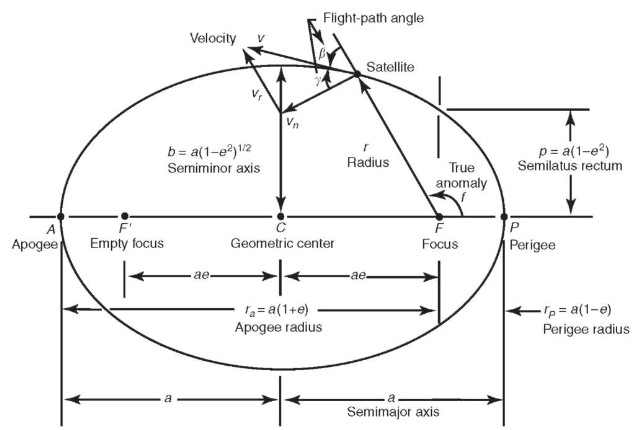
Always aligned with the velocity vector. That is the most efficient use of the thrust in order to increase the specific energy. The final γ is 31°^†.

Question: In this context, what is the angle γ? How is it defined?

^† The phenomenon is correct but 31° was a typo and should be about 39.2° instead.

Answer 1

Image illustrating the flight path angle, $\gamma$, as requested by uhoh's comment

Source

It is just the angle between the velocity vector and the tangential orbit component, $\vec{e}_{\theta}=[-\sin(\theta), \cos(\theta), 0]^T$, assuming the orbit plane is the $XY$ plane. The bounds for $\gamma(t)$$\in$$[-\pi/2, \pi/2]$, an the zeros happens at periapsis and apoapsis (or always if the orbit is circular).

I have seen people taking the flight path angle as the one subtended between $\vec{e}_r$ and $\vec{v}$ ($\beta$ in the image). However, in my opinion, it is quite confusing since it not coincide with the aircraft definition of flight path angle (using $\gamma$ does coincide).

In the Mark Adler's simulations, there appear to be two cases, one with high constant acceleration value to highlight the spiral behaviour where the final flight path angle seems to be 31º and one with a low value of the acceleration where the final path angle seems to be near 0º (at look), so it has clearly a dependance of the applied acceleration. More acceleration changes the orbit more faster to elliptic ones (and hence final flight path angle is higher, if termination does not occur at periapsis or apoapsis) and low acceleration seems to keep the orbit quasi-circular when raising (hence the final flight path angle is almost null).