tl;dr: The FAI is doing that because they were convinced by Johnathan McDowell's proposal, one that he first forwarded 25 years ago. The FAI's statement must refer to his recent paper on the topic. ✅
The paper ends:
6. Conclusion:
I have shown that for a typical satellite ballistic coefficient the effective Karman line is close to (within 10 km of) 80 km independent of solar and atmospheric conditions, rather than the currently popular 100 km value; and that historical orbital data for actual artificial satellites confirms that orbiting objects can survive multiple perigees at altitudes around 80–90 km. This altitude range is consistent with the highest physical boundary the atmosphere, i.e. the mesopause, and with the 50-mile ‘astronaut wings’ boundary suggested by the United States during the first years of the Space Age.
On the basis of these physical, technological and historical arguments, I therefore suggest that a value of 80 km is a more suitable choice to use as the canonical lower ‘edge of space’ in circumstances where such a dividing line between atmosphere and space is desired.
Background:
From the question Have spacecraft ever dipped below the Karman line and then safely continued spaceflight?:
The item in Science Alert's A Harvard Astrophysicist Says Outer Space Is Actually Closer Than We Think (see also Science; Outer space may have just gotten a bit closer) talks about the recent Acta Astronautica article by Jonathan McDowell The edge of space: Revisiting the Karman Line, and ends with the quip:
That doesn't mean we're going to see any commonly used definitions change soon, though: McDowell first proposed his 80-kilometre boundary line in 1994 - over 25 years ago. Perhaps we need a new term for it instead: the McDowell line.
I think the idea is that once something that's been in Earth orbit drops to the Karman line in circular orbit, it's "toast" in that it's fate is fairly well sealed and it has hours to perhaps a day at most before burning up.
Earlier, the article says:
So back to McDowell. He chose for his proposed boundary the 80-kilometre mark, just below the mesopause - the boundary between the lower mesosphere and the upper thermosphere, and the coldest point in the Earth's atmosphere.
And this is because of the satellites. McDowell analysed over 90 million points of orbital data from 43,000 satellites dating back to 1957, using archives maintained by the North American Air Defence Command.
Most of the satellites fly pretty high, but he identified 50 that flew below the 100-kilometre mark, down as low as the 80-kilometre mark, over two or more complete revolutions of Earth.
"Are you going to say [these satellites are] in space and then not in space every 2 hours?" he told Science. "That doesn't seem very helpful."
Analysis:
I've "bulletized" the block quote of the FAI statement in the question as follows:
These analyses combine data/modelling from a number of differing perspectives
- latitudinal variations during solar cycles
- theoretical lift coefficients for different size/configuration satellites ranging from cubesats to the International Space Station
- perigee/apogee elliptical analysis of actual satellite orbital lifetimes
Now let's look at McDowell's paper to see if those are addressed there.
1 latitudinal variations during solar cycles
From the McDowwell paper:
To understand the effect of these variations on the function z(k) I ran models for assorted times and geographical locations using a code which implements the NRL MSISE-00 atmospheric model [41]. Atmospheres were calculated at 10 day intervals from Jan 1960 to Jan 2020 to fully sample several solar cycles. At each selected day atmospheres were calcualted for 0, 6, 12 and 18 h GMT at four latitudes (80 S, 0 N, 45 N and 80 N) and four longitudes (0, 90, 180, 270 E). For each epoch, actual or (for future dates) predicted solar activity levels from the Celestrak space weather archive [42] [43], were used.
✅ Check!
2 theoretical lift coefficients for different size/configuration satellites ranging from cubesats to the International Space Station
Sections 5.1 through 5.3 are too long and in-depth to try to generate a sound-bite here. The paper is not paywalled and can be reviewed by the reader. However I'll summarize that instead of sticking explicitly to the drag and lift coefficients $C_D, C_L$ McDowell abstracts the problem to something he defines here as the Karman parameter
$$k(B, r, \rho) = \log_{10}(R) = \log_{10}\left( \frac{2}{B r\rho} \right)$$
and goes on to find a *fiducial Karman parameter based on a $B_0$ of 0.01 m^2/kg, where $B$ is not the well known ballistic coefficient $\beta$, but rather its reciprocal $B=C_D A/m$.
He provides values for $B$ from a wide variety of extreme examples, from the ISS, to balloons, to Dove cubesats, to a 10-m-diameter solid sphere of pure osmium!
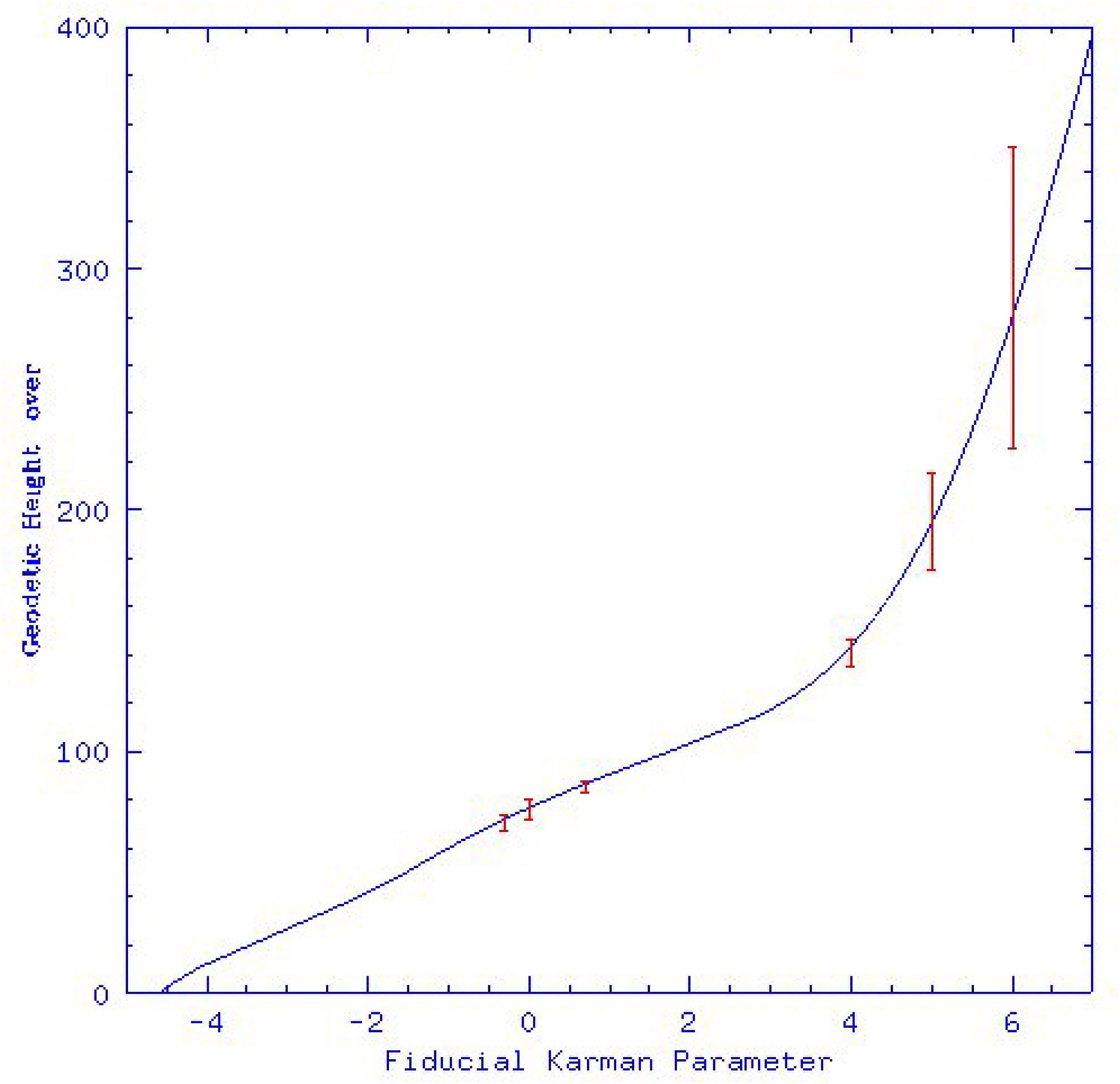
above: Fig. 2. Fiducial Karman parameter versus geodetic altitude for US Standard Atmosphere 1976. The vertical error bars summarize the results of Fig. 3, Fig. 4, indicating the range of variation in the altitude for a given value of found in runs of the NRL atmosphere model for different dates, latitudes and longitudes.
Note that based on this analysis, the logarithm of the fiducial Karman parameter reaches zero at an altitude of 80 kilometers.
✅ Check!
3 perigee/apogee elliptical analysis of actual satellite orbital lifetimes
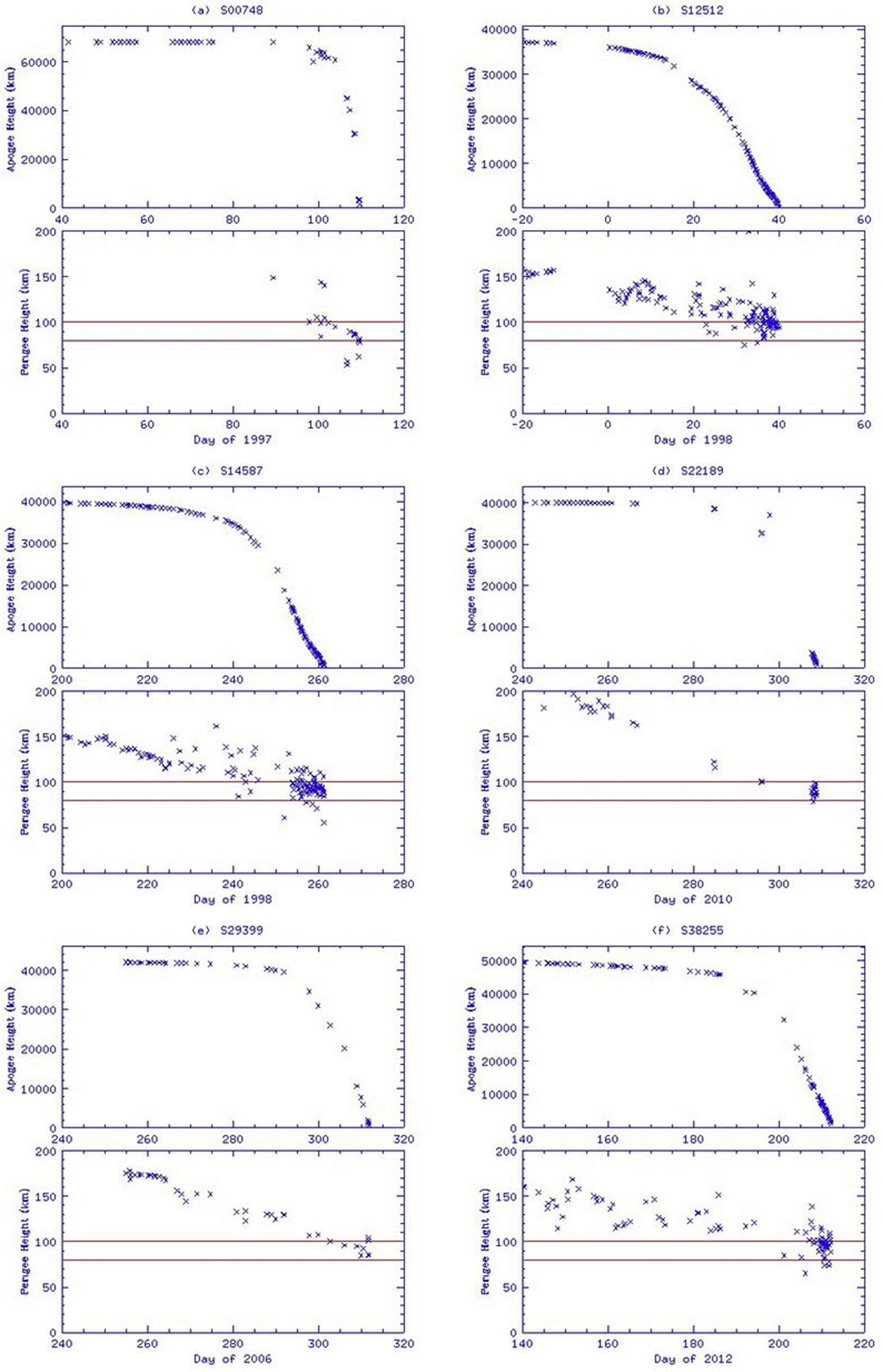
above: Fig. 1. Geodetic height of apogee and perigee versus time for the decay of selected elliptical orbit satellites. Horizontal lines at 80 and 100 km are superimposed on the perigee plots. Despite noisy fits, these satellites appear to have survived multiple perigee passages below 100 km. (a) Satellite 748 (1964-006 B, Elektron 2, 2D No. 2); (b) Satellite 12512 (1981-30 A, Molniya-3 No. 30); (c) Satellite 14587 (1983-126 A, Kosmos-1518, Oko 6022); (d) Satellite 22189 (1992-069 A, Kosmos-2217, Oko 6059); (e) Satellite 29399 (2006-038 B, Chang Zheng 3 A Y10 third stage rocket); (f) Satellite 38255 (2012-019 B, Centaur AV-031 rocket).
✅ Check!

