16
$\begingroup$

I understand with current technology we can't just fly a straight line out of the solar system but which way out would need the least fuel?

Currently to navigate the solar system it is a dance around the planets using sling shots, gravity assist and such.

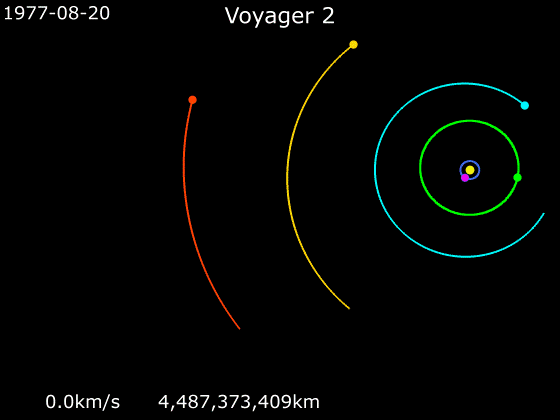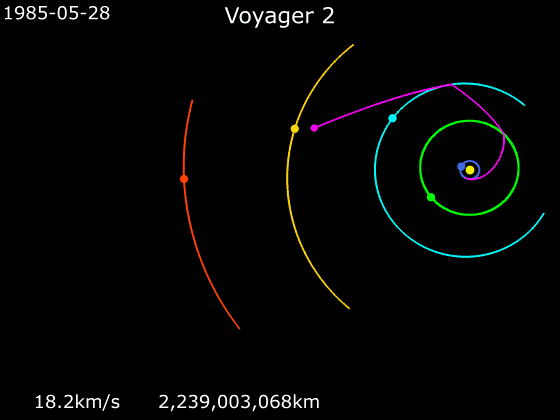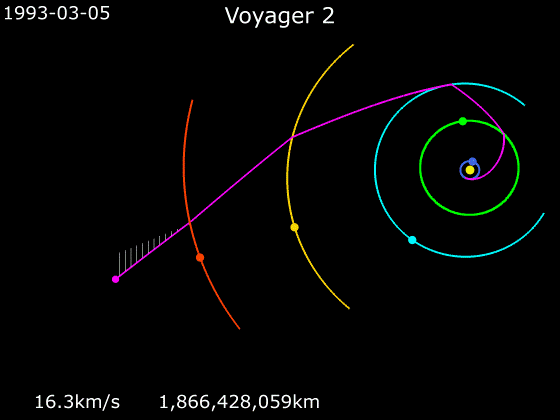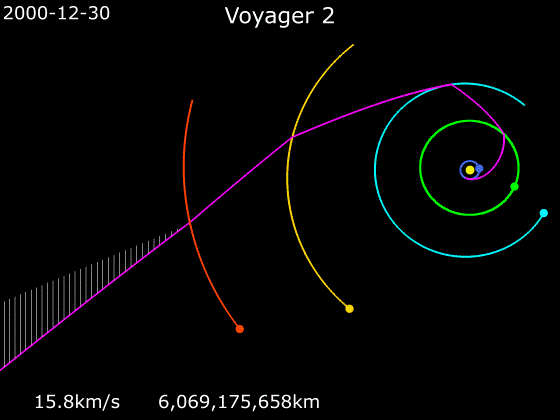
The Ground Tour trajectory of Voyager 2 "used" the first three of the four large planets Jupiter, Saturn, Uranus, and Neptune, but this was optimized for time, and it ended up with far more than heliocentric escape velocity.

Suppose instead the goal of the exercise were to barely achieve heliocentric escape velocity using the minimum fuel or delta-v, starting from LEO, with a lot more flexibility on time, say roughly 100 years from launch to achieving escape velocity (C3=0). Assume you can start at optimum configuration of the planets within their orbits.

What would that trajectory look like? Would it still use all four of these planets, or could you get by with fewer? Would it make sense to look inward, using the four rocky planets instead?

As a side question, would having even more planets present on the way out always help?

Voyager 2 Grand Tour

above: Voyager 2 Grand Tour. Source

below: Voyager 2 Grand Tour Heliocentric velocity. Source

Voyager 2 Grand Tour Heliocentric velocity

Improve this question
$\endgroup$
9
  • 4
    $\begingroup$ Where would you like to go outside the solar system? $\endgroup$
    – Thorbjørn Ravn Andersen
    Commented Feb 27, 2019 at 23:14
  • 1
    $\begingroup$ direction. "Way" implies a 'way of means' IMO. Which would use tidel influences on a machine of planetary scale. Fuel consumption: zero. $\endgroup$
    – Mazura
    Commented Feb 27, 2019 at 23:59
  • 10
    $\begingroup$ This is the second question in a few days about escaping the solar system. I'm wondering if there's something specific I need to be worried about... $\endgroup$
    – Richard
    Commented Feb 28, 2019 at 0:40
  • 3
    $\begingroup$ @Richard the galaxy is on Orion's belt $\endgroup$
    – uhoh
    Commented Feb 28, 2019 at 2:38
  • 1
    $\begingroup$ I still don't get what was wrong with the gif, other than it doesn't show the sun bobbing up and down in the plane of the Milky Way. $\endgroup$
    – Mazura
    Commented Mar 2, 2019 at 6:12

4 Answers 4

Reset to default
24
$\begingroup$

The most fuel efficient way to leave the solar system at present, is to launch into a trajectory that (like that used for Gallileo) may well involve one or several gravity assists from Earth or Venus, but which eventually gets you to Jupiter. If you can get to Jupiter you can almost certainly do so in such a way as get a slingshot into a solar escape trajectory. Encounters with other planets after that are just "icing on the cake" (you get into a faster escape trajectory) and like all gravitational slingshots there is a problem of diminishing returns. The faster you are going, the less good they do you. Anyway the problem as stated is basically the same one as getting to Jupiter.

The Trident mission proposal illustrates a trajectory of this kind. The initial launch is to a Venus transfer orbit. After that, it uses gravity assists at Venus, Earth (twice) and Jupiter to get to Neptune with no significant further fuel consumption and at a speed which will certainly take it out of the solar system.

A related question is how to get leave the solar system going as fast as possible for a given supply of fuel (equivalently a given total delta-V). You still start by going to Jupiter. Then you use the gravity of Jupiter to drop you into as energetic an orbit as possible, passing as close to the Sun as your systems can survive. Once there you burn all your remaining fuel and then you coast. You might gain a little from encounters with Jupiter and/or Saturn on the way out, but you are moving so fast it doesn't really matter.

The Thousand AU study reckoned you could achieve exit from the inner solar system at a velocity of perhaps 10-15 AU/year using this technique with a large current launcher. An additional advantage is that you can exit in any direction you like. more or less.

Improve this answer
$\endgroup$
14
  • 1
    $\begingroup$ @Muze check out the parker solar probe's trajectory, except at the closest point of approach instead of doing science you'd do a burn. I'd love to see the actual numbers though, given a particular craft and a particular perigee. $\endgroup$
    – Magic Octopus Urn
    Commented Feb 27, 2019 at 17:36
  • 1
    $\begingroup$ If for some reason you don't want to do any gravity well maneuvers at all then you want to launch tangentially to Earth's orbit to get maximum benefit from Earth's orbital velocity. You'd want to launch at midnight so that Earth's rotational velocity added in as well. But this is still much worse than going via a close solar encounter $\endgroup$
    – Steve Linton
    Commented Feb 27, 2019 at 17:40
  • 3
    $\begingroup$ @Muze What is the fastest way out is almost certainly not the most fuel efficient. Venus is the place we can get for the least energy and once you can reach another world you can escape by playing billiards--but that's a slow process unless everything is neatly lined up for you like with the Voyager probes. $\endgroup$
    – Loren Pechtel
    Commented Feb 27, 2019 at 22:13
  • 1
    $\begingroup$ How about the Voyager/Grand Tour option, where your gravity assist from Jupiter is followed by ones from Saturn, Uranus, and Neptune? AFAIK, the Voyagers didn't use fuel (other than a small amount for mid-course corrections) once they had boosted from Earth orbit. Getting to Jupiter via Venus/Earth assists might make it even more fuel-efficient. $\endgroup$
    – jamesqf
    Commented Feb 28, 2019 at 2:09
  • 9
    $\begingroup$ @Muze, If you think that the most efficient path would be a "straight line," then you are pretty far from understanding how objects move in the Solar system. Far enough that the kind of answers that you can find on a web site like this probably are going to be less than helpful. You need some book learning or some classroom learning. Good luck! $\endgroup$
    – Solomon Slow
    Commented Feb 28, 2019 at 3:00
11
$\begingroup$

If you want to avoid gravity assists, the most fuel-efficient way out of the Solar System is to launch due East from from a launch site in the Ecuadorean Andes, sometime before local midnight on a January 3 when there's a new moon. This gives you the maximum possible benefit from the Earth's movement, leaving only about 12,000 m/s of delta-V needed in excess of Earth escape velocity.

(Rough estimate: a Saturn V could barely put New Horizons directly into a solar escape trajectory. This is why we use gravity assists instead.)

Improve this answer
$\endgroup$
6
  • $\begingroup$ This is intriguing! I've just asked Launching east from a mountain on the equator at midnight during a new moon; ranking of each contribution? $\endgroup$
    – uhoh
    Commented Feb 28, 2019 at 2:32
  • $\begingroup$ Not quite work a downvote since a simple edit will fix this answer. It would be better to launch on about the third of January (Earth perihelion) than the fourth of July (Earth aphelion). The advantage from a higher orbital velocity outweighs the disadvantage from being deeper in the Sun's gravitational well thanks to the Oberth effect. $\endgroup$
    – David Hammen
    Commented Feb 28, 2019 at 17:02
  • $\begingroup$ @DavidHammen, you sure about that? At aphelion, I get a solar escape velocity of 41174 m/s and an orbital velocity of 29290 m/s for a net required velocity of 11884 m/s. At perihelion, I get a solar escape velocity of 42480 m/s and an orbital velocity of 30290 m/s, for a net required velocity of 12118 m/s. The reduced escape velocity from a higher orbit slightly outweighs the loss in Earth orbital velocity. $\endgroup$
    – Mark
    Commented Feb 28, 2019 at 20:36
  • 2
    $\begingroup$ @Mark - Quite positive. I agree with all your numbers to within a few meters per second except the escape velocity at aphelion, which is low by about 600 m/s. Moreover, look at the vis viva equation, $v^2 = \mu\left(\frac2r-\frac1a\right)$. Since escape velocity $v_e$ is ${v_e}^2 = \frac{2\mu}r$, another way to write the vis viva equation is ${v_e}^2-v^2 = \frac\mu a$. The difference between escape velocity and orbital velocity is thus $v_e-v = \frac{\mu/a}{v_e+v}$. Periapsis maximizes both $v_e$ and $v$ and therefore minimizes the difference between escape velocity and orbital velocity. $\endgroup$
    – David Hammen
    Commented Feb 28, 2019 at 23:39
  • $\begingroup$ Now, how to escape Sol's gravity altogether? $\endgroup$
    – AndrewMaxwellRockets
    Commented Mar 1, 2019 at 1:57
0
$\begingroup$

Launching from the west side of Earth and taking a slingshot course by the sun for a turn of about 90*, then doing another sling past Jupiter(burning all your fuel) would, I think, be the most efficient way to do this. Ion engines and mirrors would be the most efficient propulsion method as well, however I would recommend using common LOXY engines to get into orbit, and then the ion engines to break orbit and head towards the sun. It would work, if you were to launch your rocket today, along the orbital path of the planets...

However, another way to do it(again with the help of the sun) would be to go into LEO, and then set your periapsis to the 'night side' of Earth. Then, at periapsis, have your engines at full blast until the other side of your orbit goes to the opposite side of Sol, and then coast till you reach the bottom, and, again, go full blast. by the time you are done accelerating, your apoapsis should be well out of the solar system. sol acceleration

There is no way to go "straight" out of the solar system without being accelerated and decelerated. Gravitational forces will always be acting upon you, and until you get to the point where the nearest extrasolar body's gravity is stronger than the sun's, you won't be "out" of the solar system.

Improve this answer
$\endgroup$
12
  • 3
    $\begingroup$ Light pressure at Earth's distance from the Sun is about $6\mu Pa$. An alumnium sail 50 nm thick (much thinner and it would be transparent) has areal density about $1.3 \times 10^{-4} kg/m^2$ so with no payload would accelerate at $4\times 10^{-2} m/s^2$ (abotut 4 milli gravities). By the time it reached the orbit of Mars (ignoring the Sun's gravity) it would be moving at something in the ballpark of $100 km/s$. So yes, a very thin solar sail with no allowance for rigging or payload could escape the solar system. $\endgroup$
    – Steve Linton
    Commented Feb 27, 2019 at 18:26
  • 7
    $\begingroup$ @SteveLinton, the nice thing about solar sails is that both gravity and light pressure fall off as the inverse square of distance. If you've got a sail that provides acceleration at one distance from the Sun, it'll provide acceleration at all distances from the Sun. $\endgroup$
    – Mark
    Commented Feb 27, 2019 at 23:47
  • 1
    $\begingroup$ @sampathsris, until other stars start interfering with it. Most of the force on a solar sail comes from sunlight, not the solar wind, and that doesn't stop at the heliopause. $\endgroup$
    – Mark
    Commented Feb 28, 2019 at 6:20
  • 3
    $\begingroup$ If I'm reading this correctly this is definitely not the most fuel efficient way to leave the solar system. Going to the sun with an impulsive maneuver straight from earth is significantly more expensive than simply going straight to an escape trajectory. From LEO, it takes ~7 km/s of delta-V to get on an escape trajectory out of the solar system. To end up on a trajectory that goes near the sun takes on the order of ~20 km/s. $\endgroup$
    – Kyle
    Commented Feb 28, 2019 at 9:39
  • 3
    $\begingroup$ This should not be the accepted answer. As @Kyle noted, without gravity assists, it's very harder to get close to the Sun than it is to simply escape the solar system. Gravity assists change this dynamic. Steve Linton's answer is much better in this regard. $\endgroup$
    – David Hammen
    Commented Feb 28, 2019 at 11:36
0
$\begingroup$

Check out Could a “solar gravitational synchrotron ” use solar thermal rockets to "powerslide" spacecraft out of the solar system?

This strategy uses a solar thermal rocket and ISRU water to accelerate spacecraft to solar escape velocity. There are no timing limitations from gravity assist rendezvous. Any departure direction in the ecliptic is allowed.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.