I have read (even on this site), that exhaust gas velocity is (normally?) sonic (exactly Mach 1) through the choke point of a De Laval, or convergent-divergent, nozzle:
At the "throat", where the cross-sectional area is at its minimum, the gas velocity locally becomes sonic (Mach number = 1.0), a condition called choked flow.
Why is that optimal/required?
What would be the effect of exhaust gas being supersonic through the throat?
And what about subsonic (eg Mach 0.9)?
Uwe's comment on the question is spot on. The characteristics of the flow through the nozzle depend critically on the pressure ratio - the two pressures being the pressure at the entrance to and exit from the nozzle.
Above the critical pressure ratio flow through the nozzle is subsonic and it is not choked at the throat. Below the critical pressure ratio, the flow chokes at the throat, reaching a maximum.
This figure from The Dynamics and Thermodynamics of Compressible Fluid Flow by Shapiro shows the variation in properties based on the pressure ratio.
The most interesting feature of Fig. 4.3 is the maximum in the curve of flow per unit area...The pressure ratio p/p0 where the flow per unit area is a maximum is called the critical pressure ratio and has a value for all real gasses and vapors of approximately one-half.
Pressure ratios greater than the critical correspond to subsonic flow and pressure ratios less than the critical correspond to supersonic flow.
(p. 76)
The next graph from p. 87 shows the same parameters plotted against Mach number. You can see the knee in the area ratio curve at the critical pressure ratio well here. This corresponds to the throat of the nozzle.
There's a good discussion of the phenomenon here
If you lower the back pressure enough you come to a place where the flow rate suddenly stops increasing all together and it doesn't matter how much lower you make the back pressure (even if you make it a vacuum) you can't get any more mass flow out of the nozzle. We say that the nozzle has become 'choked'. You could delay this behavior by making the nozzle throat bigger ... but eventually the same thing would happen. The nozzle will become choked even if you eliminated the throat altogether and just had a converging nozzle.
The reason for this behavior has to do with the way the flows behave at Mach 1, i.e. when the flow speed reaches the speed of sound. In a steady internal flow (like a nozzle) the Mach number can only reach 1 at a minimum in the cross-sectional area. When the nozzle isn't choked, the flow through it is entirely subsonic and, if you lower the back pressure a little, the flow goes faster and the flow rate increases. As you lower the back pressure further the flow speed at the throat eventually reaches the speed of sound (Mach 1). Any further lowering of the back pressure can't accelerate the flow through the nozzle any more, because that would entail moving the point where M=1 away from the throat where the area is a minimum, and so the flow gets stuck. The flow pattern downstream of the nozzle (in the diverging section and jet) can still change if you lower the back pressure further, but the mass flow rate is now fixed because the flow in the throat (and for that matter in the entire converging section) is now fixed too.
The changes in the flow pattern after the nozzle has become choked are not very important in our thought experiment because they don't change the mass flow rate.
The answer is much simply presented in the book by John D Anderson - Modern Compressible Flow with historical perspectives. Refer Chapter 5.
\begin{equation} \rho = \mbox{density} \end{equation} a = speed of sound, Ma = Mach number, u = speed of fluid, A = area
Area velocity relationship can be deduced from the continuity relationship - \begin{equation} \frac{d\rho}{\rho} + \frac{du}{u} + \frac{dA}{A} = 0 \end{equation}
Watch now ... \begin{equation} \frac{dA}{A} = - \{\frac{d\rho}{\rho} + \frac{du}{u}\} \end{equation}
From momentum conservation relationships between two points, we can write \begin{equation} dp = -\rho udu \\ \implies \frac{dp}{\rho} = \frac{dp}{d\rho}\frac{d\rho}{\rho} = -udu \end{equation}
and
\begin{equation} \frac{dp}{d\rho} = a^2 \end{equation}
Therefore,
\begin{equation} \frac{d\rho}{\rho} = -\frac{udu}{a^2} = -Ma^2 \frac{du}{u} \end{equation}
From these relations, we can write
\begin{equation} \frac{dA}{A} = (Ma^2 - 1)\frac{du}{u} \end{equation}
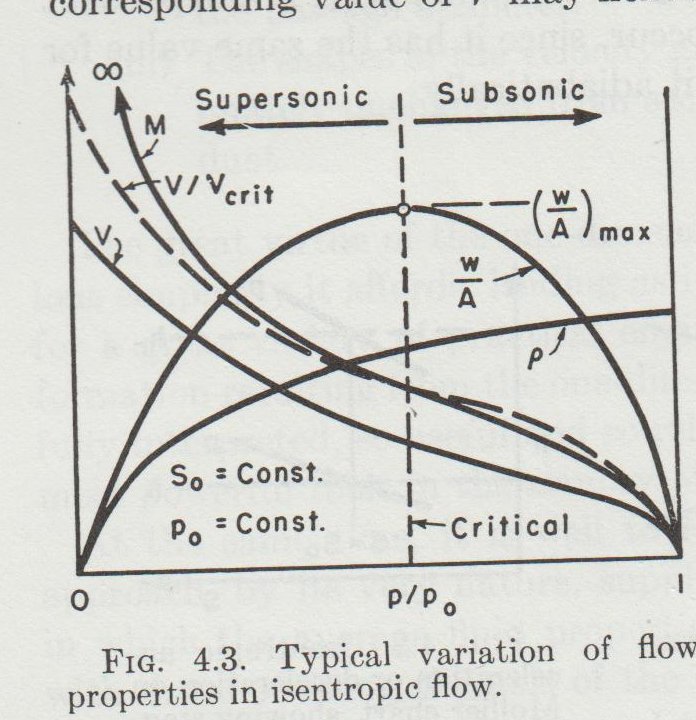
Now check for Ma = 1, you will get
\begin{equation} \frac{dA}{A} = 0 \end{equation}
This means the area function is either at maxima or minima. The practical solution is that this is minimum. This minimum area is called throat of the nozzle.
