4
$\begingroup$

Phobos
Credit: NASA / JPL-Caltech / University of Arizona

Phobos has a nearly circular orbit almost parallel with the equator of Mars.
It orbits the planet in only 7h.39 min. at a height of 6000 km above the surface with a speed of about 2.1 km/sec.

I suspect that when launching a chunk from Phobos into the direction of any martian pole ( so perpendicular to the orbital plane ) it would be difficult to reach it because of the orbital speed of this moon.

Question: Could the launching direction and speed be adapted in such a way that the chunk could reach any of the poles ?

I would like to have an answer that shows the necessary equations and calculations for verification.

Improve this question
$\endgroup$
4
  • $\begingroup$ What does "launching... from Phobos into the direction of any martian pole" mean? For example, does it mean pointing a rocket at the pole and pushing the launch button? Or does it mean launching at a backwards angle zeroing out Phobos' forward orbital velocity which it seems the first answer might assume. (What would happen if you fired a gun on a train moving as fast as a bullet? "If you shoot the bullet off the back of the train... (r)elative to the ground, the bullet will not be moving at all, and it will drop straight to the ground.") $\endgroup$
    – uhoh
    Commented Jul 8, 2021 at 22:12
  • $\begingroup$ @uhoh You can only give the chunk on Phobos one initial push. When you launch it into the direction of the north pole, that direction is perpendicular to the orbit parallel to the equator, isn't it ? But I'm amazed right now that it's so simple to get there inside a vertical plane through that pole. $\endgroup$
    – Cornelis
    Commented Jul 9, 2021 at 7:08
  • 1
    $\begingroup$ Phobos is moving at roughly 2.1 km/sec. If you aim directly at the pole you will never hit the pole because along with whatever delta-v your push is, in the frame centered on the planet it will also have that sideways velocity because it was shot moving along with Phobos. To enter into a polar orbit or planet that contains the poles, you have to shoot backwards somewhat. It seems that the answer explains the same thing, you aim mostly backwards (retrograde) and somewhat polar (zenith). So you can't launch in the direction of the pole from the moving moon if you want to hit the pole. $\endgroup$
    – uhoh
    Commented Jul 9, 2021 at 7:57
  • 1
    $\begingroup$ That's a clear explanation ! I didn't have the time last night to read the answer thoroughly, which I will do right now. Especially I'm curious about the cheaper solution. Of course the Coriolis force has nothing to do with this at all, so I'll remove that part. $\endgroup$
    – Cornelis
    Commented Jul 9, 2021 at 8:29

1 Answer 1

Reset to default
9
$\begingroup$

The direct trajectory is an ellipse intersecting the pole:

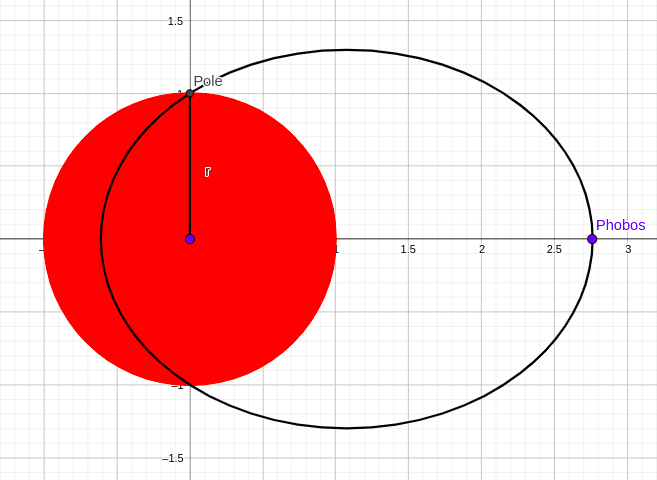
orbital problem

We do not have the semi-major axis of this trajectory, however, so we must obtain that first.

For that, we can use one of my favourites, the equation for the orbital radius in terms of the periapsis $P$, apoapsis $A$ and the distance along the apsis line $d$

$$r = P + d\frac{A - P}{A +P}$$

For our case, $d = P$, so we have:

$$r = P + P\frac{A - P}{A +P}$$

Solving for the quantity we want, we have:

$$P = \frac{Ar}{2A - r} = 2070 km$$

We then have all the parameters we need to use the vis-viva equation

$$v = \sqrt{\mu\left(\frac{2}{r} - \frac{2}{A + P}\right)}$$

We want the velocity when at Phobos, the apoapsis of that trajectory, so

$$v_A = \sqrt{\mu\left(\frac{2}{A} - \frac{2}{A + P}\right)} = 1290 m/s$$

Now, we must not forget that this is a problem in 3 dimensions:

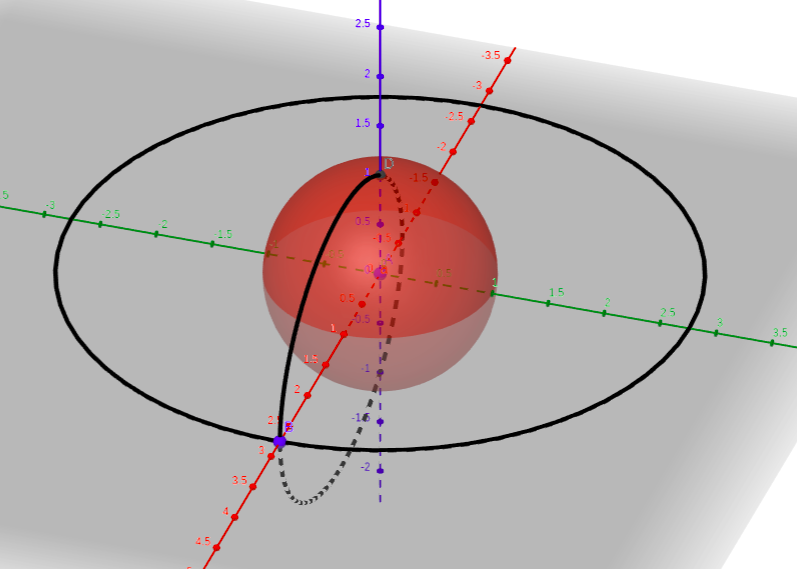
3D trajectory

Which means the apoapsis velocity of $1290 ms/$ and the orbital velocity of Phobos are orthogonal:

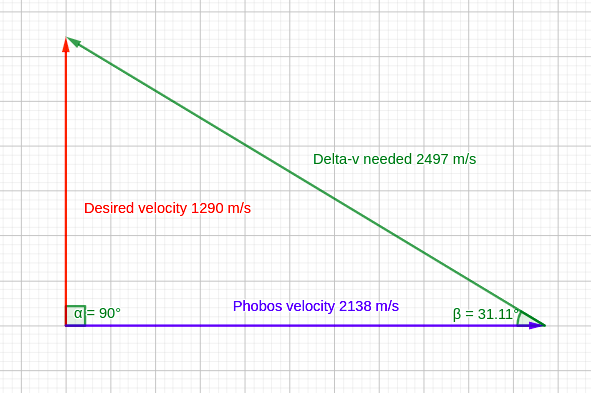
orthogonal velocities

The delta-v cost of the burn is therefore 2500 m/s, and is 30 degrees zenith from retrograde.

Cheaper and slower solution:

Launch prograde instead, up to almost escape velocity from Mars (delta-v cost: 886 m/s, $\sqrt{2} - 1$ times the orbital velocity of Phobos).

Then, at great distance, where the orbital velocity is just a few m/s, change the inclination and periapsis at almost no cost and fall back towards the Martian poles with a more violent impact.

Improve this answer
$\endgroup$
8
  • 3
    $\begingroup$ @Cornelis For the direct trajectory, no further propulsion is needed. Coriolis force only exists in rotating frames of reference, and since I'm using an inertial frame here, only gravity has to be considered. $\endgroup$
    – SE - stop firing the good guys
    Commented Jul 8, 2021 at 18:25
  • $\begingroup$ Just to understand your favourite equation for the orbital radius, where can I find the derivation of it, somewhere on Wiki ? $\endgroup$
    – Cornelis
    Commented Jul 9, 2021 at 9:44
  • $\begingroup$ @Cornelis I do not have a derivation of hand, but it it can be derived from the following property of ellipses: the sum of the distances to the foci is constant. $\endgroup$
    – SE - stop firing the good guys
    Commented Jul 9, 2021 at 10:38
  • $\begingroup$ To be precise, P(periapsis) and A(apoapsis) are points, no distances.. $\endgroup$
    – Cornelis
    Commented Jul 9, 2021 at 13:28
  • 1
    $\begingroup$ d is the distance from the periapsis to the projected point on the apsis line: i.sstatic.net/AaN5Q.png $\endgroup$
    – SE - stop firing the good guys
    Commented Jul 9, 2021 at 15:44

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.