TL;DR
Without SRBs, by maintaining the real stack's thrust to weight ratio at ignition, you run out of fuel at about 167 seconds with (if we maintain the flight profile of the real stack) a velocity around 1.4 km/s and an altitude of around 70 km.
Without SRBs, with an initial thrust to weight ratio of 1, you run out of fuel at about 289 seconds with (if we maintain the flight profile of the real stack) a velocity around 2.4 km/s and an altitude of around 100 km.
To attain a stable, low Earth orbit, you need around 7.4 km/s at approximately 110 km altitude.
A few data points
- The Orbiter (specifically OV-105) had an empty weight of 68,585 kg (68.6 Mg) or 110,000 kg (110 Mg) at liftoff
- The Super Lightweight External Tank had an empty weight of 26,500 kg (26.5 Mg) or 756,000 kg (756 Mg) when fully fueled
- The Orbiter plus the Super Lightweight External Tank thus have a combined empty weight of 95.1 Mg.
- The Orbiter (again OV-105) three main engines provided 1,752 kN thrust each (when running at 104% at sea level) for a total of 5,255 kN or 536 Mgf on SSMEs only
- The two solid rocket boosters (SRBs) massed 571 Mg each at liftoff, and contributed 12,500 kN or 1,274 Mgf of thrust each
Common assumptions
Given that the SSMEs burned through 629,340 kg of LOX and 106,261 kg of LH2 in a 480 seconds normal ascent burn, the burn rate is about 1.3 Mg/second LOX and 0.22 Mg/second LH2. We also see that the LOX to LH2 mass flow ratio is about 5.92:1 as $\frac{629\,340}{106\,261} \approx \frac{5.92}{1}$.
For simplicity, I'm not allowing for any payload whatsoever. In this scenario, if you want to carry a payload, doing so will necessarily reduce the amount of fuel you can bring, because you are basically transferring liftoff mass from fuel (which you need to get off the ground) to, as far as propulsion is concerned, dead weight.
Realistic case: thrust to weight ratio at ignition same as a normal Space Shuttle including SRBs
For a normal Space Shuttle, including external tank and SRBs, the total thrust provided by all five engines (three main engines plus two SRBs) is $3 \times 1\,752 + 2 \times 12\,500 = 30\,255$ kN or about 3,085 Mgf at liftoff. At the same time, the total mass of the fully equipped spacecraft is $110 + 756 + 2 \times 571 = 2\,008$ Mg. This gives a thrust to weight ratio (TWR) of $\frac{3\,085}{2\,008} \approx 1.536$ at the moment of ignition.
Because absent the SRBs we have 536 Mgf of thrust, we can allow for $\frac{536}{1.536} \approx 349$ Mg of gross liftoff weight while maintaining the thrust to weight ratio. Subtracting the empty weight of the Orbiter and the SLET leaves about 254 Mg that we can use for fuel.
Keeping the 5.92:1 LOX/LH2 ratio, we can load up about 36.7 Mg of LH2 and 217 Mg of LOX.
By burning 1.3 Mg/second LOX and 0.22 Mg/second LH2, this fuel load lasts $\frac{217}{1.3} \approx \frac{36.7}{0.22} \approx 167$ seconds. Consequently, we run out of gas in about 167 seconds.
Absolute best theoretical case: thrust to weight ratio = 1 at ignition
Since the orbiter plus external tank with no payload whatsoever weighs 95 Mg, and you have 536 Mgf of thrust, this leaves you with about 440 Mg that you can use for fuel and still get the spacecraft to move at all.
Keeping the LOX/LH2 mass flow ratio the same, in 440 Mg we can fill the ET with some 376 Mg LOX and 63.6 Mg LH2 ($376 \approx 63.6 \times 5.92$). Assuming that the mass flow rate is unchanged with this lower initial mass, this fuel load will be depleted after 289 seconds, give or take a fraction of a second or so.
Note that this configuration will require very different launch facilities to keep the spacecraft from being destroyed by reflected energy before it has time to climb sufficiently that this is not a major problem. But since the Space Shuttle absent the SRBs is already a quite different spacecraft from what we had, I think it's safe to say that we can also adjust the launch facilities to accomodate the different spacecraft design.
Where does that leave us?
I couldn't find any nice official NASA graph for altitude versus time for the Space Shuttle (if anyone knows of one, please comment!), but velocity versus time was easier.
This xkcd forums post by davidstarlingm references the NASA STS-30 press kit, which among a few other notable points in the trajectory puts "Negative Return" at 238 seconds with a velocity of 6,915 ft/s which is a hair under 2,108 m/s and an altitude of about 319,000 feet or 97 km. While I doubt the real curve is this nice (taking into account for example the engine dethrottling late in the ascent to maintain a maximum 3 G acceleration should result in a more S-shaped curve), looking at the plot of the velocity versus time data and the function describing the data points available:
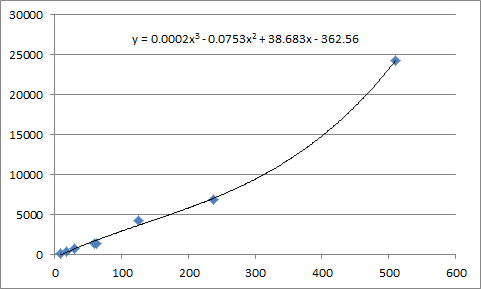
puts the Space Shuttle velocity around the 290 second mark at somewhere around 8,000 ft/s or 2,400 m/s. That's the theoretical best case, where we started out with a TWR = 1. As a quick approximation, we actually got to space today in terms of altitude; the Kármán line is at 100 km altitude, and we at least passed 97 km altitude some time before our engines shut down due to fuel exhaustion.
In the more realistic case maintaining the original stack's TWR = 1.536 at T-0, at 167 seconds the spacecraft's velocity is around 5,000 ft/s or 1,500 m/s. At the same time you are about halfway between the real spacecraft's SRB staging at 125 seconds (153,405 ft altitude in the case of STS-30) and negative return at 238 seconds (STS-30: 319,008 ft altitude), so let's split the difference and call it 236,200 ft or 72 km. In this case, we are nowhere near space.
Compare these to the real STS-30 trajectory, which was attained with main engines cutoff (MECO) at 511 seconds (so actually a somewhat longer burn) and 24,286 ft/s (7.4 km/s) velocity at about 362,000 ft or 110 km altitude.
No matter how you slice it, from 1.5 km/s or 2.4 km/s to 7.4 km/s is quite a long way to go, and especially in the case of TWR = 1.536, we also still have a fair distance to climb.
As a consequence, at these points the Space Shuttle Orbiter is an unguided, unpowered ballistic projectile on its way back toward the ground.
