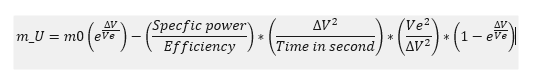This is my first answer, if this won't be clear tell me and I'll write it better. I've seen that equation when treating electric propulsion, I'll write the derivation here.
You can write your payload mass as the initial mass of your spacecraft, minus the mass of the electric power generator $m_s$ and the propellant mass $m_p$, so you can write the payload fraction as
$$\frac{m_u}{m_0}=1-\frac{m_s}{m_0}-\frac{m_p}{m_0}$$
The propellant mass is the only one ejected from the spacecraft, so it's the difference between initial and final mission's mass: $m_p=m_0-m_f$. Put that in the payload fraction and you get
$$\frac{m_u}{m_0}=\frac{m_f}{m_0}-\frac{m_s}{m_0}$$
You can think of the electrical generator mass being proportional to the generated power $P_E$ through a constant specific power $\alpha$, so $m_s=\alpha P_E$. The electrical power dictates a variation in kinetic energy of the spacecraft through the global efficiency of the propulsor $\eta$, so $\eta P_E = \frac{1}{2}\dot{m}_pc$, where $c$ is the exhaust speed. Since the mass flow is related to the thrust $T$ by $\dot{m}_pc=T$ you can write $m_s$ as:
$$m_s = \frac{\alpha}{\eta}\frac{Tc}{2}$$
We can now write the "generator mass fraction" $\frac{m_s}{m_0}$ by using the definition of mass flow $\dot{m}_p = \frac{m_p}{\Delta t}$, where $\Delta t$ is the time span of thrust and the rocket equation $\frac{m_p}{m_0}=(1-e^{-\frac{\Delta V}{c}})$:
$$\frac{m_s}{m_0}=\frac{\alpha}{\eta}\frac{T}{m_0}\frac{c}{2}$$
$$\frac{m_s}{m_0}=(1-e^{\frac{\Delta V}{c}})\frac{\alpha}{\eta}\frac{c^2}{2\Delta t}$$
Looking again at the payload fraction $\frac{m_u}{m_0}$ you can write the final to initial mass fraction with the rocket equation as $\frac{m_f}{m_0}=e^{-\frac{\Delta V}{c}}$, substitute the $\frac{m_s}{m_0}$ calculated before, and multiply and divide everything by $\Delta V^2$ to get:
$$\frac{m_u}{m_0}=e^{-\frac{\Delta V}{c}}-\frac{\alpha}{\eta}(\frac{c}{\Delta V})^2\frac{(\Delta V)^2}{2\Delta t}(1-e^{-\frac{\Delta V}{c}})$$