I have a simulation that describes a vehicle traveling at very high speeds (near or even above orbital) up out of the atmosphere and into space. I'd like to chart the rate of heating ($\dot{q}_{conv}$ and $\dot{q}_{rad}$) of the vehicle's nose cone versus time. Input parameters that I would like to provide are the atmospheric density, atmospheric temperature, atmospheric composition (that is, not necessarily Earth's atmosphere), nose cone angle, and nose tip radius, and airspeed. (If I forgot anything important, feel free to mention that in your answer.)
The equations should work for airspeeds in the 1 to 15 km/s range.
I'm fine with assuming the shape below, but if it's more convenient to provide an answer for a better shape that's fine too.
(from nose cone design)
I'm hoping that there are some formulas that I can use that resemble these
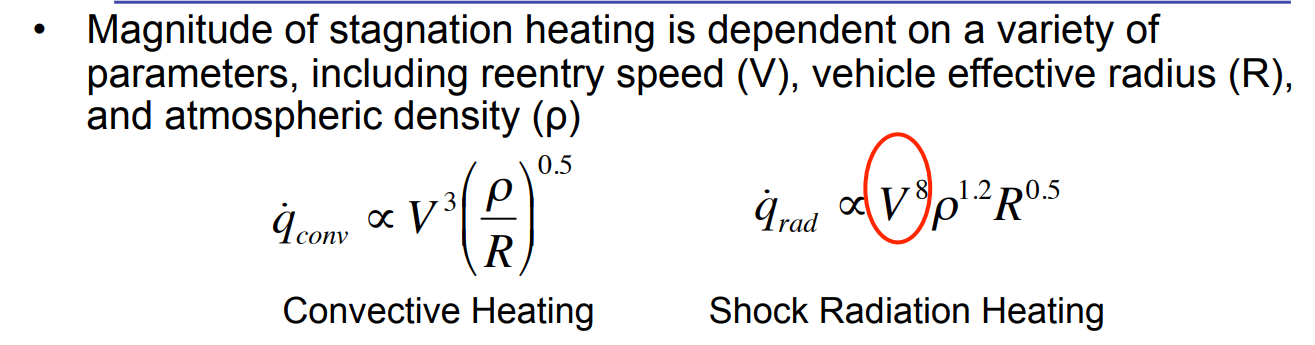 (slide 8)
(slide 8)
I would also like to be able to reference a credible source that describes the equations that I end up using, and their limitations.
I'm willing to assume a non-ablative nosecone for now.
I'm also more interested in minimizing drag to get out of the atmosphere with minimal loss of speed, so this problem is different from the usual reentry problem where the goal is to slow down the vehicle without it burning up.
I don't want to blow up the scope of this question too much, but any additional advice from someone with expertise in this area would be very much appreciated. For example, what other information would an expert working on this problem feel is important to chart to assess the engineering feasibility of launching a vehicle with a specified shape along a certain proposed trajectory?
Update - just want to share a bit of what I discovered - it might help other people to get started. I found an relevant article called "Projectile Nosetip Thermal Management for Railgun Launch to Space" which, in turn, referenced a book called.
J. D. Anderson, Jr, Hypersonic and High Temperature Gas Dynamics. New York: McGraw-Hill, 1989, pp. 289–291.


