If you're asking about interstellar travel, then the answer is pretty simple; For diffuse, unfocused sources of light like the one emitted by stars, photon flux density decreases with the inverse square of the distance to its source, and with it photon pressure (imparted momentum of absorbed or, better yet, reflected photons) on the sail. So you accelerate away from the Solar system on photon pressure of the Sun, and decelerate on photon pressure of the destination star as you get closer to it. That might involve rotating the whole sail the other way around at half point, but that's it.
For more local orbits though, things get a bit more interesting. One thing we need to consider is that wherever in the Solar system we are, we're already in orbit around something. We're always orbiting some gravity well. And to move from one orbital altitude to another, say, decreasing heliocentric orbital altitude from one of the Earth (average of 1 AU) to one of Venus (about 0.7 AU), or increasing it to one of Mars (1.5 AU) and so on, we have to either increase angular momentum to move into a higher one, or decrease it to move into a lower one. So you don't really require the sail to be accelerating directly towards the Sun to decrease your orbital altitude with respect to it. It's enough to slow your orbital speed and with it decrease your angular momentum, and the orbit will progressively shrink to a lower altitude one.
OK, so how to do that with a solar sail? Well, simple enough. We need to set the solar sail's angle to the source so that the combined force vector of both incoming photon pressure and the reflected one (assuming 100% reflexivity at 0 angle to the source, total momentum imparted on the sail that results in forward motion is two times the force of photon flux*) points such that orbital speed is slowly reduced.
Saying it differently, and let's experiment a bit, if you take a mirror that will act as our solar sail, and point it to reflect some source of light at an angle on some wall, the combined force vector will point from between the source and the reflected light spot, and through the mirror away from that middle point. If you now imagine that you're circling that light source in some direction, if you pointed your mirror such that the combined force vector points more in the opposite direction of your orbit than going with it, you're lowering your orbital speed, and with it angular momentum and altitude.
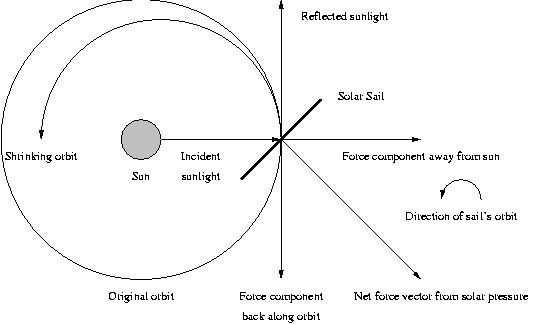
Does this make sense? No? No problem. See PearsonArtPhoto's answer here, from where I'm borrowing the image above, or Wikipedia on Solar sail - changing orbits. Now, of course in reality it's a fair bit more difficult than that. For one, solar sails don't work as momentum exchange devices only on photon pressure (or, if you want, radiation pressure) alone, but are also affected by particle pressure of solar wind. And that's already space weather, and as weather comes, it's a fair bit unpredictable and it can turn nasty. Our Sun is a relatively quiet, stable, invariable star, but destination star might not be. And sails don't come with perfect 100% reflectance in all the electromagnetic spectrum, and some of the incident radiation is absorbed, converted into heat and radiated away (which again imparts momentum on the sail) in multiple directions that might, or might not follow the shape of the sail. And you'll be doing a lot of sail angle changing, which might be achieved with orbit-synchronized rotation on own axes, during recapture at some distant gravity well, say to stabilize (circularize, reduce precession,...) an orbit around Venus when you depart from Earth's orbit, or something like this. But the point is, that sailing on solar pressure isn't really a straight line point to point travel. A solar sail might make many orbits around the Sun (read: years) to lower its orbit into heliocentric orbital altitude of, say, Venus. This is sometimes referred to as climbing up/down an orbit.
Such sails could however also be used with beamed, focused, and optionally polarized sources of light, which can in theory (if we built a capable enough source) start resembling more a true point to point travel, with far more straight trajectories between the points. Say, if we had a 50 GW phased array laser source (think happy thoughts, because that would take about 10x10 kilometers array of solar cells if they were orbiting the Earth, which might take a while to materialize), and assuming spot diameter not being any larger than the size of the sail and the sail's reflexivity 100% (i.e. 100% beam efficiency), we could get 100 kg from Earth to Mars in about 3.4 days without decelerating in nearly a perfectly straight line. And in 4.9 days with deceleration at our destination. You can read a bit more about such concepts in Relativistic Propulsion Using Directed Energy (PDF). Ideal trajectories for that (constant acceleration) also have a rather funny name - branchistochrone curve.
Deceleration with a more focused source like that (Sun can't really be considered a focused point source, it's much too big for that in the inner Solar system and it emits light in all directions), could be done by deploying a secondary sail that detaches from the spacecraft, acts as a beam concentrator and reflects it from the opposite direction back onto spacecraft's remaining sail. This works again because of the double momentum exchange with 100% reflectance, half as the photons hit the sail, and half as they are reflected away from it. And they might look something like this:
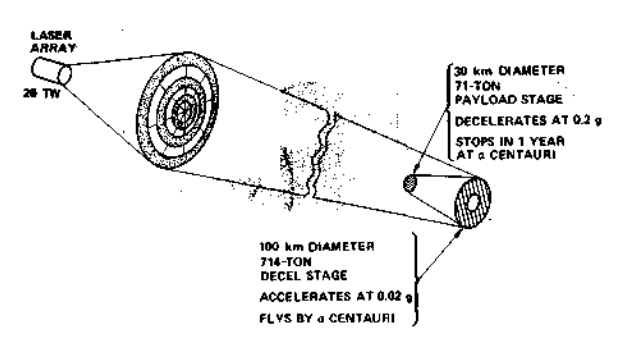
Author and source of the image is Robert L. Forward's Roundtrip Interstellar Travel Using Laser-Pushed Lightsails (PDF), where you'll find calculations and additional explanation of how it works. Or see this video of James Benford's presentation on Sailships during the 2013 Starship Century Symposium:
Alternatively, where solar sails, lightsails, or alike photon pressure sails wouldn't quite cut it, they could also be specially tailored (is that the word for manufacturing a sail?) to use other properties of destination's environment, such as having good magnetic reflectance to act as a magnetic mirror and also decelerate with the help of destination's magnetic field. And so on. But that's already cheating, right?
*It's also possible to polarize source of light and impart angular momentum of photons on the sail, which would result in sail's rotation. It does nothing for its forward motion, but it could be used to control sail's attitude from the light source, to send data to the craft, and/or to convert it into useful form of energy on the sail and use that to, say, generate own magnetic field.

