I was actually answering this question today regarding velocities of spacecraft, and it occurred to me I've never really looked at this graph in much detail before:
Heliocentric velocity of Voyager 2 plotted against distance from the sun

As you can see, after leaving Earth, the craft rapidly slowsslowed down (an example of the inverse square law), and it remainsremained below escape velocity until its encounter with Jupiter raisesraised its velocity by over 10 km/s. It receivesreceived another ~8-9 km/s slingshot from Saturn and another 1 km/s assist from Uranus.
However, the interesting event occursoccured at Neptune: It appears to have had a gravitational slowdown of approximately ~2 km/s, as opposed to an assist. Why was this?
This indicates to me that it took the opposite trajectory of this graph:
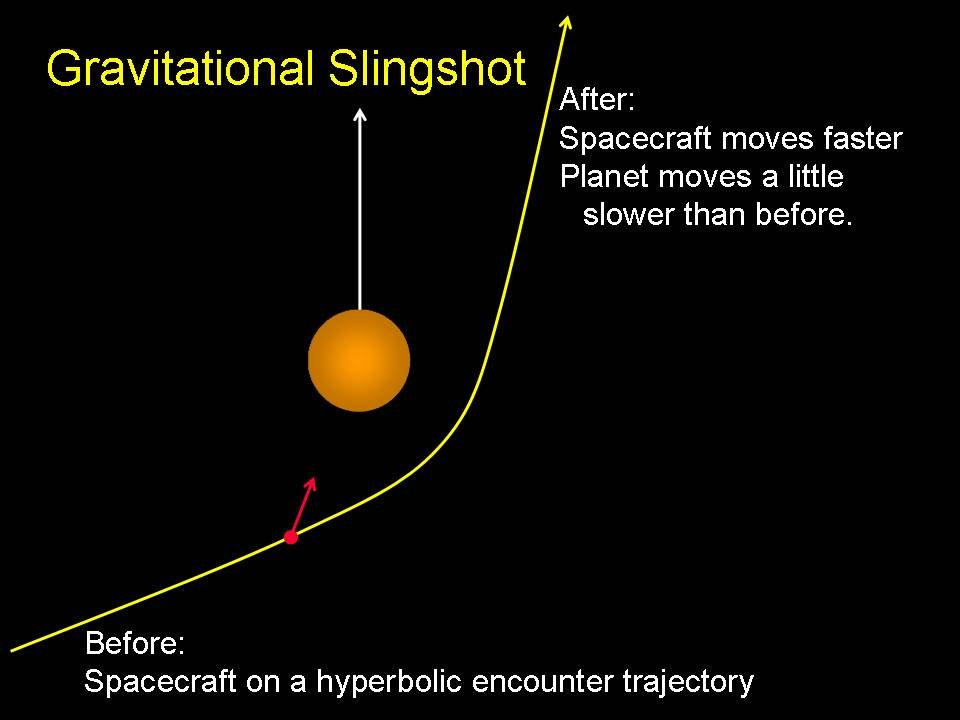
Instead of approaching the planet from behind its velocity vector, it flew in from the front and out the back.
I am aware that gravitational slowdowns can have many interesting uses, ESA's mission proposal for a Pluto orbiter require a gravitational slowdown at Jupiter to reduce its velocity for Pluto orbit insertion, so I suspect this was either a result of a science or engineering constraint.
Is there any explanation for why this was done?