The density of carbon dioxide on Venus varies from about 67 kg/m3 at the surface to about 52 kg/m3 at a height of about 5 km, calculated with this software tool
Image from Solar Powered Flight on Venus
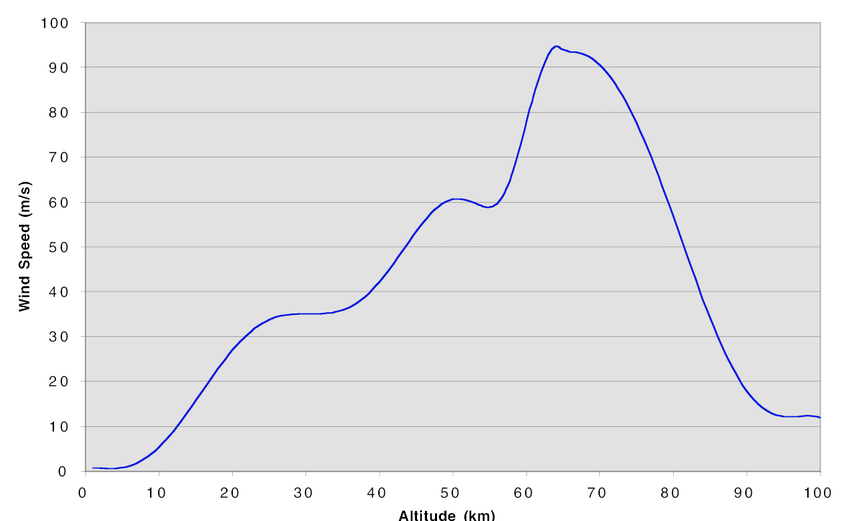
According to the graph above and Appendix A from the article the wind speed on Venus varies from 0.6 m/sec at the surface to 1.2 m/sec at a height of 5 km.
The dynamic force at the surface on 1 square meter would be: 1/2 x 67 kg/m$^3$ x (0.6$)^2$ m$^2$/sec$^2$ x 1 m$^2$ = 12.06 kgm/sec$^2$.
The dynamic force at a height of 5 km on 1 m$^2$ would be: 1/2 x 52 kg/m$^3$ x (1.2)$^2$ m$^2$/sec$^2$ x 1 m$^2$ = 37.44 kgm/sec$^2$.
Aphrodite Terra is the highland region near the equator that causes the wave in the atmosphere and looking at the image of its topography its height will be 5-7 km.
So we could assume that at a height of 5 km near the border of the highland the horizontal wind of 1.2 km/sec would be forced to go vertical, so the vertical dynamic force there would be about 37 kgm/sec$^2$.
So with a surface gravity of Venus being 8.87 m/sec$^2$ and a horizontal surface area of 1 m$^2$ for the spacecraft it probably could have a mass of several hundreda few kg to stay above the highland region !