tl;dr: No chance, not even close!
The escape velocity from the surface of a round (spherically symmetric) body is given by
$$v_{esc} = \sqrt{\left(\frac{2 GM}{r_0} \right)}, $$
showing that it is the $\frac{mass}{radius}$ ratio that's key here, not just the surface gravity given by
$$a_{g} = -\frac{GM}{r_0^2}. $$
So since
$$v_{esc} = \sqrt{a_g r_0}, $$
a lower density but larger radius body with the same surface gravity would have a higher escape velocity. You can think of that as "the gravity extending outward farther" or better yet, just dropping off slower. Gravity drops by a factor of 4 at $2r_0$, so if $r_0$ is bigger, so is $2r_0$.
The problem is a little tougher because you have to look at the design of human legs. They are optimized to work in Earth gravity; they have mass an moments of inertia that work with muscle strength and the speed with which muscle fibers can contract. For that you can start with this excellent answer to bibliography for the question Any scholarly or serious work in Sports Science for the low surface gravity of Mars or the Moon? or other things tagged reduced-gravity-sports.
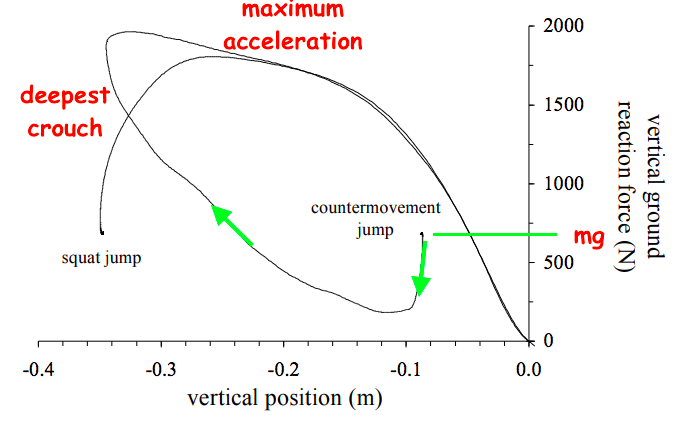
Let's look at what happens on Earth. Most people will find it a challenge to get to 1 meter in a standing high jump, and the world's record is 1.65 meters. Let's use 70 kg and 1 meter at $g_0$=9.8 m/s^2, some basic kinematics, and this page linking to the PDF Optimum Take-Off Range in Vertical Jumping to get a better picture
Published article Analysis of standing vertical jumps using a force platform Nicholas Linthorne, UNSW.
There's about a 1000 Newton force beyond the ~750 N supporting weight against gravity, or about 14 m/s^2, for about 0.25 meters. That's about 0.19 seconds and a take-off velocity of 2.6 m/s using $v = \sqrt{2 g h}$ and $t = \sqrt{2x/g}$.
The surface gravities of Mimas and Ceres are 0.064 and 0.28 m/s^2 respectively, and their escape velocities are
If you could develop only the 1000 Newtons over 0.25 meters at those surface gravities, you would also achieve that ~2.6 m/s velocity.
However, the surface gravities of Mimas and Ceres are 0.064 and 0.28 m/s^2 respectively, and their escape velocities are 160 and 510 m/s, respectively!
So... no chance, not even close!