5
$\begingroup$

Suppose we detect an object on an orbit that will pass close to Earth, entering our SOI, but escaping after just a few days. If we wanted to mount a mission to intercept that object, how would we go about determining the most delta-v efficient trajectory to accomplish it? Full disclosure, this is a question inspired by a situation in a game, but it seemed like it might be an interesting situation in real life as well.

As an aside, have we ever actually done a real mission like this?

My current thought is that, intuitively, you'd want to time it so that your interceptor's apoapsis intersects the periapsis of the inbound object, then match velocity at closest approach, but I have no idea if this is actually the most efficient way to do it.

Per request for more information (estimating how the game's situation would map to more real world units):

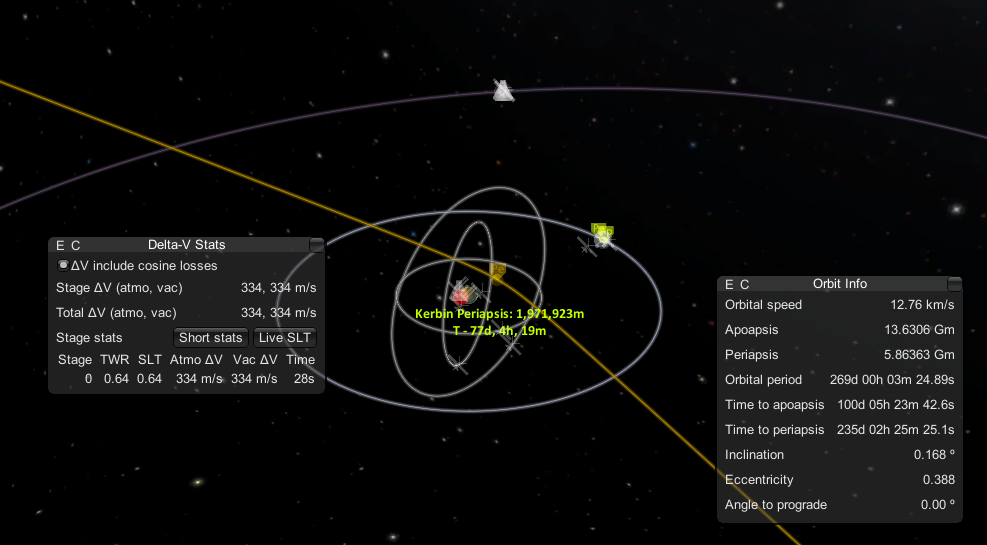
  1. Periapsis of inbound object to Earth is about 0.17 lunar distances.
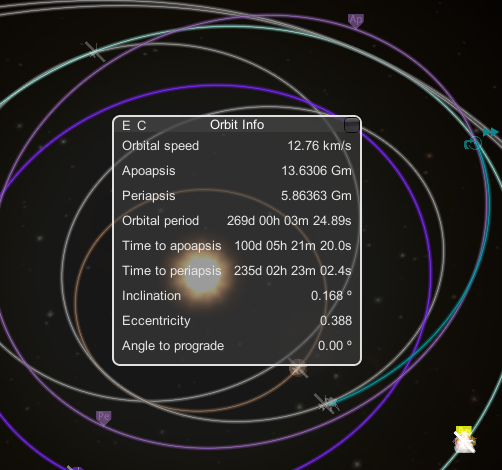
  2. Inbound orbital inclination (relative to sun) is 0.168 degrees.
  3. Eccentricity of orbit is 0.388.

I'm willing to do some math to figure things out, but I'm not sure where to get started.

Per request, here are images from the game situation.

Solar orbit of the inbound object (teal arc is inbound, violet is the estimated post-encounter orbit):

enter image description here

Orbit near Kerbin:

enter image description here

Improve this question
$\endgroup$
12
  • 1
    $\begingroup$ Well, correct me if i'm wrong, but the units are all real-world units, right? But Kerbin is smaller than Earth, that's relevant. As an orbital mechanics exercise, it holds up. Personally, i don't mind that it refers to an imaginary planet, but that is a question for the community. In order to give all the details needed, like the exact trajectory, i think you have to use the game's info. $\endgroup$
    – kim holder
    Commented Oct 31, 2015 at 15:31
  • 2
    $\begingroup$ For real world comparison, based on info you added, you'd be looking at orbits of Aten asteroids (Earth-crossers with semi-major axis < 1). You can find a list of some here or more here, then you can plug most of these in NASA Trajectory Browser search in the custom list, select other constraints and hit search. 2015 OQ21, 2007 CT26, 2004 KG1, 2014 WZ365, 2000 HB24 seem in reasonably similar orbits. $\endgroup$
    – TildalWave
    Commented Oct 31, 2015 at 16:56
  • 2
    $\begingroup$ Hyperbolic rendezvous has been studied in a few papers in JGCD: DOI 10.2514/1.62477, 10.2514/1.30071; Dynamics and Control: DOI 10.1007/bf02169490. $\endgroup$
    – Deer Hunter
    Commented Oct 31, 2015 at 18:05
  • 2
    $\begingroup$ Also this paper: Penzo, P. A., Nock, K. T., “Hyperbolic Rendezvous for Earth-MarsCycler Missions,” AAS Paper 02-162, 2002. $\endgroup$
    – Deer Hunter
    Commented Oct 31, 2015 at 18:22
  • 1
    $\begingroup$ @Deerhunter I'd guess if you want to run the iterations the gaussian variational equations coupled with your engine limitations would be a good starting point. For large impulse burn the loss of energy due to finite burn time can be quite significant (way more than 1% depending on the burn duration). $\endgroup$
    – ThePlanMan
    Commented Oct 31, 2015 at 20:36

1 Answer 1

Reset to default
3
$\begingroup$

The flyby would probably not be the best time to intercept (Unless manned). Usually the point where the object is furthest from the Sun is the key point to intercept. Specifically, a Hohmann transfer orbit. Of course, if the object has a particularly high inclination, it might help to intercept close to Earth. Of course, for these, a fly-by of Earth actually works better to adjust the inclination to a suitable approach vector for the asteroid.

For a manned mission, you would do something like the KSP path. The optimal point would probably be the point of closest approach. You would set up an orbit such that you were moving in the same direction (but not velocity) as the asteroid, and then accelerate near the point of closest approach to intercept the asteroid. This is exactly the optimal KSP mission, BTW.

From Kerbal Space program, it's much easier to intercept close because planning a maneuver for that lengthy of a time in advance is rather challenging.

As for real life, the closest I'm aware of is the Osiris-Rex mission, which will put a transmitter on an asteroid destined to pass close to Earth in about 200 years.

Improve this answer
$\endgroup$
4
  • $\begingroup$ Intercepting far from the Earth/K. is not acceptable because of real-life ECLSS limitations. Too much oxygen etc. required, and ground control is too far away to help with reducing navigation errors. A parking orbit, a hyperbolic (or elliptic) transfer, velocity-matching burn, approach and docking sequence... And yes, this is an exciting set of maneuvers. $\endgroup$
    – Deer Hunter
    Commented Oct 31, 2015 at 18:42
  • $\begingroup$ True, a manned mission would probably do a close intercept, but I don't see anything specific to manned missions in the request. Still, I'll edit appropriately. $\endgroup$
    – PearsonArtPhoto ♦
    Commented Oct 31, 2015 at 18:44
  • $\begingroup$ It is interesting to learn about more likely real-world case too; it sounds like that's basically the same idea as a transfer to another planet (i.e. another body also orbiting the sun.) $\endgroup$
    – Dan Bryant
    Commented Oct 31, 2015 at 18:52
  • $\begingroup$ It is exactly that (Except most planets are in essentially the same plane, making them easier than asteroids, which are often in different planes) $\endgroup$
    – PearsonArtPhoto ♦
    Commented Oct 31, 2015 at 18:53

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.