Using Space Stations in fixed orbits instead of Space Ships for the Whole Journey

Question

I'm very new, so I hope this question is within the guidelines of this group.

I remember an interview with Sally Ride where she mentioned using a larger habitat/space station that was put into a useful elliptical orbit with a useful eccentricity, and then using smaller space ships to dock with this larger one. (The advantage is the reusable station is big and can provide a better and safer habitat for the astronauts.)

I've been looking for a reference to this interview, but I haven't been able to find it.

So I've been trying to work out what sort of thing she meant, and this is what I've got so far.

Suppose we had a station whose orbit had half the orbital period of the moon. (Perhaps using an ion engine during an unmanned phase to establish that orbit.)

Every two orbits, the station could be in a position to be close to the moon. Using Kepler's 3rd law, (and dropping the units) $$ a=T^\frac{2}{3} $$ $$ a=(0.5)^\frac{2}{3} \approx 0.63 $$ So an eccentric version of this orbit could reach the moon (if it was smaller than 0.5, this wouldn't work).

If $r_a+r_p=2a$, then $r_p=2a-r_p$

If $r_a=1$, $a=0.63$, then $r_p=2(0.63)-1=0.26$

That eccentricity would be. $$ e=\frac{r_a-r_p}{r_a+r_p} $$ $$ e=\frac{1-0.26}{1+0.26} $$ $$ e \approx 0.6 $$

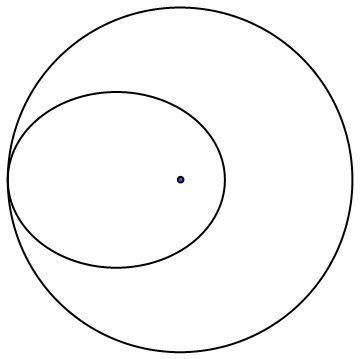
This orbit would look something like this.

I haven't seen anyone else at NASA mention this sort of station. What would be the pro's and con's of this? Is anyone aware of any careful analysis of the usefulness of an orbit like this (or something similar)?

---

Additional

There's a slick formula (I worked it out on my own, but I'm sure it's out there somewhere) to find the velocity for an elliptical orbit. $$v=v_0 \sqrt{1+e}$$ Where v_0 is the velocity of the circular orbit. And v is the velocity where the orbits intersect at one point. If we are talking about an orbit that is inside the circular orbit, then the value e is negative. In our case $$v=v_0 \sqrt{1-0.6} \approx 0.6 v_0$$ The orbital velocity for the moon is $$v_0 \approx 1.0 \; \frac{km}{s}$$ So $$\Delta v \approx 0.4 \frac{km}{s} \approx 400 \frac{m}{s}$$ But this doesn't take into account the orbital speed around the moon.

Answer 1

Similar solutions have been proposed for going to Mars as well. The upside is that you have a large vehicle for the long term (and dull) part of the mission that's safety critical - moving your people across deep space.

Downsides - you have to launch your station, that's hard. You have to resupply it in the short window it's near Earth. It has to payback it's initial cost quickly - if you're only going to use it a dozen times, and it costs 100 billion dollars... you'd be better off doing a custom build each mission, and upgrading it as you learn things.