tl;drs:
- Don't worry about the magnetic fields.
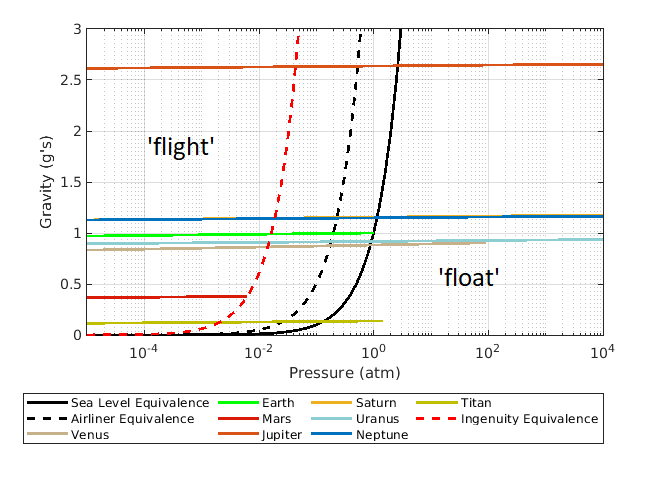
- Jupiter certainly has an altitude range with the same pressures at which we fly on Earth, just develop your aircraft aerodynamically to fly on Earth with 2.5 times as much weight as you'll fly there (simulating the 2.5 x higher surface gravity), and fly it there instead but good luck finding a power source! At 5.5 AU from the Sun sunlight is only 3% what it is here, so don't imagine a Solar Impulse-styled solar powered aircraft! Instead think more along the lines of a kilopower small space-rated nuclear reactor currently under development, or put a humungous solar collector farm in Jupiter orbit and beam kilowatts of power to your aircraft using a laser or microwave beam while they are in line-of-sight contact, and then let it operate as a glider (if possible, though at 2.5 g it's going to be rough) during the time when they are out of sight. Batteries are probably going to be too heavy in this gravity, I think you will need a nuclear reactor or solar farm one way or the other.
We are used to handling small magnets where the gradients are large, the field near each pole surface is much larger than just a few cm away, so ferromagnetic metal objects are strongly attracted to the poles.
In a nearly uniform magnetic field, a piece of steel or iron is not pushed or pulled at all. There is no gradient, so there's no decrease or increase in energy moving in any direction.
There certainly can be torques on the object about its center of mass, but those can be zeroed out by distributing any ferromagnetic materials (or internally generated fields due to permanent magnets or electromagnets) through some careful engineering by balancing their distribution, adding some extra electromagnets to null the torque (magnetotorquers) or just plain internal shielding or flux closing, like the yolks around motors that have permanent magnets inside.
The magnetic part (2nd term) of the Lorentz force
$$\mathbf{F} = q \mathbf{E} + q \mathbf{v} \times \mathbf{B}$$
says that there is a force on a charge that's moving in a magnetic field.
If the field is uniform and the charge is in a conducting loop like a circuit or a metal satellite frame, then this will induce no net current in the loop, so don't worry about eddy currents producing drag and loss of altitude (at least to first order).
What will happen though is that the Lorentz force will tend to push charges to one side of the spacecraft; there could build up a small static dipole charge across the craft pushed there by the Lorenz force until their electric field were just strong enough to cancel the Lorentz force.
$$F_E = qE = qvB$$
or just
$$E = vB$$
We can calculate the size as follows. The equatorial field strength of Jupiter at the surface is about 4.2 Gauss, or 4.2$\times 10^{-4}$ Tesla. The standard gravitational parameter of Jupiter is 1.27$\times 10^{17}$ m3/s2 so the orbital velocity at Jupiter's equatorial radius of 7.15$\times 10^{5}$ meters we can calculate the orbital velocity of a Karman plane to be 42,000 m/s (82,000 knotts) using the vis-viva equation
So at orbital speed in our Karman plane which hopefully you won't be doing, the field built up across a 1 meter wide spacecraft at the 1 atmospheric pressure point (1 bar) of Jupiter's atmosphere will be 17.7 volts, using
$$\Delta V = dE = dvB$$
where the voltage difference $\Delta V$ is equal to the distance across the spacecraft $d$ times the electric field $E$.
At a nice Piper Cub cruising speed of 34 m/s it's only 14 millivolts, so not much to worry about.
Basically, just build your craft to work in 10 gauss and you can fly pole to equator (where it's double the strength at the equator and pointing up/down) without worries.