Ratio of low-thrust slow spiral to Hohmann transfer $\Delta V$?

Question

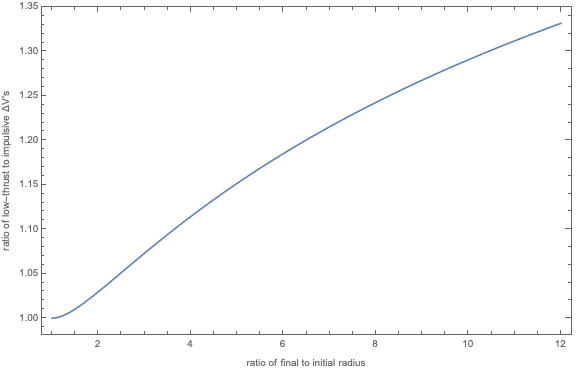
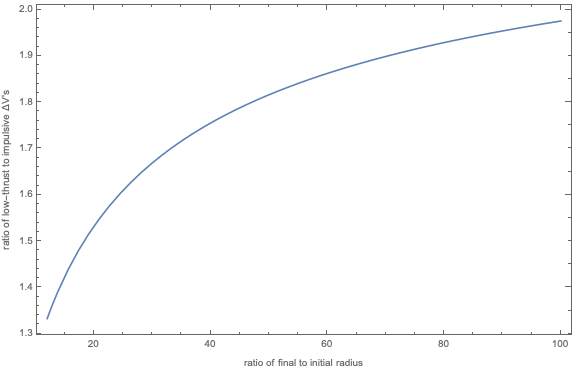
Going from one circular orbit to another, there are tables out there and equations that give the total $\Delta V$ needed for a two or three impulse maneuver scenario.

For a very low thrust propulsion system, one would just spiral from one circle to the other. For finite thrust the final orbit wouldn't be a perfect circle for a simple continuous burn (there's a tiny wiggle due to abruptly turning the engine on and off at the beginning and end) but let's look at the limit of very low thrust and very long "burn" time.

If necessary, also assume the high voltage engine consumes a negligible amount of propellant mass.

In this limit, how much more $\Delta V$ is needed for the low-thrust spiral compared to a two-impulse Hohmann transfer or a three-impulse bi-elliptic transfer, from one circular orbit to another?

Ideally a plot of the ratio as a function of initial to final radius ratio, but if that's not possible, at least calculate Earth to Mars.

Answer 1

This answer has the two-impulse Hohmann transfer $\Delta V$. It is:

$$\sqrt{2x\over x+1}+\sqrt{1\over x}-\sqrt{2\over x\left(x+1\right)}-1$$

where $x$ is the ratio of the higher orbit radius to the lower orbit radius, assuming (without loss of generality) that the lower orbit radius is $1$ and $\mu$ is $1$.

This answer notes that in the limit of very low thrust, the $\Delta V$ between two circular orbits is simply the difference of the velocities of the two orbits. With the same assumptions we have:

$$1-\sqrt{1\over x}$$

Plotting the ratio:

That was plotted up to about where a Hohmann transfer is always lower $\Delta V$ than a bi-elliptic transfer. Above that, we have for a bi-elliptic transfer with the intermediate apoapsis at $\infty$, again with the same assumptions:

$$\left(\sqrt{2}-1\right)\left(1+\sqrt{1\over x}\right)$$

Continuing the plot:

That asymptotes out to $\sqrt{2}+1$, as noted in this answer.

---

As a side note, the $x$ value above which a bi-elliptic transfer at $\infty$ is better is the largest real root of $x^6-14 x^5+23 x^4+20 x^3-9 x^2-6 x+1=0$:

$$\frac{1}{3} \left(7+4 \sqrt{2}+\frac{2 \sqrt[3]{2} \left(11+9 \sqrt{2}\right) \cos \left(\frac{1}{3} \tan ^{-1}\left(\frac{3 \sqrt{303+216 \sqrt{2}}}{395+268 \sqrt{2}}\right)\right)}{\sqrt[6]{9450+6677 \sqrt{2}}}+2 \sqrt[6]{2 \left(9450+6677 \sqrt{2}\right)} \cos \left(\frac{1}{3} \tan ^{-1}\left(\frac{3 \sqrt{303+216 \sqrt{2}}}{395+268 \sqrt{2}}\right)\right)\right)$$

which is $x\approx 11.93876547$.