Looking at images of the DART space craft with the solar cells fully extended, the solar cells on each side of the craft are offset from one another, instead of being inline, as the picture below shows.
Why are the solar cells offset? Is it because of a mass distribution issue, or issues relating to possible rotation of the craft as the cells are rolled out, or something else?
A guess rather than an answer.
I suspect it is because attaching the solar arrays at the middle of each face will create a conflict with the High-Gain Antenna (either accomodation or pointing masking).
This can be inferred for example from this picture taken from NASA Gallery (Dart gets its 2nd wing)
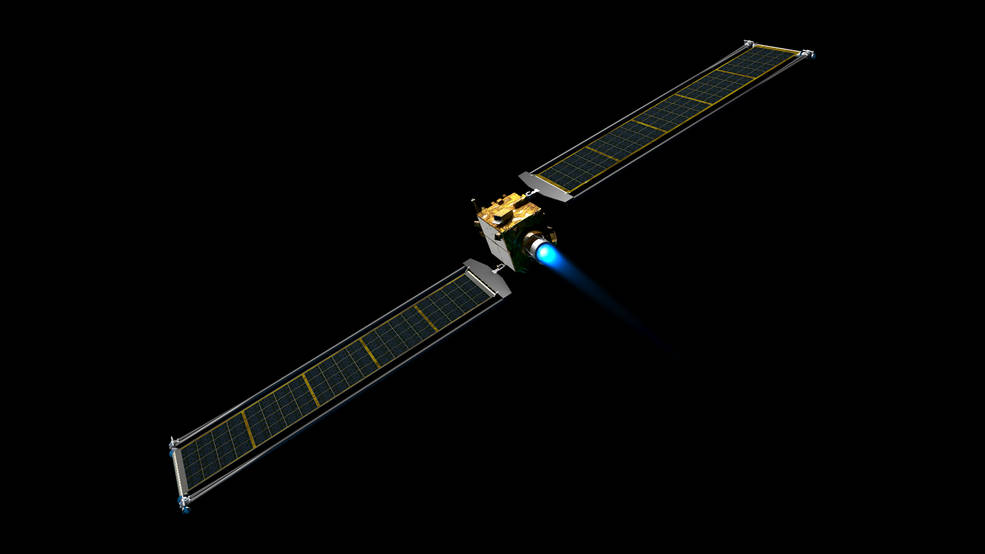
I don't know for sure, but I think it's because the panels start rolled up into a cylinder on each side, like this:
O$\square$O
If the cylinders were attached to the rectangular box in the center of opposite faces, like O-$\square$-O , then the first turn (the right-angle junction between the $-$ and the O) would be quite sharp, so it might break the panel components, and it folds in the opposite direction from the rest of the unwinding.
If instead you attach the cylinders at the corners the way DART has done, like O_$\square\bar{}\!$O , then the attachment point is already properly oriented, and the initial curvature is the gentlest possible.
The result, as Jörg said, is symmetrical: ___$\square\bar{}\!\bar{}\!\bar{}$ is symmetric under rotation, just not reflection. $-\square-$ is symmetric under both (or would be, if I could get the vertical spacing right in my $\LaTeX$ ascii art). If you mean why use ___$\square\bar{}\!\bar{}\!\bar{}$ rather than $\bar{}\!\bar{}\!\bar{}\square\bar{}\!\bar{}\!\bar{}$ (symmetric under reflection but not rotation), it's because in the way they did it, the center of mass stays near the geometric center of the spacecraft. If they attached both wings to one face, the center of mass would move toward that panel as the booms unrolled, which would make maneuver control trickier.
