Interpreting phasing maneuver results using electric propulsion in GMAT

Question

I'm trying to simulate an anomaly phasing maneuver in three different scenarios using electric propulsion in GMAT. The idea is to use the altitude difference (of ~7-8km) between two spacecrafts to investigate the anomaly drift after 11 days. Since the orbits of both S/Cs are circular and near-equatorial, I used GMAT's TLONG (RAAN + AOP + TA) to quantify the anomaly phasing.

Scenario 1

The first scenario serves as baseline, where S/C 2 (~570km) maintains a ~7km higher altitude than S/C 1 (~563km) from the start till the end of the simulation. No maneuvers were performed for either S/Cs. After 11 days, the longitudinal drift was about 91.4 degrees. (Simple math shows this to be accurate)

Scenario 2

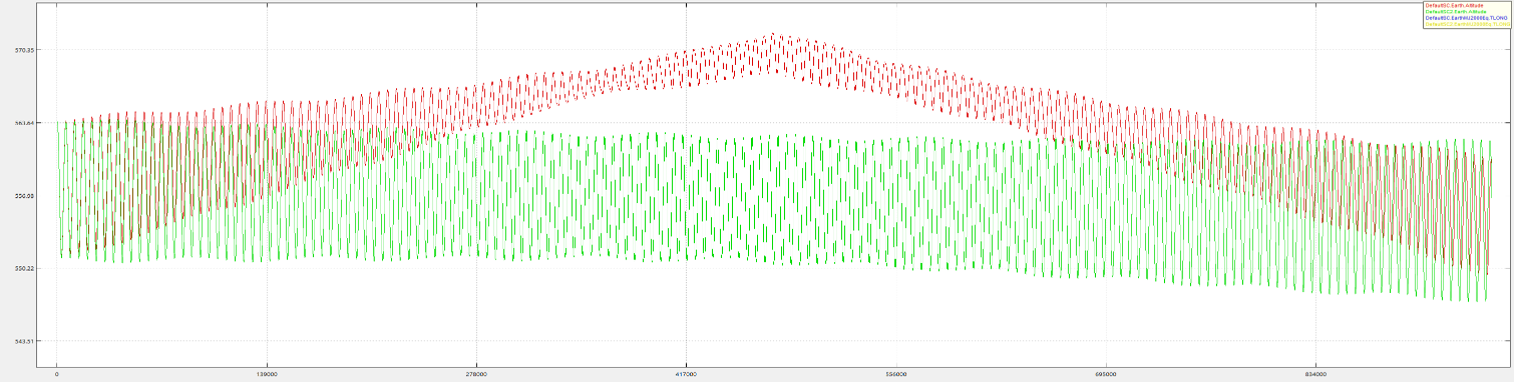
The second scenario starts out with both S/C at the same altitude of ~563km. S/C 1 then undergoes a continuous finite burn for 5.5 days, increasing its altitude to ~570km, before another finite burn for 5.5 days back down. The altitude profile of both S/Cs can be seen below. (Red line is S/C 1 and Green Line is S/C 2)

After 11 days, the longitudinal drift was similar to scenario 1: about 90.8 degrees.

Scenario 3

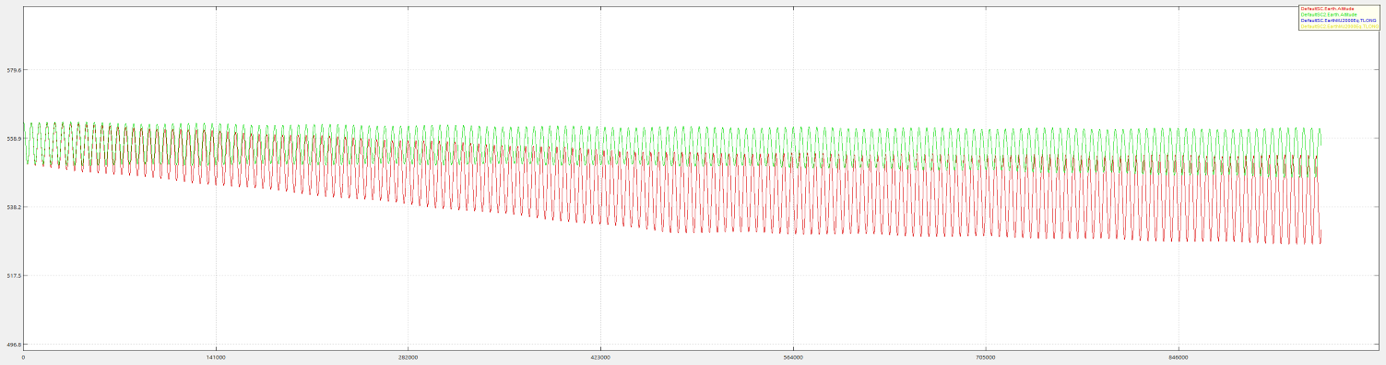
Lastly, the third scenario is the inverse of the second. S/C 1 undergoes a finite burn down for 5.5 days, before a finite burn back up for 5.5 days. The altitude profile of both S/Cs can likewise be seen below (it appears that upwards burn did no effect).

The longitudinal drift after 11 days was quite different, at 136.6 degrees.

My questions are these:

1. Why is the longitudinal drift in the second scenario similar to the first scenario? Intuitively, a larger altitude differential would mean a larger drift rate. I would expect scenario 2, having no altitude differential at the start, to have lesser longitudinal drift compared to scenario 1.

2. Why is the altitude profile of S/C 1 so dissimilar in scenario 2 and 3? The thruster and burn characteristics used for both scenarios are identical. The only difference is the order of the burns.

3. Accordingly, why is the longitudinal drift for scenario 2 and 3 so different?

Related: Analysing a phasing maneuver using GMAT- how do I reliably quantify their "phase difference"