In theory, yes, one could build a bridge.
There are several potential problems.
The first problem is that no known natural orbit is circular; all of them are ellipses. If, for example, the semi-major axis of the co-orbital elipse is 11000km, and the semi-minor axis is 10000 km, you need 1000km of "flex" in your bridge.
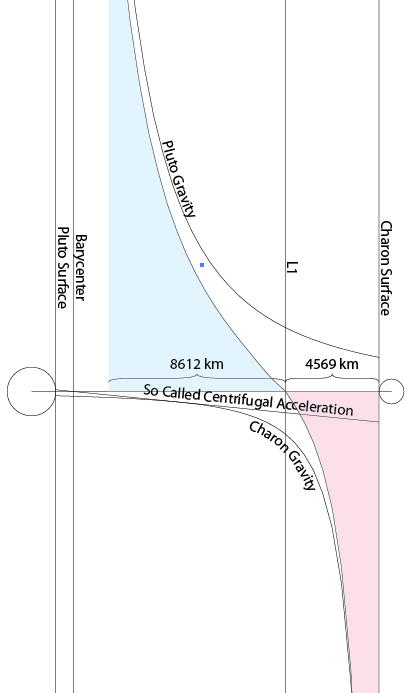
Further, the "stable" point in the middle is not all that stable, If the two are not the same mass, the L1 point will be closer to the smaller body while the barycenter is closer to the larger. And L1 points are not actually all that stable, anyway.
Since the stable orbital balance point is harder to establish, one cannot "hang" the bridge. If one cannot hang the bridge, then one must push up the bridge. Compressive strength is a major flaw; at present, all known materials require hanging the elevator from orbit, rather than building it up, and even then, only carbon nanotube is viable for Earth orbit.
Given the issue of putting the thing into orbit, the geosynchronous point will be the L1. "stable" L1 orbits are not points, but complex wobbles around the L1 point, due to N-body solution issues. This instability of the L1 makes the initial station unable to be actually all that geosynchronus.
Further still, even tho tidally locked, all know tidally locked bodies "wobble" - the lock isn't absolute, and their orbits are also inclined versus the equator. This means that you have to allow for the non-match - the anchor point must move, or the system must flex from the L1.
This makes, for this author, the bridge to be unlikely, at least barring some form of orbital alterations to eliminate the inclination and eccentricity.