CanCould Apollo's Sextant navigation for Cislunar Celestial Navigation use similar geometry as Marine Navigation?
My grandfather would be familiar with the instrument in this astronaut’s hands, although he would be puzzled by the view out the window.
There are numerous internet references to Apollo spacecraft using a sextant for cislunar celestial position fixes but I’m unable to find an explanation of the geometric method used.
The sextant on Apollo had 3 functions:
- Attitude measurement using star sights. This was used to correct drift in the Inertial Measurement Unit (IMU) utilizing programs P51 and P52 https://web.mit.edu/digitalapollo/Documents/Chapter6/hoagprogreport.pdf . This is analogous to aircraft zeroing their gyrocompass on their runway heading before take off.
- Orbital fixes while in low Earth and Lunar orbits, using known surface landmarks. This is analogous to Marine Coastal Piloting, which uses previously surveyed shore landmarks.
- Celestial fixes during Cislunar Navigation. This used star and horizon sights, along with the Apollo P23 program. It is this Cislunar Navigation which I am puzzling over. It is analogous to offshore Celestial Marine Navigation.
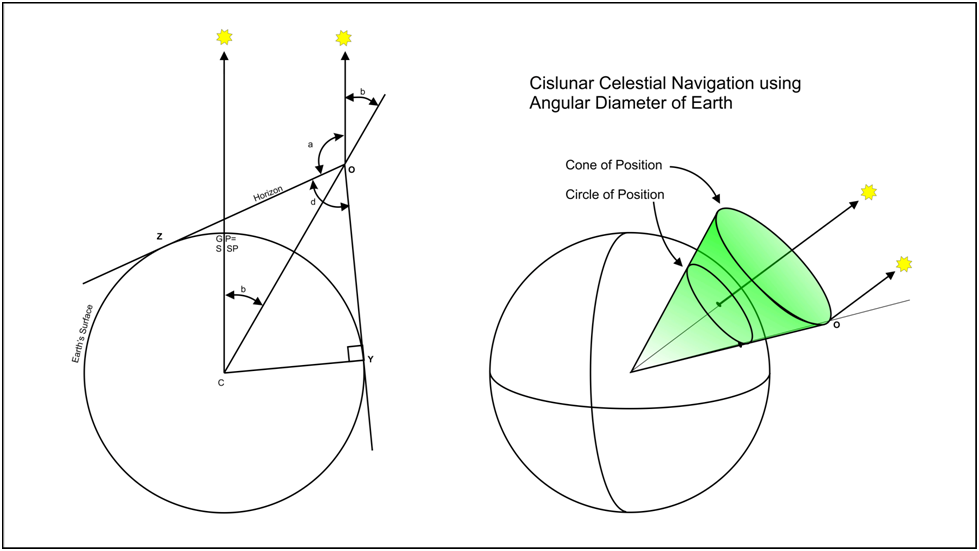
Offshore Marine Celestial Navigation uses the horizon as a horizontal reference plane with which to measure the angular altitude of a celestial body. This provides a circle of position on the Earth (and celestial sphere). Three or more circles from different celestial bodies will provide intersecting circles of position, giving a fix.
This method will not work in a spacecraft. The horizon will no longer provide a planar reference surface, but instead a conical reference. (Aircraft sextants dodge the absent horizon problem by using an internal artificial horizon. But a bubble-type artificial horizon will not work in free fall.)
Sighting on the visible horizon from space provides a “cone of position”. The apex of the cone is at an unknown altitude. Three such cones from observation of three different celestial bodies could give a 3D fix if you have computer program clever enough to figure out the conic intersections.
Another possible method is to go back to traditional marine celestial navigation by obtaining three circles of position. A sight on the Earth’s diameter would give the altitude of the observer. It would also allow the apparent angular altitude of the celestial body “a” to derive “b”.
In the sketch, “a” and “d” would be measured. Since the radius of the earth and all angles are known, the observer’s altitude can be calculated. Three celestial observations give three intersecting circles of position on the celestial sphere. This can be translated into geographic position if desired.
Sextants (including the ones on Apollo) are calibrated to 10 seconds of arc. Lunar diameter measurements could approach this accuracy, but Earth diameter measurements would be hampered by the “fuzzy edge” of the atmosphere. In Apollo 8, Jim Lovell was “remarkably consistent” in his choice of apparent horizon altitude. But he was using a single sunlit horizon and a star, not one sunlit and one night time horizon (which would often be necessary to measure earth diameter).
Does anyone know what geometric method was used for cislunar celestial navigation in Apollo? Anyone think my Grampa’s Marine Celestial navigation could be used in space (with addition of Planetary Diameters) ?