What is the closest distance a human being has come to Mars ever since the beginning of the space age?

Question

Ever since we started space exploration, what is the closest a living human being has come to Mars?

Answer 1

Let's start with some basic facts, which helps narrowing it down:

• Humans have not been outside the Earth-Moon system.
• Except from the Apollo program, humans have not been outside low Earth Orbit.
• The distance between the Earth and Mars varies in a 2.13 year long cycle. Some of these minima are lower than others.

As a first approximation, everyone on Earth has roughly the same distance to Mars during these minima, which are 78.3 million kilometres on average.

But the orbit of Mars is not circular, and neither is the orbit of the Earth. They also have a slight relative inclination, and the closest lineup is not going to happen in the same location each time.

Over the course of history, one of these lineups must have been the closest, with some unknown human being the closest. But since you specify "since the beginning of the space age", we can do better.

The extra distance of Apollo astronauts of 0.4 million kilometres is significant, but those missions didn't happen close enough to the Earth-Mars minimum distance to compete, so those are ruled out. (uhoh covers this in greater detail)

The closest approach of Mars since the beginning of the space age happened at August 27, 2003 at 9:51 UTC at 55,758,006 kilometres centre-centre. (apparently the closest one in 60,000 years, and the closest one until 2287. I find the large difference between these two numbers somewhat suspicious).

But how long was this window?

At that moment, Earth and Mars must have had no relative velocity along the distance axis, otherwise, the close encounter would have happened slightly before or slightly after. This situation doesn't last long, and the distance drifts in this way, both before and after the closest approach:

• Over 1 minute, it increased by 8 metres.
• Over 1 hour, it increased by 31 km.
• Over 2 hours, it increased by 124 km.

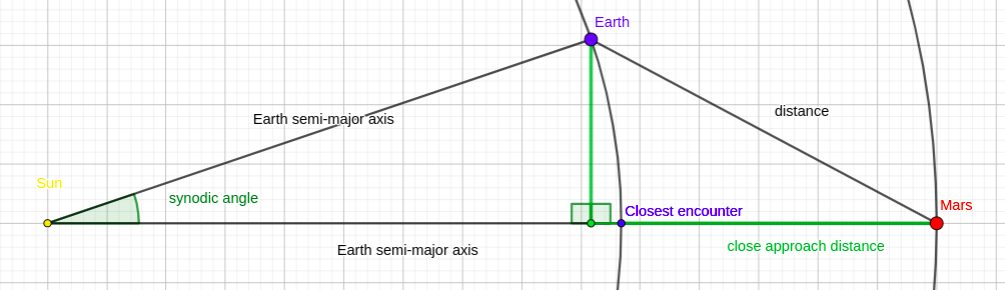
$$\theta_{synodic} = \frac{2\pi \Delta t}{P_{synodic}}$$

$$\Delta dist_{vertical} = \sin{\theta_{synodic}} \cdot a_{Earth}$$

$$\Delta dist_{horizontal} = (1 - \cos{\theta_{synodic}}) \cdot a_{Earth}$$

$$distance = \sqrt{\Delta dist_{vertical}^2 + (\Delta dist_{horizontal} + dist_{closest})^2} - dist_{closest}$$

Since that's a time difference both ways, a person on a mountain or aboard an air plane in the correct hemisphere, at the best latitude, has a good chance of being the winner, with a somewhat lower chance over Africa.

A complication is the International space station: It's pretty close to Earth, so unless it happened to have an inclination of less than 19.5 degrees relative to the direction of Mars, it would not have come closer than other parts of the Earth. Until I can find the orbital data required, the expedition 7 crew of Yuri Malenchenko and Ed Lu have a 22% chance of being the winners.

Update after checking the orbit of the ISS: Sadly, The ISS has its "high beta" seasons in mid May and November, which are maximally bad for being close to Mars. Since the end of August is only a little over one and a half month away from that, the relative inclination of the station would not be low enough to secure the record.

Further update, after finding the "best" latitude (10° 08' south):

(update: Cornelisinspace worked out more accurate coordinates)

The best longitude (147° 45' west )on the other hand would have been where it was midnight at 9:51 UTC, which happened in the Pacific ocean.

Both the latitude and longitude is quite sensitive. Since this is ocean, it's a competition in "who was closest to 10° 08'N 147° 45'W"

Did any plane cross the 10° 08' degree line in the pacific ocean at local solar midnight on August 26/August 27? If not, the winner is some passenger on a ship, or otherwise, a high-altitude person on a pacific island.

(The terrain on the Martian side also matters)

The distance is then: 55,758,006 km, minus the radius of the Earth, minus the radius of Mars, with a couple of kilometres of uncertainty for the exact terrain on Mars and for who on Earth actually was the closest.

Answer 2

Even if Apollo 11's crew would build a Moon base in 1969, and lived there until now, they would not be closer to Mars than without such base!

Mars approaches Earth during opposition every couple of years. However the distance between Mars and Earth varies a lot between these approaches because of eccentricity of Mars and Earth orbits. From Mars opposition table you can see that the closest opposition was Aug 28, 2003. The closest approach was actually a day earlier Aug 27. The closest distance on that day was 55,758,006 km. The next closest opposition was in 1973, which was 56.20 millions of km. The difference between these two approaches was larger than the maximum distance between Moon and Earth (which is ~0.4 millions of km). So, the Moon had only one chance to beat Earth's record for closest approach to Mars after 1969: around Aug 27, 2003.

Unfortunately, Aug 27 was a new moon (at 17:27). It means that Moon was between Earth and Sun, so it was farther from Mars than Earth. It could be closer to Mars only ~7 days before or after Aug 27. Unfortunately, at that time the distance between Mars and Earth was too big. Using calculator we can see that Aug 20 Mars was 0.47 Mkm farther than closest approach, so Moon had no chance to beat Earth.

It means that building Moon base in 1969 would not get us any closer to Mars!

Answer 3

It could have almost been an Apollo astronaut!

but only during 1969-1972, and it wasn't.

I think I can rule it out conclusively.

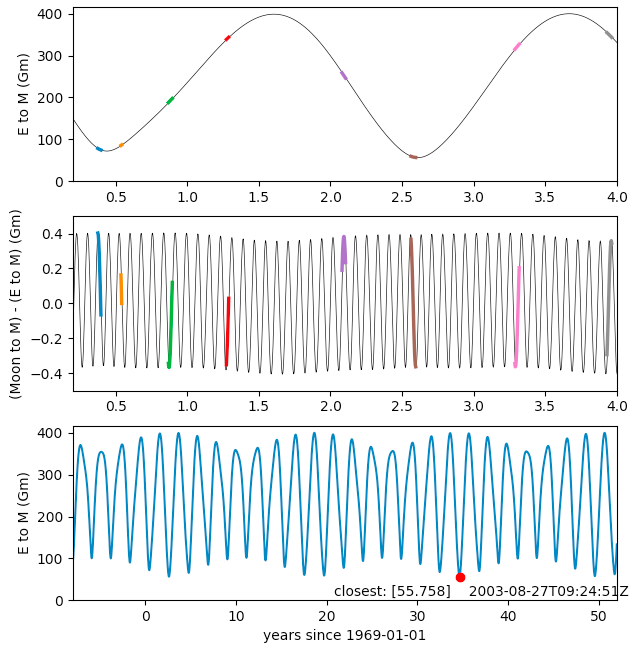
55.758 million km on 2003-08-27 is the closest the Earth has been to Mars since 1961. No trip to the Moon got closer.

---

I took Apollo 10 through 17 dates and plotted them on the distances of Earth to Mars, and to the difference between Moon to Mars and Earth to Mars just for fun.

I thought I'd try to simply look at actual data.

There is no chance that an astronaut got closer to Mars during an Apollo mission than Earth itself did since 1961.

hastily and poorly-written Python script:

from skyfield.api import Topos
from skyfield.api import Loader
import numpy as np
import matplotlib.pyplot as plt

from skyfield.api import load
loaddata = Loader('~/Documents/fishing/SkyData')  # avoids multiple copies of large files

ts = loaddata.timescale() # include builtin=True if you want to use older files (you may miss some leap-seconds)
eph = loaddata('de421.bsp')

earth, moon, mars = [eph[x] for x in ('earth', 'moon', 'mars')]

apollos = [(10, 1969, 5, 18, 26), (11, 1969, 7, 16, 18),
           (12, 1969, 11, 14, 24), (13, 1970, 4, 11, 17),
           (14, 1971, 1, 31, 40), (15, 1971, 7, 26, 38),
           (16, 1972, 4, 16, 27), (17, 1972, 12, 7, 19)]
# https://en.wikipedia.org/wiki/Apollo_program

timez_apollo = []
for n, year, month, d_start, d_stop in apollos:
    times = ts.utc(year, month, range(d_start, d_stop+1))
    timez_apollo.append(times)

days = 1 + np.arange(5*365.2564+1)
times = ts.utc(1969, 1, days)
years = days/365.2564
t_1969 = times.tt[0]

epos, moonpos, mpos = [x.at(times).position.km for x in (earth, moon, mars)]
r_earth = np.sqrt(((epos - mpos)**2).sum(axis=0))
dr_moon = np.sqrt(((moonpos - mpos)**2).sum(axis=0)) - r_earth

fig = plt.figure()
ax1 = fig.add_subplot(3, 1, 1)
ax2 = fig.add_subplot(3, 1, 2)
ax3 = fig.add_subplot(3, 1, 3)
ax1.plot(years, r_earth/1E+06, '-k', linewidth=0.5)
ax2.plot(years, dr_moon/1E+06, '-k', linewidth=0.5)
for timez in timez_apollo:
    yearz = (timez.tt - t_1969) / 365.2564
    epoz, moonpoz, mpoz = [x.at(timez).position.km for x in (earth, moon, mars)]
    r_earthz = np.sqrt(((epoz - mpoz)**2).sum(axis=0))
    dr_moonz = np.sqrt(((moonpoz - mpoz)**2).sum(axis=0)) - r_earthz
    ax1.plot(yearz, r_earthz/1E+06, linewidth=2.5)
    ax2.plot(yearz, dr_moonz/1E+06, linewidth=2.5)
ax2.set_ylim(-0.5, 0.5)
ax1.set_xlim(0.2, 4.0)
ax2.set_xlim(0.2, 4.0)
ax1.set_ylim(0, None)

timesbig = ts.J(np.arange(1961, 2021, 0.001))

eposbig, mposbig = [x.at(timesbig).position.km for x in (earth, mars)]
r_earthbig = np.sqrt(((eposbig - mposbig)**2).sum(axis=0))
yearsbig = (timesbig.tt - t_1969) / 365.2564
ax3.plot(yearsbig, r_earthbig/1E+06)
closest = np.argmax(-r_earthbig)
ax3.plot(yearsbig[closest:closest+1], r_earthbig[closest:closest+1]/1E+06, 'or')

print(timesbig.utc_iso()[closest])

message_left = 'closest: ' + str(np.round(r_earthbig[closest:closest+1]/1E+06, 3)) + '  '
message_right = '  ' + timesbig.utc_iso()[closest]
ax3.text(yearsbig[closest], 10, message_left, ha='right')
ax3.text(yearsbig[closest], 10, message_right, ha='left')

ax3.set_xlabel('years since 1969-01-01')
ax3.set_xlim(yearsbig[0], yearsbig[-1])
ax3.set_ylim(0, None)

ax1.set_ylabel('E to M (Gm)')
ax2.set_ylabel('(Moon to M) - (E to M) (Gm)')
ax3.set_ylabel('E to M (Gm)')
plt.show()

Answer 4

It's very likely that inhabitants of the atoll Makatea in the South Pacific were the closest to Mars !

(As was discovered and announced by @SE-stop firing the good guys in one of his comments to this answer.)

Now that one of the other answers has ruled out any Apollo astronaut, and the ISS crew could be excluded too, we can focus on determining the exact location on Earth, and this can be done with the aid of the HORIZONS Web-Interface.
With the Table Settings Quantities 1 and 15, the Target Body Earth and the Observer Location Sun(body center) we can see the Sunsub longitude and the Sunsub latitude at the start of August 27, 2003.
Averaging for 9.51 UTC gives longitude 330.26405⁰ and latitude 10.210355⁰ for the place where the Sun would be in the zenith.
Comparing this table with one for Mars as the Target Body we see that the right ascension for Earth and Mars are almost equal at that special time, so the plane that contains the Sun, Earth and Mars was then almost perpendicular to the ecliptic.(and the angles of declination of the two planets would lie in that plane.)
If the Earth and Mars had also the same declination at that time, we just could draw a line from the "Sunsub" spot on Earth through its centre to the surface on the other side where Mars would then be in the zenith.(supposing the Earth would be a perfect sphere).
That "midnight spot" would then have coordinates 150.264⁰ West and 10.210355⁰ South.
But when we look at still another table with Target Body Mars but with this time Observation Location "Geocentric" at August 27, 2003, Mars has a considerable declination of over -15⁾, minus the declination of Earth of -10⁾ 9', and then averaged, what gives a total of -5⁰ 35' for 9.51 UTC.

That makes the final coordinates of the closest spot to Mars: 150⁰ 16'W, 15⁰ 47'S.
(somewhere between the atoll Mataiva and the island Huahine)

Using the R.A.(right ascension) on both the tables of Earth and Mars at august 27 and 28, 2003 gives an angular velocity for Earth relative to Mars of 75.08" per 24 hours at the time.
With Table Settings Quantities 19 and averaging for 9.51 UTC, the Sun-Earth distance was calculated to be 151,159,530.3 km.

The island Huahine is the closest to the 150⁰ 16' W, 15⁰ 47'S spot with a distance of 130 km, and by the curvature of the Earth that would make it 1325 m. further away from Mars at 9.51 UTC.
But 8 minutes earlier the closest point to Mars was near the atoll Makatea 213 km to the east, and with the equations in the answer from @SE-stop firing the good guys a distance of 258 m. further away from Mars was calculated.

The closest point on Mars to Earth at that time appeared to be 25.51⁰ E, 9.08⁰ S.