1
$\begingroup$

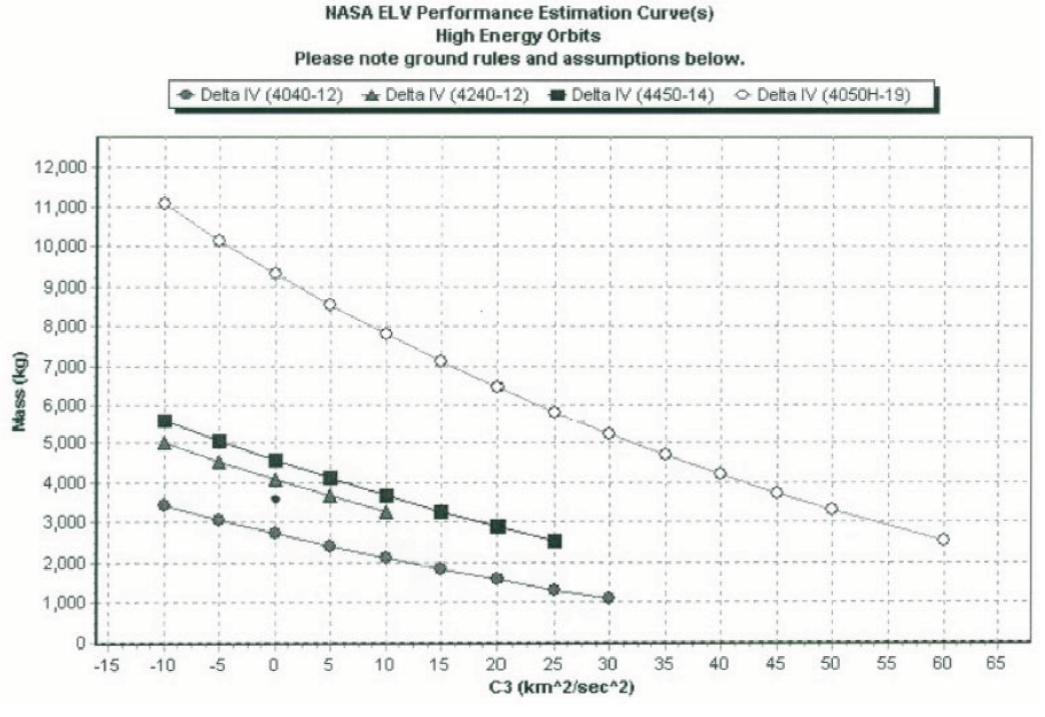
In this answer I've interpreted the x axis of the plot in the question (also shown below) labeled as $C_3$ (characteristic energy) to be the "excess $v^2$ above escape velocity from Earth", and so I'd written the relation.

$$C_3 = v^2 - v_{esc}^2.$$

Could I have some confirmation that this is a correct way to use the $C_3$ used in the plot? Am I missing a factor of 2 or are there other subtleties nor not-so-subtleties that I've overlooked?

The expression in @MarkAdler's answer is cast in terms of potential energy (per unit mass) but I've assumed conservation of total energy and applied it to kinetic energy (per unit mass).

The figure below is from the linked question, there is currently no original source listed for the image.

enter image description here

Improve this question
$\endgroup$

1 Answer 1

Reset to default
5
$\begingroup$

That is the formula. Wikipedia shows it a bit different:

$\tfrac{1}{2} v^2 - \mu/r = \text{constant} = \tfrac{1}{2} C_3$

Multiply by two, and the only question becomes the $2\mu/r$ term. Escape velocity is $\sqrt{\frac{2GM}{R}}$ per this source. If you square that, as you did in your initial formula, you come out with exactly the same thing as the Wikipedia article. Thus your formulation is correct.

As for the chart, it comes from Nasa's Performance Vehicle website.

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ yay! Thank you for the validation, now I can sleep soundly. $\endgroup$
    – uhoh
    Commented Mar 19, 2018 at 18:25

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.