Reading the linked questions, answers, and references (thanks @uhoh), particularly R. Whitley and R. Martinez, 2015, Options for Staging Orbits in Cis-Lunar Space and this figure:
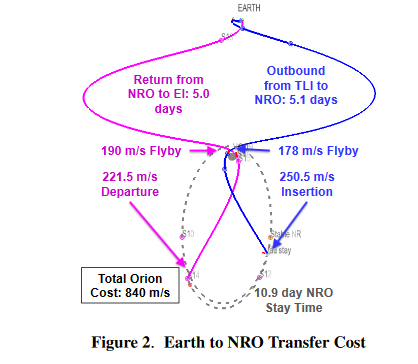
Leads me to believe that getting to a NRHO is much like an 'Apollo-style' lunar transfer (i.e., in plane, Hohmann-ish transfer orbit). The only difference comes in targeting the lunar flyby over one of the poles to displace the spacecraft out of the Earth-Moon plane (hence the term 'halo') and the 'capture' burn into the NRHO is slowing the spacecraft down (from its lunar escape trajectory). This removes a lot of 3-body dynamics shenanigans from the problem.
A good, quick way to calculate the required C3 is to pretend your rocket is performing a Hohmann transfer to the Moon's (average) orbital distance, 384400 km. From a 250 km low Earth parking orbit:
$$C3=-\frac{GM}{a}, a=\frac{6378km+250km+384400km}{2}=195514km$$
$$C3=-2.0\frac{km^2}{s^2}$$
I recall a professor (whose graduate research was designing lunar landers) saying a C3 value of $-1\frac{km^2}{s^2}$ is commonly used in early analyses.
This Apollo By the Numbers archived webpage (linked from the TLI Wikipedia) has the Apollo C3 data (converted to nice units):
| Mission |
C3 ($\frac{km^2}{s^2}$) |
| Apollo 8 |
-1.5 |
| Apollo 10 |
-1.3 |
| Apollo 11 |
-1.4 |
| Apollo 12 |
-1.8 |
| Apollo 13 |
-1.4 |
| Apollo 14 |
-1.7 |
| Apollo 15 |
-1.5 |
| Apollo 16 |
-1.6 |
| Apollo 17 |
-1.7 |
I don't know where you got the performance information but I hope it is NASA Launch Services Program Launch Vehicle Performance Website and the SLS mission planner's guide. Side note, New Glenn and Vulcan are now on the NLSP performance website!
