6
$\begingroup$

Suppose I designed a complex, self-contained, robotic system to harvest and convert Martian atmospheric CO2 and ground H2O into rocket fuel (CH4 + O2) and after exhaustive computational fluid dynamic (CFD) simulations, I wanted to test and verify convection and fluidic transfers under a simulated Martian gravity.

After reading @MBM's answer I realized that when flying a shallower profile with constant ground speed and parabolic-in-time altitude the simulated Martian gravity would point towards Earth at all time, and not the floor.

Since my apparatus is so large that it needs to remain parallel to the floor and can't fit on a rotating carriage, I need a flight profile that produces simulated Martian gravity that points towards the floor for as long as possible.

What would that modified flight trajectory be? Could an aircraft ever simulate Martian gravity perpendicular to the aircraft's floor?

Improve this question
$\endgroup$
20
  • 1
    $\begingroup$ I don't think the problem is the ground speed so much as constraining the attitude of the aircraft. For the zero gee case the plane has to fly at the zero lift angle of attack, for the Martian case there would likely be a specified angle of attack. $\endgroup$
    – Organic Marble
    Commented Apr 17, 2018 at 2:13
  • 1
    $\begingroup$ Maybe with rotating wings you could achieve this, but it is'nt a realistic solution... To answer, you could look up the vertical acceleration profile of AF 447, which should be in the order of magnitude of the max acceleration of a horizontal plane $\endgroup$
    – Antzi
    Commented Apr 17, 2018 at 2:39
  • 2
    $\begingroup$ Define "aircraft". Take a helicopter to service ceiling, cut rotor speed to produce 38% of hovering lift, fall until terrified? VTVL rocket? $\endgroup$
    – Russell Borogove
    Commented Apr 17, 2018 at 4:51
  • 1
    $\begingroup$ I was addressing your request for an estimate of duration, @uhoh, $\endgroup$
    – user20636
    Commented Apr 17, 2018 at 11:05
  • 3
    $\begingroup$ Or you could just design your plant to be insensitive to gravity, and verify by operating it right side up, up side down, and maybe also sitting on its sides for kicks. Or run it while rotating in two axes. $\endgroup$
    – Mark Adler
    Commented Apr 17, 2018 at 16:27

5 Answers 5

Reset to default
8
$\begingroup$

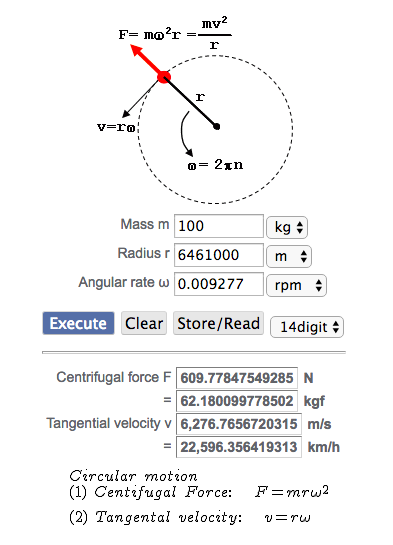
Okay, since the agreement seems to be that this is on topic and I'm a pilot I'll take a shot. The quick answer is yes, because the simulated gravity is generated from the airplane's lift, other forces are balanced out.

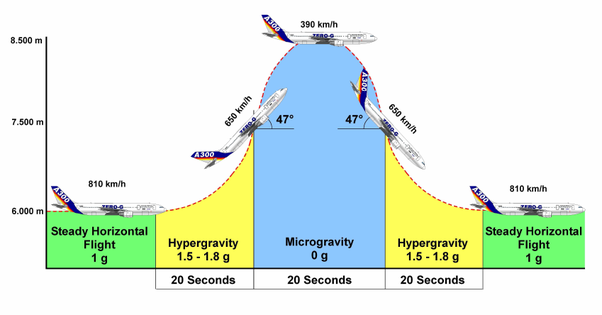
Longer answer is slightly more complicated: Can you simulate martian gravity using an airplane, absolutely yes. Here's how a parabolic flight profile looks: Parabolic arc

The airplane us pulled up into a steep climb, then the pilots begin a pushover which accelerates the airplane downward at 9.8ms2. In videos of the vomit comet you'll see people float off the floor at this point. The pushover is continued until the airplane is in a steep descent and picks up speed for the next iteration. It is the change from the airplane from a steep climb to a steep descent that creates simulated zero gravity, not the dive on the other side of the parabola.

The forces acting on the airplane at that point look like this:

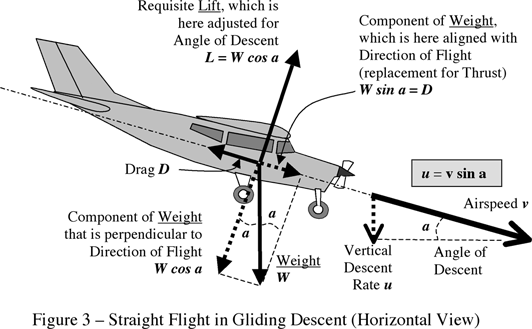
Forces acting on an airplane

The upward force felt by the passengers inside is perpendicular to the angle of descent, or climb (called the angle of attack, which is the angle of the wing through the relative wind). This is always point "up" in reference to the airplane, not in reference to the direction of gravity. So, in order to have your martian gravity the pilots would need to fly a profile that created a constant downward acceleration of 6.1ms2 (martian gravity is 3.7ms2, so 9.8 - 3.7 = 6.1) rather than 9.8ms2, which is as simple as putting less forward pressure on the stick. During that parabolic arc the wings are producing 3.7ms2 of lift.

You might think that a slower pushover means more time at martian gravity than zero gravity, however it's not that simple. As a pilot my main consideration would be to maintain a safe flight speed, the steep climb would put me behind the drag curve, and the slower pushover would allow more speed to bleed off. I might have to carry more airspeed or lessen the time of the maneuver, but it could be done.

Improve this answer
$\endgroup$
9
  • 2
    $\begingroup$ The question is about the direction of the force, not the magnitude. Whether Martian gravity can be simulated has already been asked and answered.space.stackexchange.com/questions/23448/… $\endgroup$
    – Organic Marble
    Commented Apr 17, 2018 at 15:59
  • $\begingroup$ I've answered that @OrganicMarble, apparent gravity is perpendicular to the angle of attack. If that's not coming out I'll work on it $\endgroup$
    – GdD
    Commented Apr 17, 2018 at 16:01
  • $\begingroup$ This is working in the right direction, but probably altitude, airspeed and angle of attack all have to be time dependent to make this work well. $\endgroup$
    – uhoh
    Commented Apr 17, 2018 at 17:21
  • $\begingroup$ Absolutely, the flight profile has to be executed precisely and has a narrow set of parameters @uhoh. $\endgroup$
    – GdD
    Commented Apr 17, 2018 at 17:27
  • $\begingroup$ How do you avoid a stall during such a steep climb? $\endgroup$
    – Cloud
    Commented Apr 18, 2018 at 6:36
4
$\begingroup$

Here is a non-parabolic approach:

An aircraft able to sustain straight and level powered flight at 90km altitude, at a speed of 22'596 km/h or 6276 m/s will feel 37.83% of Earth's gravity normal to aircraft's floor, which is Mars gravity, until it runs out of fuel. During powered flight it will circle the Earth every 1 hour 47 minutes.

North American X-15 was about 15'000km/h short on top speed to achieve this.

centrifugalforce

(source)

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ This is a great answer by the way! Technically the standard zero-gee "parabolic" flight is elliptical, with the far focal point at the center of the Earth, and circular flight is elliptical as well. So these are just two extremes of elliptical flight. You've found a way to stretch the period of sustained low gravity from several minutes to infinity though, which is quite cool! $\endgroup$
    – uhoh
    Commented Nov 6, 2018 at 12:44
3
$\begingroup$

When the Mythbusters were tacking the Apollo missions they did a lunar gravity walk in a plane flying parabolas. It certainly looked like the floor of the airplane was level by their perspective and it would have been pretty useless for their purpose if it wasn't. (The issue was the hopping locomotion the astronauts used. Obviously, the Mythbusters didn't have real space suits but they did have on a heavy and motion-limiting simulation of one--and found the hopping motion natural and easier than normal walking.)

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ Here is a clip, perhaps it's of what you've mentioned? I can see them hopping along and it's not horribly off-perpendicular, but it's hard to tell how close. I would guess then that the companies that offer this service (there must be more than one now) might even offer this as a product now, perhaps even with a perpendicularity spec. youtube.com/watch?v=OM5-BhMNXOI $\endgroup$
    – uhoh
    Commented Nov 6, 2018 at 9:31
  • 1
    $\begingroup$ @uhoh Yup, that's the clip. Look in the background of the first parabola--there's a guy standing there with a headset on. He's not moving, he must be standing upright to the local acceleration--and he's standing straight up. The acceleration is down through the floor, not tilted. (Everyone else is either down or moving around, you can't judge their vertical.) $\endgroup$
    – Loren Pechtel
    Commented Nov 6, 2018 at 9:54
2
$\begingroup$

Aircraft are not the only devices that can create partial gravity. A drop tower can also be used. Most of them only support freefall trajectories, but the Einstein-Elevator of the Hannover Institute of Technology can run trajectories at any acceleration between 0.1 and 1 G.

In addition to the main purpose of experiments under microgravity, other experiments with hypogravity in the range of 0-1 g and hypergravity in the range of 1-5 g are possible.

For the test execution, the test setup is placed in a vertically movable vacuum chamber, called the gondola, which is made of carbon fiber reinforced plastic... A linear motor is used to accelerate these two components through a brief acceleration phase...

For hypogravity tests, the linear motor stays attached to the experiment gondola and controls its acceleration curve.

Payload of this tower is 1000 kg.

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ Wow, microgravity as low as <10-6 g, very fancy! $\endgroup$
    – uhoh
    Commented Nov 6, 2018 at 13:48
1
$\begingroup$

There are a couple ways I'd like to describe the answer to this.

An appeal to intuition

The parabolic arc of the aircraft only changes the apparent gravity felt by someone falling onto it, not the trajectory itself. Essentially you feel the force you would on a Martian-G aircraft that is flying the same attitude profile.

If you were sitting in a seat on that aircraft, you'd feel pushed into the seat back as the aircraft climbed and pushed into the seat belts as the aircraft dove.

To alleviate those forces, you'd need the aircraft to decelerate on the climb and accelerate in the dive. That acceleration/deceleration profile would be a function of the attitude during the maneuver.

It's not totally clear to me what that implies about the practicality of the maneuver; you'd need to start on the high side, probably, to minimize deceleration time, but also need an aircraft that could spend that remaining time accelerating in the dive without exceeding it's V_ne.

The way they taught me in engineering school

A free-body diagram (which I may draw later) will show that the normal force provided by the aircraft's floor will necessarily have a component pointing "out of" the parabolic arc, i.e. a backward-pointing force on the upward side of the arc, and a forward-pointing force on the downward side of the arc.

The implied answer is the same, though. The aircraft needs to be decelerating for the sum of apparent forces to be perpendicular to the floor during the climb, and accelerating for the sum of apparent forces to be perpendicular to the floor during the descent. (...this part of the answer could admittedly be improved, but it's late where I am right now.)

Improve this answer
$\endgroup$
0

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.