Why do malfunctioning satellites come back to Earth?

Question

In school, I learned that if a satellite moves at a speed $\sqrt{gR}$ (the square root of the product of the acceleration due to gravity and radius of the Earth), then it will remain in Earth's orbit. As we know, there are no opposing forces to a satellite, so its speed will stay constant, as per Newton's 3rd law of motion.

But today I saw this news article, saying that a European satellite is falling back to Earth. Why will the satellite fall back to Earth, and why it will move around in space? If the satellite is falling due to Earth's gravitational force, then why it did not fall when it was in working condition?

Answer 1

In short, the European Space Agency's GOCE (Gravity Field and Steady-State Ocean Circulation Explorer) satellite was last orbited in extremely low altitude elliptical Low Earth Orbit (LEO) with perigee of 195 kilometres (121 mi) and apogee of 201 kilometres (125 mi) altitude above the sea-level. At that altitude, there is still some atmospheric pressure, albeit extremely tenuous, but given the satellite's velocity and surface area still enough to result in meaningful drag and decreasing its orbital velocity. Additionally, the Sun's increased activity during the last weeks resulted in increased particle density solar winds reaching the Earth through (flares and coronal mass ejections) and have somewhat increased the satellites rate of orbital decay due to increased atmospheric drag.

   

   ^{Artist's depiction of the European Space Agency's Gravity Field and Steady-State Ocean Circulation Explorer satellite in orbit.}

GOCE is even an aerodynamically streamlined satellite to reduce that drag to minimum possible and require less of its propellants to constantly do orbital reboosts to increase its velocity and with it altitude, but since it ran out of its propellant (GOCE used Xenon ion propulsion thrusters powered by 1,300 W fixed solar panels), its orbit will slowly decay due to atmospheric drag and it will end its life falling towards the lower and denser atmosphere where it will presumably completely burn up.

These orbital reboosts are a standard procedure also for the International Space Station (ISS) that currently orbits at the altitude of 230 miles (370 km), and each new visiting spacecraft (such as today's Soyuz TMA-11M that was just launched from Baikonur Cosmodrome) lends it a hand with its own thrusters after the spacecraft has successfully docked with the station.

Some values and a graph of atmospheric pressure at LEO altitudes and beyond are listed on the Wikipedia page on International Standard Atmosphere. Quoting a Cornell University document on Simultaneous Orbit and Atmospheric Density Estimation for a Satellite Constellation (PDF):

For many satellites in low earth orbit (LEO), the largest dynamic model uncertainty stems from atmospheric drag. Acceleration due to atmospheric drag $a_D$ is related to atmospheric density $p$ by the equation:

$$a_D = - {1\over2}({C_D{A_v(t)\over{m_s}}})\ {pv_r}^2e_v$$

where $C_D$ is a drag coefficient, $A_v(t)$ is the cross-sectional area of the satellite in the direction of travel, $m_s$ is the total spacecraft mass, $v_r$ is the velocity magnitude relative to the ambient atmosphere, and $e_v$ is a unit vector in the relative velocity direction. Uncertainty enters this equation in three ways. First, the scalar product $({C_D{A\over{m_s}}})$, known as the inverse ballistic coefficient, is generally uncertain and may be time-varying. Second, the relative velocity may be uncertain, either because it has not yet been estimated accurately or because the local wind does not rotate perfectly with the Earth. Finally, atmospheric density is very difficult to determine. Three basic paradigms exist for dealing with drag uncertainty: It can be modeled, measured directly or indirectly, or estimated in conjunction with satellite orbits.

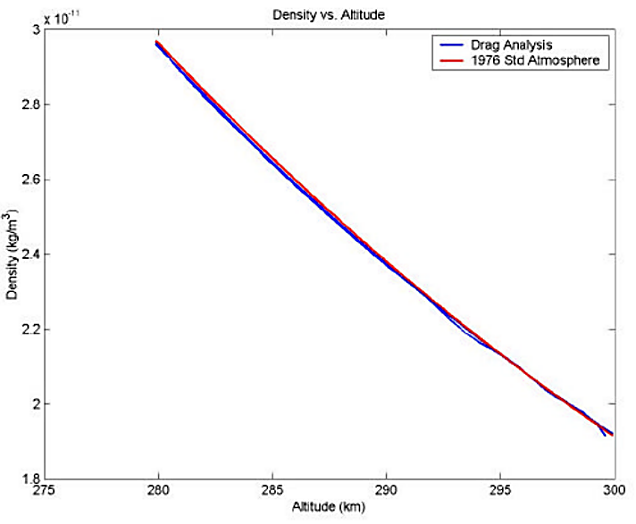
And this is a calculated density and reference density vs. altitude graph for Circular Equatorial Orbit (Direct PDF download: Determination of Atmospheric Density in Low-Earth Orbit Using GPS Data, United States Naval Academy, John L. Young III):

    

Satellites orbited above any atmospheric drag like the ones in Geosynchronous (GSO) or Geostationary Orbit (GEO), usually communications satellites, however aren't disposed of by deorbiting them into the atmosphere where they could burn, but are placed in a graveyard or junk disposal orbit a few hundred kilometers above the GEO belt.

Answer 2

It is incorrect that there are no opposing forces on an Earth-orbiting satellite. In Low Earth Orbit, drag is a significant factor that will cause most satellites to reenter within years to decades of launch. In fact, LEO satellites have a 25-year maximum life requirement. In order to obtain US government permission to launch a satellite the operator must conduct an analysis to show the satellite is expected to re-enter with high probability within 25 years.

The exact altitude at which re-entry is certain is hard to state because it is a function of many variables (hence the need for an analysis and not a strict altitude cut-off). The most important variable is when during the approximately 11-year solar cycle the satellite will operate. The solar cycle is correlated to changes in high-altitude atmospheric density, which changes the drag forces on a satellite by more than an order of magnitude.

Increased solar heating makes the thermosphere puff out as denser layers from lower altitudes expand upward. The density of the thermosphere can soar by a factor of 50 during solar maximum, with a commensurate increase in atmospheric drag on satellites.

Solar pressure can also serve to de-orbit a satellite; however, the net effects are less obvious because solar pressure is applied in the direction of incident sunlight and thus for an arbitrary orbit does not have a deterministic direction with respect to the spacecraft's velocity vector. In contrast, drag always serves to slow down a satellite and thus lower its orbit. Furthermore, solar pressure is typically a much smaller force than drag: during low solar cycles solar pressure is typically on par with drag, whereas during high solar cycles drag is a factor of 10 or more higher.

That said, anything below 500 km is definitely coming home; it's only a matter of when. Above that and it could be several decades before re-entry. Remember that because the density profile as a function of altitude is roughly exponential, re-entry happens in the same way a man goes bankrupt:

Two ways. Gradually, then suddenly.

Geosynchronous satellites, which operate near 42,000 km have effectively zero drag. They will not re-enter in any time horizon worth considering. That is why GEO birds are supposed to be placed in a graveyard orbit when they have reached end-of-life but before they are no longer capable of responding to ground commands. GPS satellites operate at half-GEO; they're not coming home either.

Lastly, the rationale for a LEO orbit could be an entire post, but the short answer is that many missions don't make sense at GEO. For example, it's hard to take high-resolution pictures of the Earth from that far away. Almost exclusively GEO satellites are used for telecommunications. LEO satellites are used for all sorts of other missions, from Earth remote sensing, to measuring Earth's magnetic field, to specialized science payloads.