5
$\begingroup$

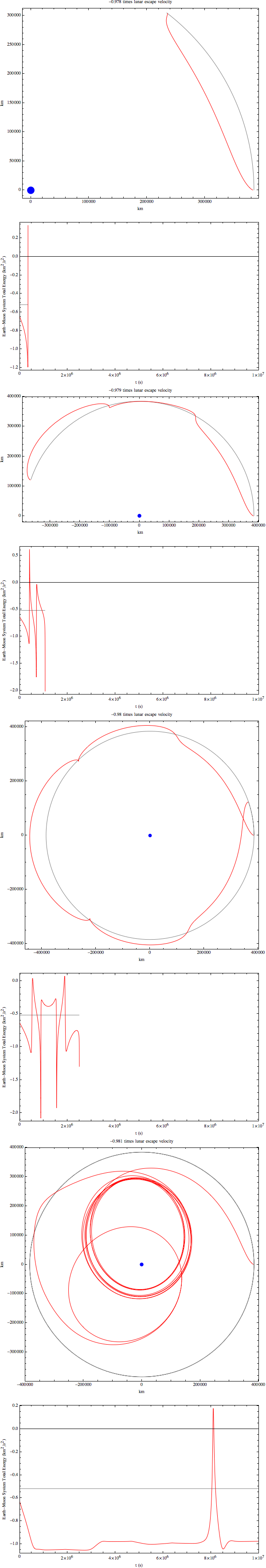
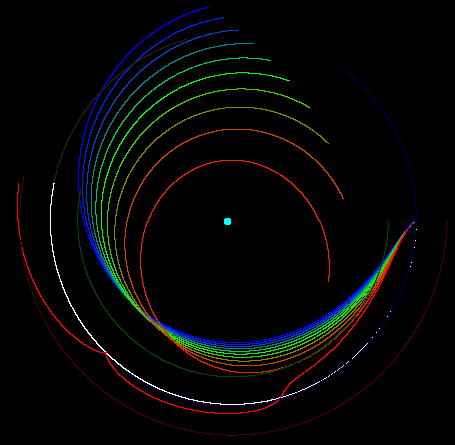
Let's say you are standing on the Moon, with the Earth directly above your head (the moon being tidally locked with the earth. At this point you and the Moon are sharing an orbit, both experiencing the same orbital elements.

You climb into a cannon that has just enough force for you to reach escape velocity (or, maybe more appropriately, leave the Moon's sphere of influence). Pull the trigger, and force is applied orthogonal to your velocity vector.

How does your new orbit differ from the Moon's? What orbital elements changed in this process? Would your orbital period relative to the moon decrease?

UPDATE

These answers are all really great, but I made things too complicated by involving the moon. Instead, more simply, how do orbital elements change when force is applied orthogonal to the velocity vector of an orbiting mass? Think about a satellite in GSO.

Improve this question
$\endgroup$
6
  • 1
    $\begingroup$ A nit, but you don't have quite the orbital elements of the Moon. In fact your trajectory is not an orbit. You are one lunar radius from the center of the Moon, and you are rotating counter to the orbital motion of the Moon to keep you looking straight up at Earth. So your path is quite close to an orbit whose semi-major axis is one lunar radius less than the Moon's semi-major axis, but the period of your path is that of the Moon's. Your "orbit" doesn't match your orbit period. Your path is not an orbit. $\endgroup$
    – Mark Adler
    Commented Mar 15, 2014 at 14:53
  • $\begingroup$ The title of the thread asks a rather different question than does the question itself. I suggest re-asking the question raised in the title. Get rid of the Moon. It's a complicating factor. Ask instead what happens when an orbiting spacecraft fires orthogonal to its velocity vector. $\endgroup$
    – David Hammen
    Commented Mar 17, 2014 at 19:16
  • $\begingroup$ I agree. The question became more complicated then intended, but the answers were creative enough that I really enjoyed reading the answers. $\endgroup$
    – Stu
    Commented Mar 17, 2014 at 19:38
  • $\begingroup$ Please ask a new question instead of updating this one. $\endgroup$
    – Mark Adler
    Commented Mar 17, 2014 at 21:06
  • $\begingroup$ The new question is very different from the original one. Now I am regretting investing time and energy running an n-body sim. It's also vague -- there are a multitude of directions orthogonal to the velocity vector. I'll assume you mean straight down. And what is the shape of the orbit? I'll assume you mean circular. It would change the flight path angle. The new perigee would be less than 180 degrees ahead. It would also increase the semi-major axis and the period some, depending on how much force is applied. $\endgroup$
    – HopDavid
    Commented Apr 10, 2014 at 5:35

3 Answers 3

Reset to default
6
$\begingroup$

Would your orbital period relative to the moon decrease?

Somewhat surprisingly, yes. You will end up in a slightly smaller orbit about the Earth with a slightly smaller period. That's assuming that (1) a patched conic is a valid approach (it probably isn't in this case), and (2) I've done my math right.

I'll assume the Moon is in a circular orbit at 384500 km, moving at 1.022 km/s relative to the Earth. I'll assume you fire the canon at the sub-Earth point at a velocity of 2.375 km/s, aiming straight towards the Earth.

Using a patched conic approximation, you are on a radial parabolic trajectory. It will take 1.315 days or 0.04814 sidereal months to reach a distance of 66000 km from the center of the Moon (that's the radius of Moon's sphere of influence; c.f. the 60000 km radius of the Moon's Hill sphere), at which point that initial 2.375 km/s velocity will have dropped to 0.3854 km/s.

Converting to geocentric coordinates, you will be 322100 km from the center of the Earth and moving at a speed of 1.195 km/s relative to the Earth.

Using the vis-viva equation, this means you will be in an elliptical orbit about the Earth with a semi-major axis of 380700 km. That orbit has a period of 27.056 days.

You won't stay in that orbit for long. The Moon is going to perturb that orbit, big time.

Improve this answer
$\endgroup$
18
  • 1
    $\begingroup$ +1 I didn't do maths (lack of time) but are you sure that the orbital period changes much? Otherwise, it sounds about right, you'd increase eccentricity and decrease periapsis at one end and increase apoapsis at the other. It should have a significant precession tho, I imagine it would eventually settle in a rosette orbit around Earth with translunar apoapsis? $\endgroup$
    – TildalWave
    Commented Mar 14, 2014 at 17:32
  • $\begingroup$ Nevermind, didn't see all the links to WolframAlpha. Indeed, it's rather surprising. :) $\endgroup$
    – TildalWave
    Commented Mar 14, 2014 at 17:44
  • $\begingroup$ Yes, it is surprising. My initial guess was that the period would increase because firing the cannon adds energy. The Moon steals most of that energy as the projectile drops Earthward. Note that if the Moon wasn't there, firing orthogonally at 2.38 km/s to a circular orbit at 384500 km would result in a hyperbolic trajectory. Earth escape velocity at that distance is only 1.44 km/s. $\endgroup$
    – David Hammen
    Commented Mar 14, 2014 at 17:52
  • 1
    $\begingroup$ My initial guess was that it doesn't change the orbital period / semi-major axis and merely increases orbital eccentricity, since the new vector towards the focus is orthogonal to the orbital velocity vector (so non-canceling). It does add energy, but also makes the projectile come closer to Earth's SOI, so I assumed those more or less cancel each other out... eventually. Now I wonder how many orbits till it hits the Moon again... If (when) I'll find the time, I'll fire up GMAT and see what it comes out with. Should be fun. :)) $\endgroup$
    – TildalWave
    Commented Mar 14, 2014 at 17:56
  • 1
    $\begingroup$ That boosting orthogonally to a circular orbit doesn't change the orbital period / semi-major axis by much at all is a reasonable approximation if Δv/v is much less than 1. Here, Δv/v is 2.38. That approximation doesn't hold in this case. $\endgroup$
    – David Hammen
    Commented Mar 14, 2014 at 18:05
5
$\begingroup$

"Your new orbit" isn't simply a two-body orbit about the Earth that you might be thinking of, since the Moon is still there after you (barely) escape. In the long term it will be a complicated trajectory that will eventually result in you impacting the Moon, escaping the Earth-Moon system, or possibly even impacting the Earth. So the concept of orbital elements is not terribly useful.

However, you can imagine an effective orbital period when you are orbiting the Earth but are far from the Moon. That is related to your total specific energy, where if that is less than the Moon's, your orbital period is less than the Moon's, and vice-versa.

Here are some examples of your trajectory where you are launched at or a little above the lunar escape velocity. (The gray circle is the Moon's orbit. The blue disk in the middle is the Earth to-scale.)

Shown under each trajectory is your specific energy in the Earth-Moon system. You can see that initially it is always less than the Moon's (the gray line), and so your "orbital period" is shorter than the Moon's. But it can go above the Moon's after a few lunar flybys, and usually does. Where the plot doesn't go all the way across, it impacted the Moon where it ended. In one case, you can see that you escape the Earth-Moon system completely.

plots of trajectories and specific energy

Here are some interesting borderline cases. Below 0.978 they all do the same thing -- immediately reimpact.

plots of borderline cases

Improve this answer
$\endgroup$
14
  • $\begingroup$ Try a few runs with slightly less than escape velocity -- e.g., 99% of escape velocity (-0.99 times escape per the titles of your graphs), maybe even down to 98.5% of escape velocity. Much less than that and the projectile just falls back toward the Moon. $\endgroup$
    – David Hammen
    Commented Mar 15, 2014 at 20:22
  • $\begingroup$ Yes, I did those and it behaves much the same down around $-0.99$. I've also run them around $+1$, where the code automatically starts instead at the far point of the Moon and shoots straight up. $\endgroup$
    – Mark Adler
    Commented Mar 15, 2014 at 20:30
  • $\begingroup$ Try lower even lower. Things get weird when the projectile has barely enough energy to escape the Moon's SOI (~66000 km). $\endgroup$
    – David Hammen
    Commented Mar 15, 2014 at 20:42
  • $\begingroup$ To leave the moon's sphere of influence it suffices to get the apolune close to EML1. From the moon's near point straight up, that takes about 98% escape velocity. $\endgroup$
    – HopDavid
    Commented Mar 15, 2014 at 20:43
  • $\begingroup$ I don't trust the results too low, since I don't have detection of impact in the code. I did run cases there and lower still, and they are quite wonky. (Technical term.) However there were probably impacts with the Moon. I might look at putting in such detection ... $\endgroup$
    – Mark Adler
    Commented Mar 15, 2014 at 21:14
2
$\begingroup$

3 body sim

Here's a screen capture from an orbital sim for a variety of pellets launched straight up from the moon's near point. The red #1 pellet is just under escape velocity. The blue #11 is a hair over. Tidalwave, The blue dot is the earth. The white orbit is the moon. I placed darker colored pellets at EML1, EML2 as well as EML4 and EML5.

The rainbow pellets are from what I call orbital shotgun. The pellets velocity and direction vary from one extreme (pellet one) to another (Pellet 11). Here is the page I used to do this sim: Orbital Shotgun Making smaller time increments makes the model more accurate but slower. I had to make time increments pretty small for this sim. It can be seen that pellet 1 doesn't quite make it past EML1 and falls back to the moon. The rest of the pellets escape the moon's sphere of influence and fall into earth centric orbits

In general pellets the pellets will hit the earth, moon, or be completely ejected. Just a tiny variation in velocity can make a huge difference in outcome. In this diagram the fastest pellet varies from the slowest by .6 meters/second: enter image description here

Improve this answer
$\endgroup$
0

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.