tl;dr: It may depend a lot on their proximity to a blast at the time. For the lingering radioactivity afterward, that's all confined to the atmosphere and the ground and water; add to that the effects of nuclear winter, the pair on the ISS may live longer in space than most people on the Earth!
I don't think much prompt radiation (gamma rays) makes it through earth's atmosphere, which has an areal mass density equivalent to 76 centimeters of mercury.
Even if it did they're still a minimum of 400 km away from the blast and $1/r^2$ applies.
There is still the EMP to worry about, though $1/r^2$ still applies.
As explained in the link above and here if a deliberate EMP device was used, the burst would be at high altitude in the atmosphere or in space. The prompt gamma ray pulse propagates to the thicker atmosphere where the electrons in the atoms of the atmosphere all jump at once, producing a lower frequency electromagnetic transient. That will propagate back up to the ISS' 400 km and if it's passing over the footprint of the EMP device it may get zapped†.
Once zapped, critical electronics may be fried, and while some systems may have backup circuit boards I don't know if they are stored in EMP-proof bags.
Without critical electronics they may not be able to point the solar panels, or even orient the ISS' attitude properly. With those lost, they would have a far lower average solar power. It's possible that they could conserve power enough to survive though.
This begs several new questions:
- What is the minimum average electrical power on which two people could survive on the ISS (air, water, etc.)
- If the ISS assumed the worst possible attitude (highest drag orientation) how long would it take to re-enter the atmosphere, assuming a) a quiet Sun, and b) an active Sun?
Hopefully the ISS' metal construction will provide substantial electromagnetic shielding against the EMP. If it were a perfect faraday cage it would, but most makeshift faraday cages aren't perfect and there's all those important antennas and anti-charging gizmos on the outside.
†Of course in the unlikely event that the ISS is passing over the center of an EMP device detonation it could end up only kilometers away from the detonation itself! Then there's primary radiation to worry about as well as blindness for anyone looking out a window in the wrong direction at the time.
From and here
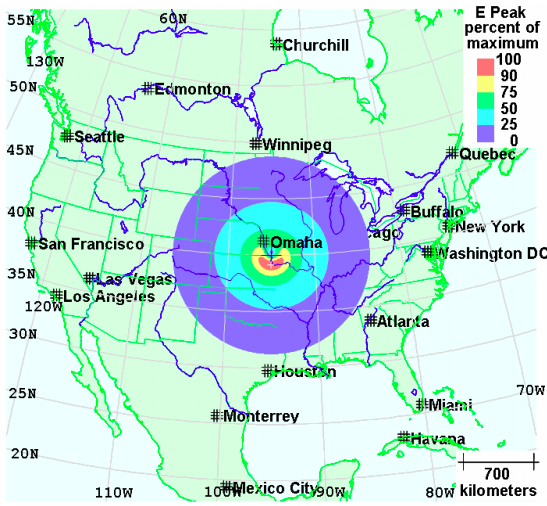
Figure 2-3. A sample E1 HEMP “smile” diagram. Such diagrams show contours of peak incident E levels, for a burst height of 75 km in this example. Here contour levels are shown as fractions of the biggest peak level (which is to the south of the burst point for this northern latitude burst). Over the exposed region, the average value is 10.4% of the maximum (12.4% if we use the square root of the average square of the peak instead).
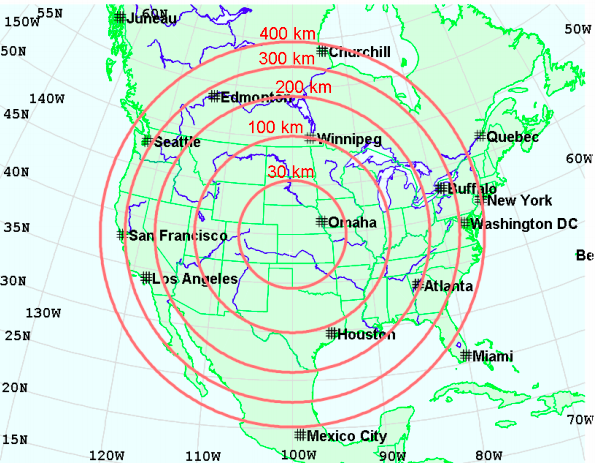
Figure 2-9. Samples of E1 HEMP exposed regions for several heights. The red circles show the exposed regions for the given burst heights, for a nuclear burst over the central U.S.

