Delta-v penalty for stopping in a distant Earth orbit versus using atmospheric reentry for braking and returning to Earth from Mars?

Question

Comments below this answer to What precautions are planned to prevent an accident or anomaly from releasing Martians into Earth's environment? say:

Seems to me like a high earth orbit lab might be a good idea.

and

t’s too expensive in delta v ...

For background one can for example read about the Asteroid Redirect Mission and its proposed distant retrograde obit or DRO in answers to:

• What ever happened to the Asteroid Redirect Mission?
• Could "Asteroid Redirect" make the moon more dangerous for astronauts for a long time?
• How much does the tidal effect affect satellites in a retrograde orbit?

Question: For a sample return mission launched from Mars, what would be the delta-v penalty for stopping in a distant Earth orbit (retrograde or prograde) versus using atmospheric reentry for braking?

Answer 1

Running the numbers...

As far as Delta-V goes, Directly Braking into a high circular orbit is the most expensive, Aerobraking into a Hohmann transfer to your destination is less expensive. Aerobraking and Parachute landing is the least expensive.

We'll concentrate on calculating just the Delta-V that the spacecraft uses while flying by/orbiting Earth. The earlier part of the trip is identical in all cases. We'll assume a Hohmann Transfer Orbit returning from Mars during a transfer window. We'll also make a number of simplifying assumptions:

• Earth and Mars are in circular, ecliptic orbits.
• The destination Earth Orbit is in the Ecliptic Plane.
• The Spacecraft is capable of instantaneous impulses.
• The Spacecraft has a heat shield and variable-geometry flaps to increase or decrease the power of aerobraking, so regardless of the speed reduction necessary, it can aerobrake at our chosen altitude.
• The Moon minds its own business and stays out of our way.

Returning from Mars:

We'll need the following parameters:

• the semi-major axis of Mars' orbit, $a_M = 2.27 \times10^{11}\mathrm{m}$
• The semi-major axis of Earth's orbit, $a_E = 1.47 \times 10^{11}\mathrm{m}$
• The Gravitational Parameter of the Sun, $\mu_S =1.33 \times 10^{20}\mathrm{m^3/s^2} $
• The Gravitational Parameter of Earth, $\mu_E =3.99 \times 10^{14}\mathrm{m^3/s^2} $
• Earth's average Orbital Velocity, $v_E=2.98 \times 10^4 \mathrm{m/s}$
• Radius of the Earth, $r_E = 6.37 \times 10^6 \mathrm{m}$

And from there calculate the Hohmann Transfer semimajor axis: $$a_h = \frac{a_E + a_M}{2} =1.87 \times 10^{11}\mathrm{m} $$

And use the Vis-Viva equation to determine the spacecraft's velocity at Hohmann perihelion: $$v_{hp} = \sqrt{\mu_S\left(\frac{2}{a_E}-\frac{1}{a_h}\right)}=3.31 \times 10^4 \mathrm{m/s} $$

Since on the ideal Hohmann Transfer from Mars to Eath, the spacecraft is moving in the same direction and catches up to Earth from behind, we can subtract to get the Earth-relative velocity on a distant approach: $$v_{E\infty}=v_hp - v_E = 3.34\times10^3 \mathrm{m/s}$$

Earth-Relative Hyperbolic Flyby:

For the hyperbolic trajectory past Earth, we can determine the Specific Orbital Energy of the incoming spacecraft, which will remain constant relative to Earth during the flyby: $$\epsilon = \frac{v_{E\infty}^2}{2} = 5.58 \times10^6 \mathrm{J/kg}$$ Calculate the Semimajor Axis of the Hyperbolic flyby: $$a_{hyp}=-\frac{\mu_E}{2\epsilon}=-3.58\times10^7\mathrm{m}$$

And back to the vis-viva equation to get the velocity on the incoming hyperbola as a function of radial distance from earth $r$ $$v_{hyp}=\sqrt{\mu_E\left(\frac{2}{r}-\frac{1}{a_{hyp}}\right)}$$

As noted in my comment on Polygnome's answer, this works out to $11.4\mathrm{km/s}$ at an altitude of about $340 \mathrm{km}$ over Earth.

Option One: Direct Injection into Circular Earth Orbit

So now we can calculate the delta-V necessary to come in directly from interplanetary space, and brake into a circular orbit at our flyby's chosen periapsis, by comparing with the Circular Orbit Velocity for the same distance: $$v_{circ}=\sqrt{\frac{\mu_E}{r}}$$

And the $\Delta v$ is the difference between the two. $$\Delta v_{direct} =v_{hyp} - v_{circ} = \sqrt{\mu_E\left(\frac{2}{r}-\frac{1}{a_{hyp}}\right)} - \sqrt{\frac{\mu_E}{r}}$$

Some interesting notes here: It appears that the Delta-V to brake to a circular orbit minimizes when the velocity at the hyperbolic periapsis is twice the circular orbit velocity. For these chosen parameters. it looks like this happens at a radius of about $71500 \mathrm{km}$, or an altitude above Earth of about $65100 \mathrm{km}$, with a required delta-v of about $2360 \mathrm{m/s}$.

Option Two: Aerobraking into Hohmann Transfer to Destination Earth Orbit

Let's aerobrake into an elliptical orbit, and circularize at our new apoapsis instead. Putting numbers on the aerobraking altitude gets very tricky, and I don't have the expertise to put together an atmospheric model for the required aerobraking altitude. Looking at the HITEN spacecraft, it performed Aerobraking at an altitude of 125 km over the pacific, so let's use that.

Aerobraking radial distance is thus: $$r_{aero} = r_E + 1.25 \times 10^5 \mathrm{m} = 6.50 \times 10^6 \mathrm{m}$$

And we can use that as the lower radius to calculate the calculate the Hohmann transfer delta-V for circularization at the destination orbit radius $r$

$$\Delta v_{aero}=\sqrt{\frac{\mu_E}{r}}\left(1-\sqrt{\frac{2r_{aero}}{r_{aero}+r}}\right)$$

Option 3: Aerobrake and Land with parachutes.

You get all your deceleration delta-v from hitting the atmosphere at 11.7 km/s. As such, the extra required delta-V is $$\Delta v_{smackdown} = 0\mathrm{m/s}$$

Delta-V comparison on Mars Hohmann Return to Orbit - Direct Injection vs Aerobrake Hohmann (GeoGebra Graph)

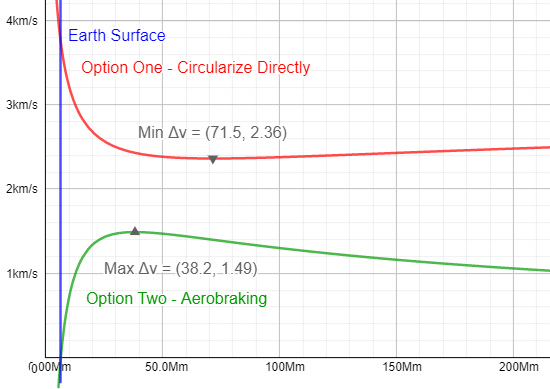
Horizontal axis is circular orbit radius from Earth in thousands of kilometers (Mm). Vertical axis is required $\Delta v$ in kilometers per second. Blue line indicates the surface of the Earth.

The Red line is Option 1: the delta-V requirements to directly brake into the desired circular orbit over Earth from the interplanetary Hohmann trajectory from Mars. As the radius of the destination orbit rises, the required delta-V decreases, until it reaches the orbital distance where the periapsis velocity of the flyby would be twice that of the circular orbit velocity, then rises again to asymptotically approach $v_{E\infty}$. For the parameters chosen, this minimum occurs at an orbital radius of about $71500 \mathrm{km}$, with a delta-V requirment of about $2360 \mathrm{m/s}$.

The Green Line is option 2: Delta-V requirements for Aerobraking at 125 km altitude into a Hohmann trajectory, circularising at the destination altitude. For the parameters chosen, it starts at 0 for the 125-km-altitude orbit, rises to a peak, and then asymptotically decreases to 0. The maximum occurs at an orbital radius of about $38200 \mathrm{km}$, with a delta-V requirement of about $1490 \mathrm{m/s}$

Conclusion: If you can swing it, and your desired destination is an Earth Orbit, aerobraking into the Hohmann is the way to go, especially if you're doing it in Kerbal Space Program, where heat shielding and delta-V are cheap, and you can quicksave and quickrestore to avoid potential tragedy and embarrassment.

Answer 2

Mars return velocity is about 11.4km/s [1] (NASA gives 11.56km/s [2]). LEO orbital velocity is about 7.8km/s. Bringing enough fuel to do this (3.6 km/s) would increase the size of the rocket needed for liftoff manifold. Each kg of payload exponentially increases the size of the LV. And you need the engine for the burn, and need to store the fuel over extremely log periods.

You need a heat shield for return to Earth anyways. Lunar return is about 11km/s. Designing a heat shield for 11.4km/s instead of 11km/s is by far easier than bringing additional fuel for 3.6km/s dv at the end of the mission, in the last stage.