Can't sleep so...
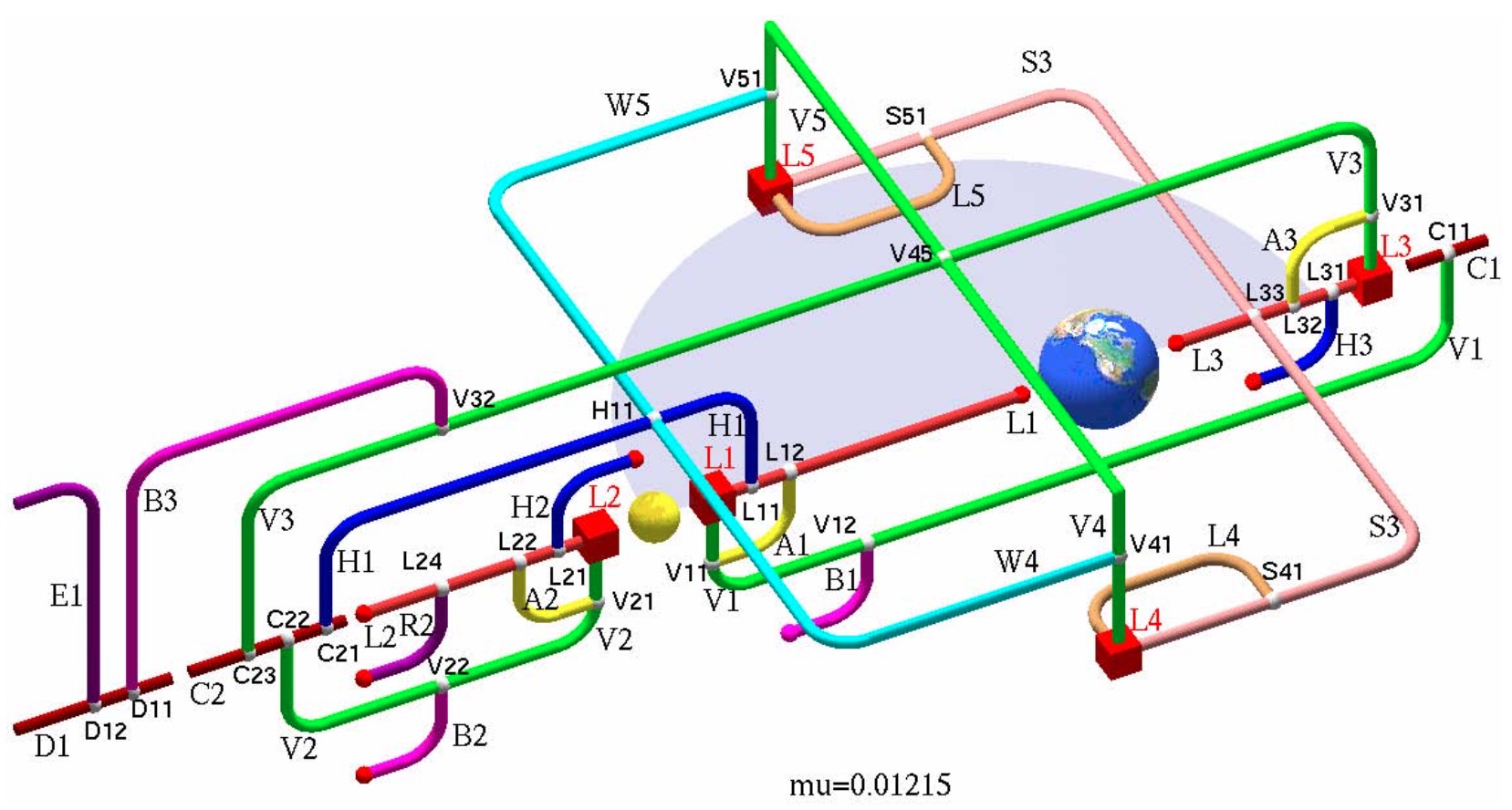
(Cropped and annotated from) Fig. 3. Bifurcation diagram for the Earth–Moon system (μ = 0.01215), showing families of periodic orbits that emanate from the libration points and from subsequent branch points. The red cubes are the libration points. Small white spheres denote branch points, and small dark-red spheres denote collision orbits. The planar families C1, C2, and D1, are only partially represented; in particular, the fact that D1 arises from C1 via a period-doubling bifurcation is not indicated in the diagram. A glossary of the notation used is given in Table 1.
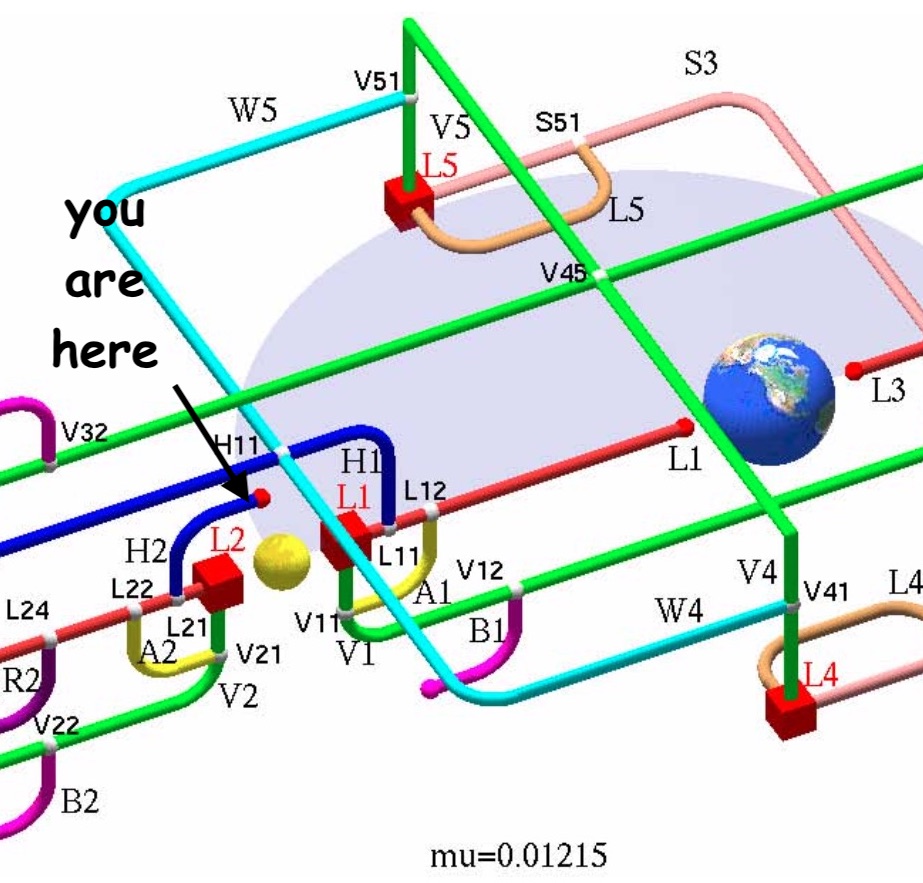
I've added an arrow pointing to the H2 branch near the end furthest from Earth-Moon L2 near the 'small dark-red sphere' which denotes the 'collision orbit' with the Moon.
In this paper the $H_{i=1, 2, 3}$ designates the "conventional" halo orbits we all know and love, associated with the three collinear Lagrange points L1, L2 and L3. In this paper's schemes for naming all these crazy orbits $H_i$ seems to be for "halo" and $L_i$ for either "Lyapunov" ($i=1, 2, 3$) or "Long" ($i=4, 5$).
In other papers these same halo orbits are often labeled as $L_i, i=1, 2, 3$ See for example:
Basically our friendly neighborhood halo orbits that we think of as giant slightly flattened but mostly round and titled are from the chunk of orbits near the middle of the mathematical family, and as you look to the extreme end closest to the Moon they get more and more thin and vertical, with perilunes that will eventually intersect the lunar surface (that small dark red sphere) if you take it to the extreme.


above: Screenshot from Near Rectilinear Halo Orbit Explained and Visualized click for larger below: "Fig. 14. The Northern Halo family H2 of the Earth–Moon system."
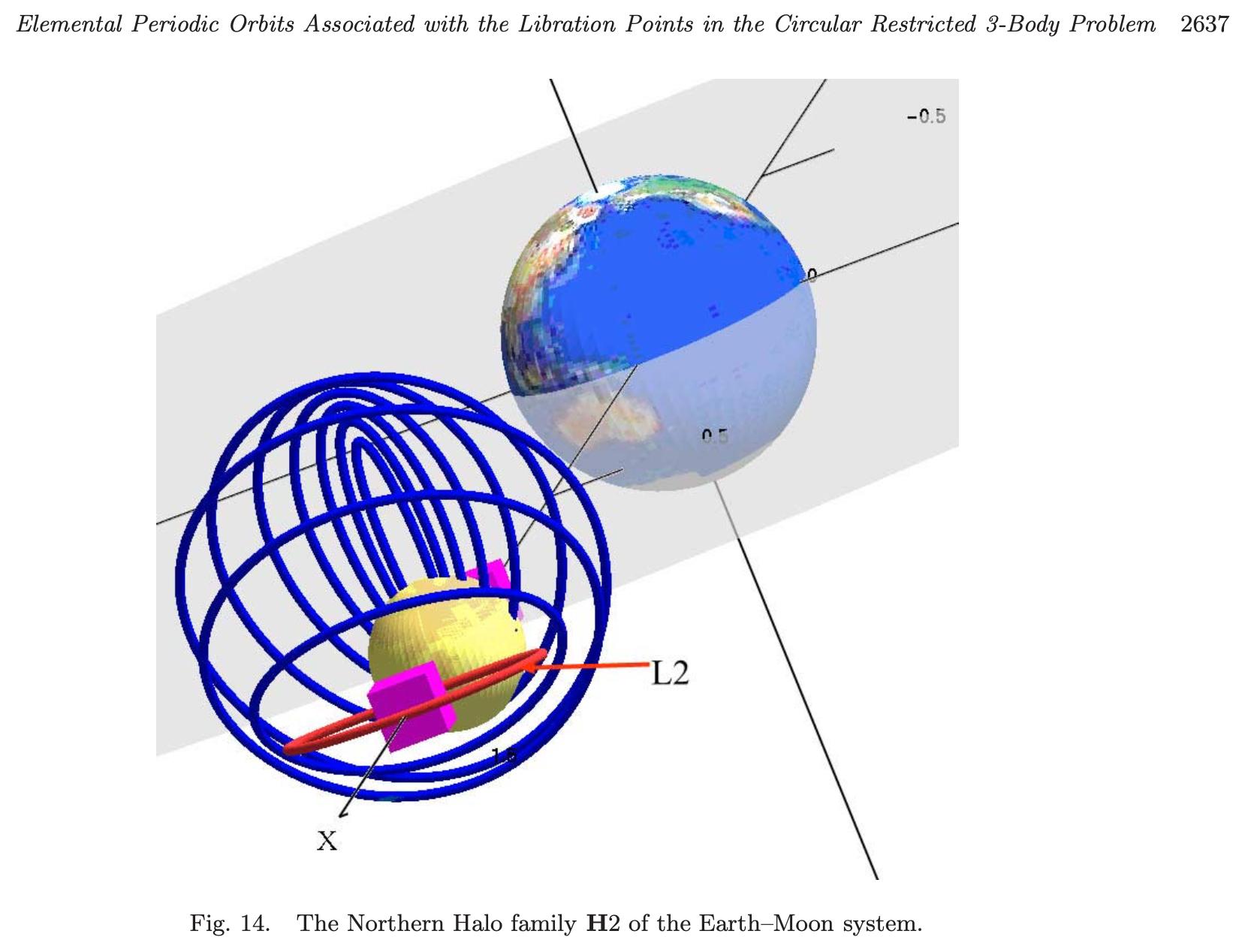
This figure is suboptimal and confusing, but it shows the same family of curves shown in the video. The red curve is the "beginning" of the family as a nearly flat orbit around L2, and the last blue vertical line that intersects the absurdly oversized rendering of the Moon is the L2 near rectilinear halo orbit of Artemis fame.
The label "L2" points to the horizontal beginning of the $L_2$ family of halo orbits, denoted in red.
The unlabeled close pink cube is the Earth-Moon L2 point, the other one partially obscured by the absurdly oversized Moon is the L1 point. The most vertical blue halo orbit is not behind the Moon near L1, it shoots straight down through the top of this oversized Moon to orbit its center.
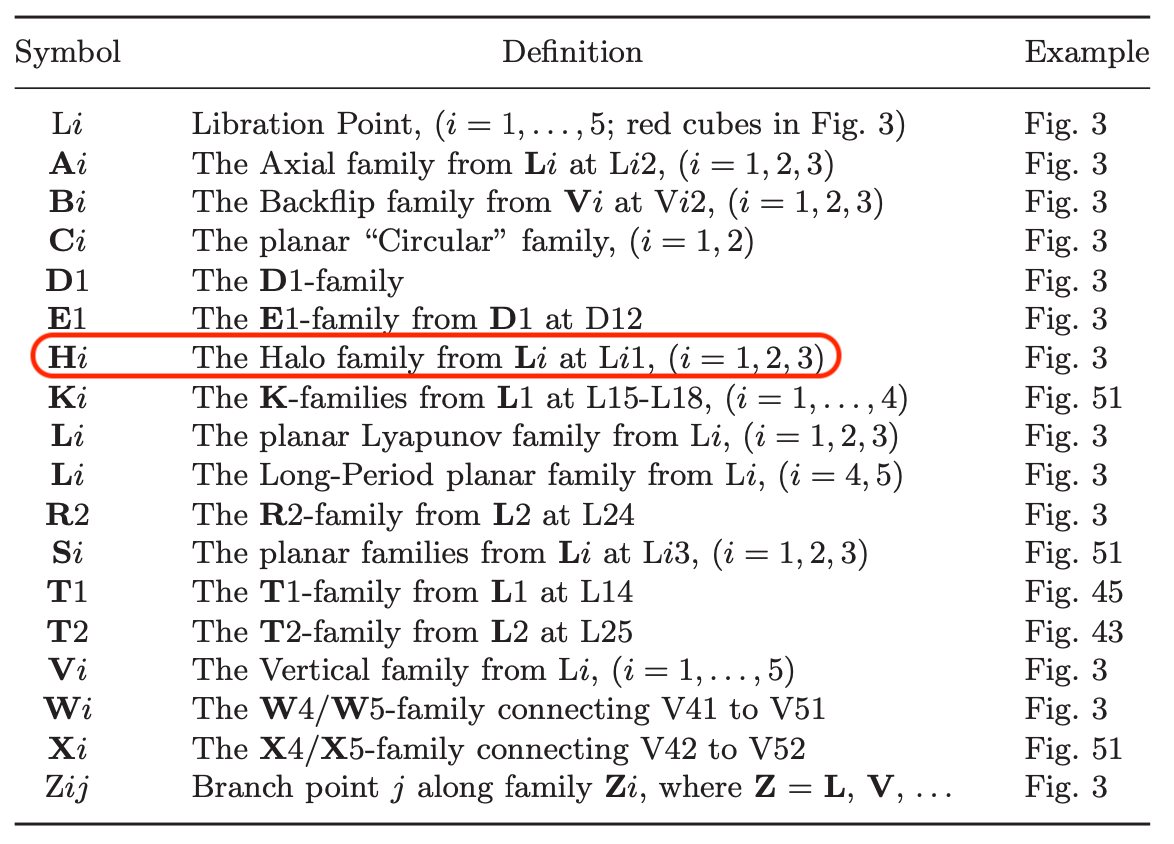
Table 1. Abbreviations used in this paper for orbit families and branch points. Note that orbit families are denoted with boldface capital letters, while we do not use boldface letters for libration points and branch points. We also note that not all of these items need to be present for any specific value of μ