Why was the 100m Green Bank dish needed together with DSN's 70m Goldstone dish to detect Chandrayaan-1 in lunar orbit?

Question

The Phys.org article New NASA radar technique finds lost lunar spacecraft describes the use of radar to relocate two spacecraft that were in orbit around the moon but who's orbit had not been actively tracked for a while. (See also the JPL version.)

"We have been able to detect NASA's Lunar Reconnaissance Orbiter [LRO] and the Indian Space Research Organization's Chandrayaan-1 spacecraft in lunar orbit with ground-based radar," said Marina Brozovic, a radar scientist at JPL and principal investigator for the test project. "Finding LRO was relatively easy, as we were working with the mission's navigators and had precise orbit data where it was located. Finding India's Chandrayaan-1 required a bit more detective work because the last contact with the spacecraft was in August of 2009." (emphasis added)

The article goes on to mention the use of powerful radar signals broadcast by the Deep Space Network's 70m Goldstone dish and received by the Green Bank 100m dish.

Question: Since the deep space network can perform ranging on spacecraft much farther away (tens of thousands of times farther than the moon) by itself, why was it necessary to use a non-colocated, non-DSN dish to receive signals in this case?

---

Later in the article:

Radar echoes from the spacecraft were obtained seven more times over three months and are in perfect agreement with the new orbital predictions. Some of the follow-up observations were done with the Arecibo Observatory in Puerto Rico, which has the most powerful astronomical radar system on Earth. Arecibo is operated by the National Science Foundation with funding from NASA's Planetary Defense Coordination Office for the radar capability.

...which suggests to me at least that the Arecibo dish could perform the measurement alone, without the need of a second dish.

---

Edit: In both cases a pseduo-random coded radio signals are broadcast at the satellite. For spacecraft in deep space it is received, amplified, and simultaneously and coherently rebroadcast back, while for radar ranging the return signal is passively reflected back. Here coherently means that the carrier signal for the transmission is carefully phase-locked with the incoming signal's carrier so that even though it is at a different frequency, the doppler shift can be recovered and analyzed much in the same way as in radar.

Due to the $1/r^4$ loss of signal intensity, radar detection of spacecraft can not be used much past a few lunar distances, so for much longer distances the amplification and coherent rebroadcast is required. From a signal processing point of view, delay and doppler information are recovered by correlating the received signal with the transmitted code. However from an operational point of view there may be substantial differences.

---

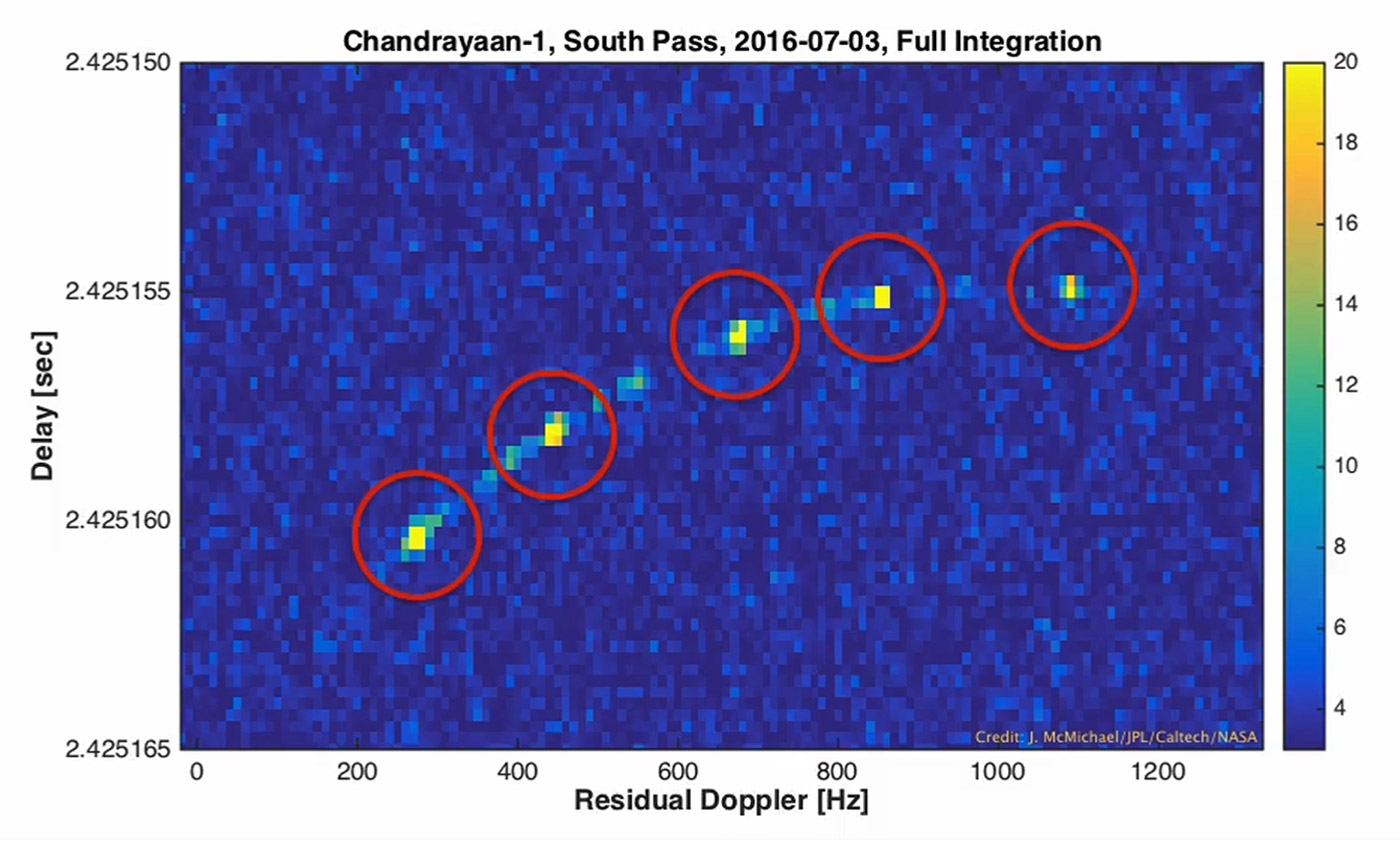
above: "Radar imagery acquired of the Chandrayaan-1 spacecraft as it flew over the moon's south pole on July 3, 2016. The imagery was acquired using NASA's 70-meter (230-foot) antenna at the Goldstone Deep Space Communications Complex in California. This is one of four detections of Chandrayaan-1 from that day." Credit: NASA/JPL-Caltech. From here

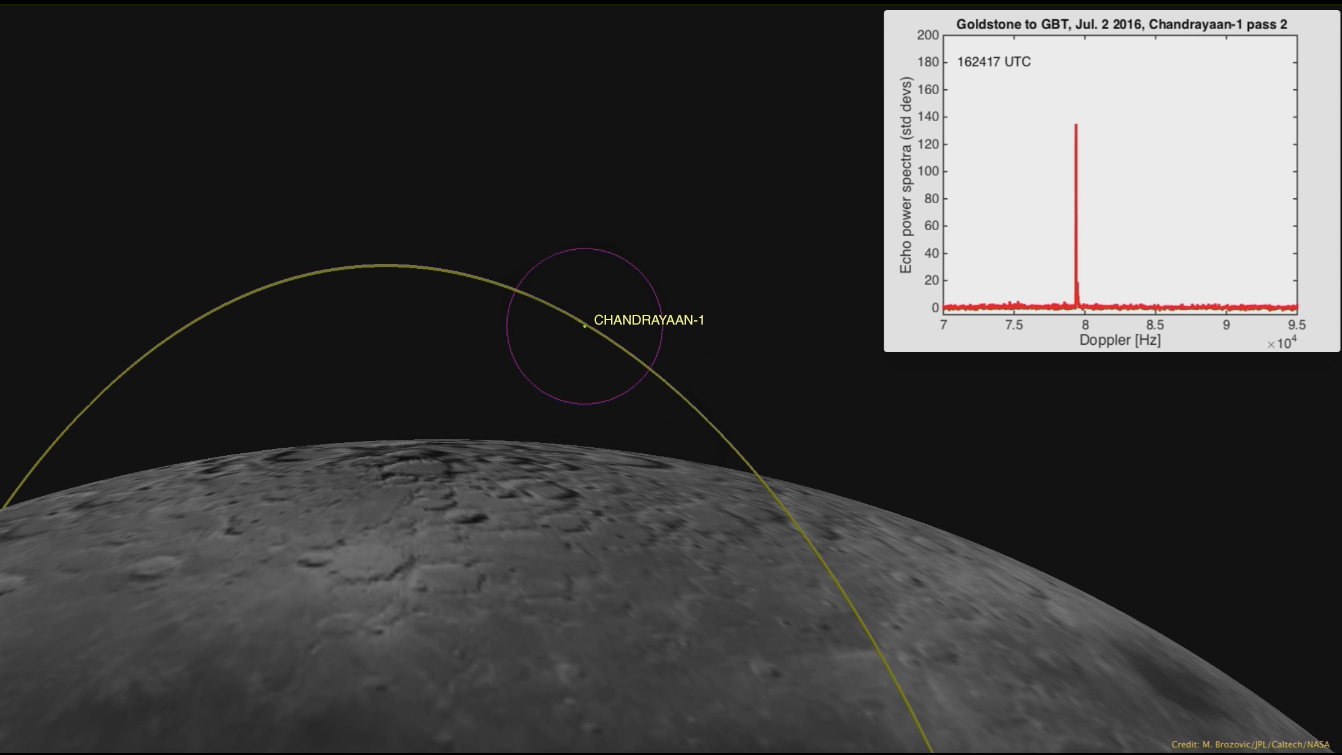
above: "This computer-generated image depicts the Chandrayaan-1's location at time it was detected by the Goldstone Solar System radar on July 2, 2016. The 120-mile (200-kilometer) wide purple circle represents the width of the Goldstone radar beam at lunar distance. The white box in the upper-right corner of the animation depicts the strength of echo. Inside the radar beam (purple circle), the echo from the spacecraft alternated between being very strong and very weak, as the radar beam scattered from the flat metal surfaces." Credit: NASA/JPL-Caltech. From here

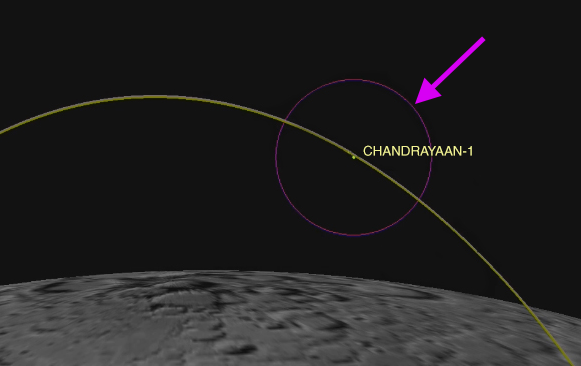
above: Cropped section of the previous figure, with an arrow added to draw attention to "The 120-mile (200-kilometer) wide purple circle represents the width of the Goldstone radar beam at lunar distance." Credit: NASA/JPL-Caltech. From here

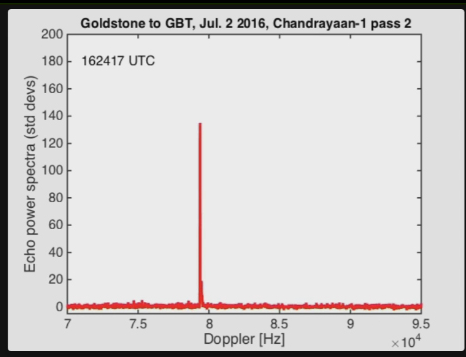
above: Cropped section of the previous figure to draw attention to "The white box in the upper-right corner of the animation depicts the strength of echo." Credit: NASA/JPL-Caltech. From here

Answer 1

The explanation has to do with the operation of the radar transmitters and the round trip light travel time.

It takes about 3 seconds for a radar pulse to travel from the Earth to the Moon and back. The planetary radar transmitters are high power; the Goldstone transmitter (at full strength) is 500 kW, the Arecibo transmitter is nearly 1000 kW. By contrast, the radar return is quite weak. It is difficult (essentially impossible) to design a system that could simultaneously transmit this much power and receive a weak echo. Only a small amount of the transmitted power would have to leak into the receiver for it to swamp the received echo.

Accordingly, if the configuration is monostatic (i.e., the same antenna transmits and receives), the transmitter has to be switched on and off. Switching the transmitter this rapidly can be damaging, either to the transmitter or to associated components.

By contrast, with a bistatic configuration (i.e., one transmitting antenna and one receiving antenna), the transmitter can be left on and long tracks can be obtained, which also can be valuable to build up signal-to-noise ratio.

Answer 2

I know nothing of the activity you're asking about, but I do know something about radar.

All the radar systems I've worked with used a single antenna to both transmit and receive. The power of the transmitter is very large compared to the return echo that needs to be received, and the very sensitive receiver needs to be disconnected from the antenna when the radar pulse is generated. The receiver is then connected to the antenna until the next pulse. This is performed by a duplexer, which in many radars consists of a T/R tube.

Switching from transmit to receive operation takes time. This effects the Minimum range of the radar. Perhaps this is why a different antenna/receiver was necessary.

Also, as Phiteros points out in a comment, it could also be the size of the receive antenna was necessary to get the sensitivity needed.

Answer 3

Since the deep space network can perform ranging on spacecraft much farther away (tens of thousands of times farther than the moon) by itself, why was it necessary to use a non-colocated, non-DSN dish to receive signals in this case?

The ranging you're referring to is cooperative radio ranging: The DSN sends a signal to the spacecraft, the spacecraft receives it, and sends it back at maximum gain after a predetermined time. I think signal strength reduces with 2r² in this case.

Radar ranging, in contrast relies on an echo of the transmitted signal, which is much weaker. Signal strength reduces with r⁴.

Answer 4

This started out as a comment on one of the answers already provided, but it grew much too big to stay that way.

There is good reason to collect and process bistatic ranging data even when there is no problem collecting monostatic data. The more of it you take --- in particular, the more bistatic observers you use, and the greater the differences in their locations --- the greater the geometric diversity in your measurement set, and the faster and more accurately good positioning algorithms can find the solution.

One drawback to monostatic radar is that while range is determined well, angular position is not. The uncertainty region is usually described as a "plate" or "pancake", because it's a thin section of spherical shell that may be only a few meters thick but several to many kilometers across (similar to the aspect ratio of a piece of paper). This is particularly bad at large ranges, since the measurement uncertainly in the two directions perpendicular to range are angle errors, which grow linearly with distance when converted to position errors.

Processing bistatic ranging data leads to differently-shaped uncertainty regions; they are still plate-like, but now they are ellipsoidal in section. The farther apart the two radars are, the less spherical the ellipsoid becomes. Having additional bistatic sites gives ellipsoids with different axis directions, which is particularly good when those axes are close to orthogonal.

Why this actually makes anything better depends on the angles at which the uncertainty regions cross. This is tricky to visualize, even with pictures (see link at bottom), because it's not clear at first why the pictures have anything to do with the math. The basic idea is that any measurement (of range, speed, angle, or anything else) gives us an estimate of a volume in which the target most likely lies (to within some nominal confidence, like 50% or 95%). To estimate where the target actually is, make a bunch of different measurements, convert those to uncertainty volumes (the 3D version of a "confidence interval"), intersect them with each other, and the target is most likely near the center of the volume all of those input volumes have in common.

The problem with monostatic ranging, whether with radar reflections or with transponded timing signals, is that the surfaces given by repeated measurement don't change enough. Three pancakes from three different monostatic locations with mutually orthogonal lines of sight intersect in a cube, each side of which is only as thick as the range uncertainty. The angle measurement uncertainty has disappeared, because the constraint that the target be consistent with all three reports led the algorithm to throw away the low quality data, since it had better estimates to use from the other measurements. Three pancakes from the same site at slightly different times, however, are very nearly three copies of the same pancake, so the volume in which they intersect is nearly just the one pancake you started with. Subsequent observations were redundant with each other, because they were made along the same line of sight; the bigger the difference in line of sight direction, the better use that can be made of each additional observation.

The same thing happens when trying to estimate the orbit of a geosynchronous satellite using the measured delay on the time-tagged telemetry signal: you get a good estimate only along the direction you are looking, which usually is almost all radial, and you have very poor sensitivity to errors in the in-track and cross-track directions. Ranging between two widely separated ground stations, however, makes it much easier to resolve errors in the directions other than range. They don't change the range resolution, but they do mean you get to use the range resolution in more than one direction, and don't have to stick with the much lower quality angular resolution.

Everything that I've said so far is true with only minor modification for optical measurement: there, you are very sure of the angular coordinates but have almost no idea what the range might be, so your uncertainty volumes are thin but very long (usually described as pencils or soda straws). You have the same problem of intersecting three straws nearly sharing an axis gives you almost no more information than each straw alone, but also the same advantage that three straws with mutually orthogonal axes give a tiny nearly-cubic volume which has your good resolution in all directions, not just some. If you have both an optical and a radar measurement from the same site, the intersection of a pancake with a straw that shares its axis is a tiny volume, so you have achieved geometric diversity by means of measurement type diversity.

When applied to navigation systems, this topic is often called geometric dilution of precision (GDOP). It is always important to bear in mind that the geometry in question is not a property only of the locations of the receiving stations with respect to each other --- it is the geometry of the ways curved surfaces of uncertain measurement estimation intersect with each other, which depends also on the type of measurements being made, the location of the target, and sometimes the velocities of all the objects involved. A better source of images for this than the Wikipedia link above is animated Matlab documentation (please let me know if this link is subscriber only)

I mentioned velocity here because both measurement plots shown in the question have Doppler shift on the horizontal axis. When processing radar returns from a fast-moving object, you can't avoid noticing that you have to apply a significant frequency shift in order to receive the return at all. As long as the moving object is moving quickly with respect to the Moon (not "selenosynchronous"), all the return from the Moon falls in Doppler bins you just throw away, so it is easy to separate even for monostatic processing. What gets much more interesting is the shape of the volume that corresponds to each of the Doppler measurements. They are much more complicated, and don't have standard names, because the equations were too hard for classical geometers to address. Ellipsoids, hyperboloids, and related things are the solutions of second-degree equations, but frequency difference processing has to solve for the intersections among solutions to eighth-degree equations, giving extra bumps and wiggles and sometimes spiky bits. No matter the shape, repeated monostatic measurements at closely spaced times will give only a small change each time, so that technique is tricky and you may have to wait a long time to get enough change in the measurement volume. If, on the other hand, you process both range (distance, via travel time) and range-rate (velocity, via Doppler shift) simultaneously, the surfaces are so different that you often can do it monostatically at a single time, just as with the case of simultaneous radar range and optical angles.

Now, since the radar emitter in this example is a Deep Space Network antenna, I should say a few words about Voyager as well as Chandrayaan. As described in this question about "three-way Doppler shift measurement", Voyager is so far away that even at light speed, the radio signal takes so long to get there and come back that the site has moved significantly. This causes us some trouble even in defining "monostatic", because if your one physical site sees Voyager in two measurably different parts of the sky on send than on receive, because you really have moved, the collection geometry really is bistatic, so you are forced to process the return that way, and get the benefits even from your one lone site. However, again since "Voyager is so danged far away", even the full width of the Earth subtends a very small angle from the satellite's point of view, so the amount of geometric diversity that applies to the computation is greatly reduced, and you might need one station on Earth and the other on the Moon to get the same effect.