Given that the cubic body is of homogeneous mass (say Iron) and we want to start with the best possible orbit and input nothing afterwards, could something orbit this cubic body stably?
-
4$\begingroup$ I downloaded these last year and planned on plotting some of the orbits. The first paper deals with a non-rotating cube, and finds there exist stable orbits both in the x, y, and z=0 planes, as well as other out-of-plane orbits. The second paper deals with orbits around a rotating cube - the rotating lumpy gravity field makes for an exciting ride. I believe they find stable orbits, some of which "hover" or orbit around a certain region above the rotating cube, like a geosynchronous orbit would. $\endgroup$– uhohCommented Apr 1, 2017 at 18:23
-
1$\begingroup$ While both papers have many fascinating looking plots, most are either plots of energy, invariant manifolds, or Poincaré maps. I don't have time right now to post a quality answer, but I'm sure there are other people who can. Excellent question!! $\endgroup$– uhohCommented Apr 1, 2017 at 18:28
-
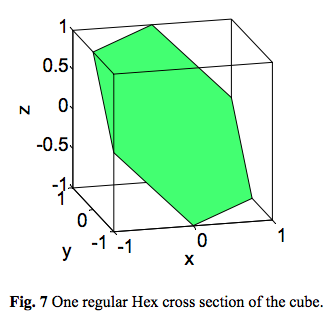
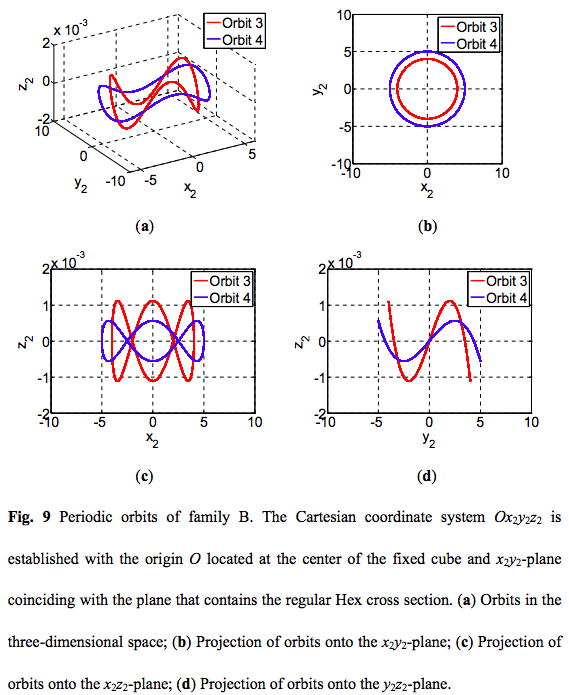
1$\begingroup$ Figures, 7 through 9 of the first paper do show example plots of three families of orbits (A, B, C). Note that the $x_2, y_2, z_2$ axes in Figure 8 are fixed to the non-symmetric plane, not the original cube axes. $\endgroup$– uhohCommented Apr 2, 2017 at 4:16
-
1$\begingroup$ Thank you very much! Now down to the work of understanding it. :) $\endgroup$– Keith DGuyCommented Apr 2, 2017 at 21:09
-
1$\begingroup$ Yep, that's how I feel too! It may take a little time but we'll get there. There may be write-ups of this research in popular science or math news sites or journals, I'll take a look. $\endgroup$– uhohCommented Apr 3, 2017 at 1:56
1 Answer
Before the question is closed I'll post my original comment as an answer.
The problem of simply calculating the gravitational field of a cube is already interesting in part because more complex objects such as asteroids can be built-up from many smaller cubes, for example using the Marching Cubes technique.
See also:
The idea of using the "Polyhedra Method" to approximate an arbitrarily shaped object as a series of polyhedra seems to go back to the book The Theory of the Potential, MacMillan W. D. 1930, New York: McGraw-Hill. Republished by Dover, New York, 1958. This method was used in the very interesting paper by JPL’s Robert A. Werner and Daniel J. Scheeres Exterior gravitation of a polyhedron derived and compared with harmonic and mascon gravitation representations of asteroid 4769 Castalia Celest. Mech. Dyn. Astron. 65(3), 313–344 (1997) where they compare to using a spherical harmonic technique (e.g. $J_2$...) as well as trying to define the potential in terms of a central or spherical mass plus a series of "mascons" or mass concentrations.
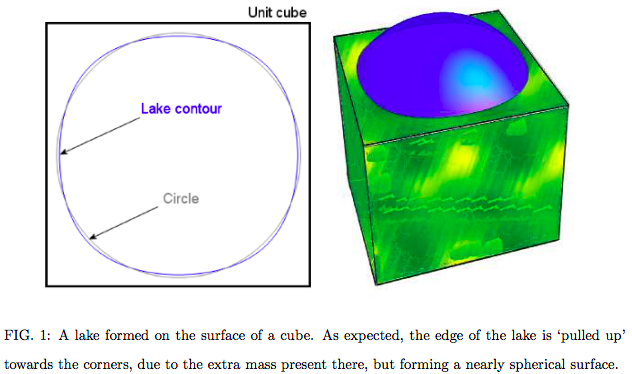
The first discussion to the problem that also considers the orbits about cubes that I've found was by James M. Chappell, Mark J. Chappell, Azhar Iqbal and Derek Abbott in their 2012 paper The gravitational field of a cube (also here). Here they also explore what a "lake" would look like as well as some of the possible orbits. However they were not able to identify stable, closed orbits.
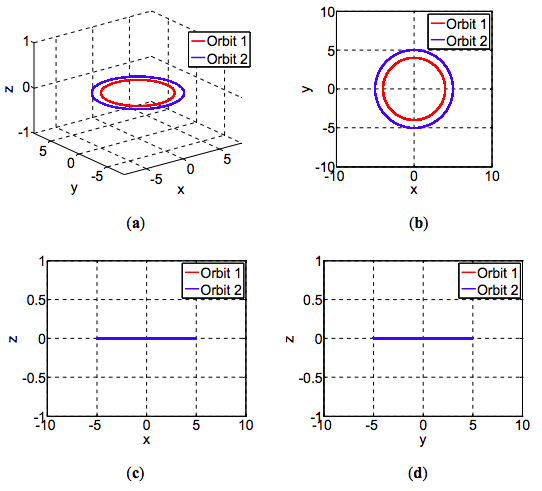
The problem was studied further in the 2011 paper by Xiaodong Liu, Hexi Baoyin, Xingrui Ma Periodic orbits in the gravity field of a fixed homogeneous cube. They identified orbits which they state are closed and stable around a fixed, non rotating cube, both in the symmetry plane (one the cube's "equators") as well as in the plane of the inscribed hexagon with the cube.
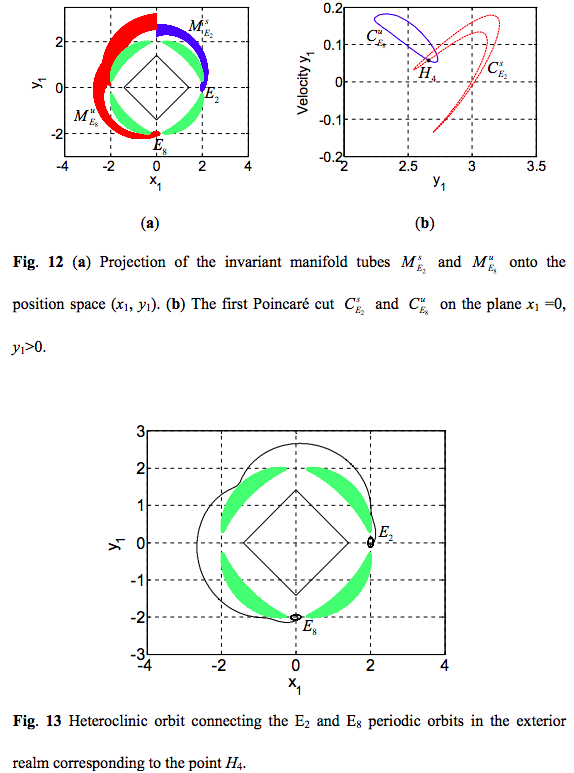
In the companion paper by the same authors around the same time Equilibria, periodic orbits around equilibria, and heteroclinic connections in the gravity field of a rotating homogeneous cube they analyze orbits about a rotating cube. They find stable orbits, some of which "hover" or orbit around a certain region above the rotating cube, like a geosynchronous orbit would.
It is always difficult to draw orbits in rotating frames in 2D. I'll include one "sample" figure which gives a flavor of their results, but one has to read the paper in depth to appreciate what the orbits will actually be like.
-
1$\begingroup$ Very exotic orbits. Hard to believe that orbits of such a complexity could be stable. If there is a little influence of drag or radiation pressure, will these orbits be still stable? $\endgroup$– UweCommented Dec 9, 2017 at 11:52
-
1$\begingroup$ @Uwe In these papers, the term "stable" refers to mathematical stability, which does not necessarily address what happens when a new, external perturbing influence is added to the problem. The authors do claim stability; "The stability of these periodic orbits can be determined by the eigenvalues of the monodromy matrix. The system is stable only if all eigenvalues of the monodromy matrix are located on the unit circle." Of course you know that a lossy influence such as drag will bring almost any orbit down in time. $\endgroup$– uhohCommented Dec 9, 2017 at 12:01